Calculate the mean deviation about median of the following data: 34, 66, 30, 38, 44, 50, 40, 60, 42, 51
To calculate the mean deviation about the median for the given data set 34,66,30,38,44,50,40,60,42,51, we will follow these steps:
Step 1: Arrange the Data in Ascending Order
First, we need to arrange the data in ascending order.
Data in ascending order:
30, 34, 38, 40, 42, 44, 50, 51, 60, 66
Step 2: Find the Median
To find the median, we need to identify the middle value(s) of the ordered data. Since there are 10 numbers (even count), the median will be the average of the 5th and 6th terms.
5th term: 42
6th term: 44
Median calculation:
Median=42+442=862=43
Step 3: Calculate the Mean Deviation
The mean deviation is calculated using the formula:
Mean Deviation=∑|xi−Median|n
where xi are the data points, and n is the number of data points.
Calculating |xi−Median| for each data point:
- For 30: |30−43|=13
- For 34: |34−43|=9
- For 38: |38−43|=5
- For 40: |40−43|=3
- For 42: |42−43|=1
- For 44: |44−43|=1
- For 50: |50−43|=7
- For 51: |51−43|=8
- For 60: |60−43|=17
- For 66: |66−43|=23
Step 4: Sum of Absolute Deviations
Now, we will sum all the absolute deviations calculated above:
Sum=13+9+5+3+1+1+7+8+17+23=85
Step 5: Divide by the Number of Data Points
Finally, we divide the sum of absolute deviations by the number of data points (which is 10):
Mean Deviation=8510=8.5
Final Answer
The mean deviation about the median is 8.5.
---
First, we need to arrange the data in ascending order.
30, 34, 38, 40, 42, 44, 50, 51, 60, 66
To find the median, we need to identify the middle value(s) of the ordered data. Since there are 10 numbers (even count), the median will be the average of the 5th and 6th terms.
6th term: 44
Median=42+442=862=43
The mean deviation is calculated using the formula:
Mean Deviation=∑|xi−Median|n
where xi are the data points, and n is the number of data points.
- For 34: |34−43|=9
- For 38: |38−43|=5
- For 40: |40−43|=3
- For 42: |42−43|=1
- For 44: |44−43|=1
- For 50: |50−43|=7
- For 51: |51−43|=8
- For 60: |60−43|=17
- For 66: |66−43|=23
Now, we will sum all the absolute deviations calculated above:
Sum=13+9+5+3+1+1+7+8+17+23=85
Finally, we divide the sum of absolute deviations by the number of data points (which is 10):
The mean deviation about the median is 8.5.
Topper's Solved these Questions
Similar Questions
Find the mean deviation about median for the following data:

View SolutionFind the mean deviation about mean for the following data:
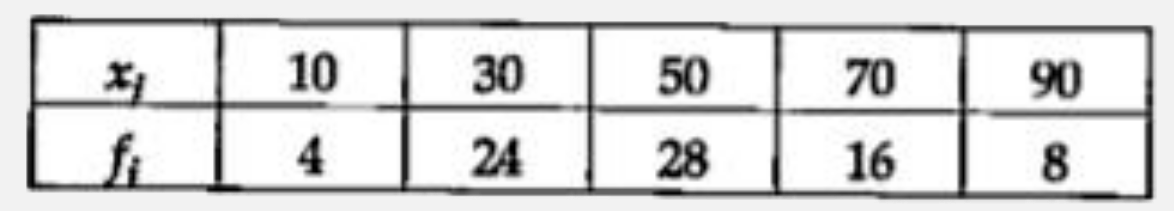
View SolutionFind the mean deviation from the median for the following data:
34, 66, 30, 38, 44, 50, 40, 60, 42, 51.
View SolutionCalculate the mean deviation about median from the following data: 340, 150, 210, 240, 300, 310, 320.
View SolutionCalculate the mean deviation about median from the following data: 340, 150, 210, 240, 300, 310, 320.
View SolutionFind the mean deviation about the mean for the following data :

View SolutionFind the mean deviation about the mean for the following data :

View SolutionFind the mean deviation about the mean for the following data :

View SolutionFind the mean deviation about the median for the following data:
11, 3, 8, 7, 5, 14, 10, 2, 9
View SolutionFind the mean deviation using median for the following datas:

View Solution
