Calculate the mean deviation of the following income groups of five and seven members from their medians: 1st income group (in Rs.): 4000 4200 4400 4600 4800 2nd income group (in Rs.): 3800 4000 4200 4400 4600 4800 5800
To calculate the mean deviation of the given income groups from their medians, we will follow these steps:
Step 1: Identify the Income Groups
The income groups are:
1. First income group: 4000, 4200, 4400, 4600, 4800
2. Second income group: 3800, 4000, 4200, 4400, 4600, 4800, 5800
Step 2: Calculate the Median for the First Income Group
The first income group has 5 members. The median is calculated as follows:
- Median = (n+1)2th observation
- Here, n=5, so the median is the (5+1)2=3rd observation.
- The 3rd observation is 4400.
Median of the first income group = 4400
Step 3: Calculate the Absolute Deviations from the Median for the First Income Group
Now, we will calculate the absolute deviations of each income from the median (4400):
- For 4000: |4000−4400|=400
- For 4200: |4200−4400|=200
- For 4400: |4400−4400|=0
- For 4600: |4600−4400|=200
- For 4800: |4800−4400|=400
Step 4: Sum the Absolute Deviations for the First Income Group
Now, we sum the absolute deviations:
- Total = 400+200+0+200+400=1200
Step 5: Calculate the Mean Deviation for the First Income Group
Mean deviation is calculated as:
- Mean Deviation = Total Absolute Deviationsn
- Here, n=5, so:
- Mean Deviation = 12005=240
Mean Deviation of the first income group = 240
Step 6: Calculate the Median for the Second Income Group
The second income group has 7 members. The median is calculated as follows:
- Median = (n+1)2th observation
- Here, n=7, so the median is the (7+1)2=4th observation.
- The 4th observation is 4400.
Median of the second income group = 4400
Step 7: Calculate the Absolute Deviations from the Median for the Second Income Group
Now, we will calculate the absolute deviations of each income from the median (4400):
- For 3800: |3800−4400|=600
- For 4000: |4000−4400|=400
- For 4200: |4200−4400|=200
- For 4400: |4400−4400|=0
- For 4600: |4600−4400|=200
- For 4800: |4800−4400|=400
- For 5800: |5800−4400|=1400
Step 8: Sum the Absolute Deviations for the Second Income Group
Now, we sum the absolute deviations:
- Total = 600+400+200+0+200+400+1400=2800
Step 9: Calculate the Mean Deviation for the Second Income Group
Mean deviation is calculated as:
- Mean Deviation = Total Absolute Deviationsn
- Here, n=7, so:
- Mean Deviation = 28007=400
Mean Deviation of the second income group = 400
Final Results
- Mean Deviation of the first income group = 240
- Mean Deviation of the second income group = 400
---
The income groups are:
1. First income group: 4000, 4200, 4400, 4600, 4800
2. Second income group: 3800, 4000, 4200, 4400, 4600, 4800, 5800
The first income group has 5 members. The median is calculated as follows:
- Median = (n+1)2th observation
- Here, n=5, so the median is the (5+1)2=3rd observation.
- The 3rd observation is 4400.
Now, we will calculate the absolute deviations of each income from the median (4400):
- For 4000: |4000−4400|=400
- For 4200: |4200−4400|=200
- For 4400: |4400−4400|=0
- For 4600: |4600−4400|=200
- For 4800: |4800−4400|=400
Now, we sum the absolute deviations:
- Total = 400+200+0+200+400=1200
Mean deviation is calculated as:
- Mean Deviation = Total Absolute Deviationsn
- Here, n=5, so:
- Mean Deviation = 12005=240
The second income group has 7 members. The median is calculated as follows:
- Median = (n+1)2th observation
- Here, n=7, so the median is the (7+1)2=4th observation.
- The 4th observation is 4400.
Now, we will calculate the absolute deviations of each income from the median (4400):
- For 3800: |3800−4400|=600
- For 4000: |4000−4400|=400
- For 4200: |4200−4400|=200
- For 4400: |4400−4400|=0
- For 4600: |4600−4400|=200
- For 4800: |4800−4400|=400
- For 5800: |5800−4400|=1400
Now, we sum the absolute deviations:
- Total = 600+400+200+0+200+400+1400=2800
Mean deviation is calculated as:
- Mean Deviation = Total Absolute Deviationsn
- Here, n=7, so:
- Mean Deviation = 28007=400
- Mean Deviation of the first income group = 240
- Mean Deviation of the second income group = 400
Topper's Solved these Questions
Similar Questions
Calculate coefficient of variation from the following data: Income (in Rs.) 1000-1700 1700-2400 2400-3100 3100-3800 3800-4500 4500-5200 No. o families 12 18 20 25 35 10
View SolutionThe following table gives the distribution of income of 100 families in a village. Calculate the standard deviation: Income Rs., 0-1000, 1000-2000, 2000-3000, 3000-4000, 4000-5000, 5000-6000 No. of Families, 18, 26, 30, 12, 10, 4
View SolutionThe following table gives the distribution of income of 100 families in a village. Calculate the standard deviation: Income Rs. 0-1000 1000-2000 2000-3000 3000-4000 4000-5000 5000-6000 No. of Families 18 26 30 12 10 4
View SolutionIntramolecular aldol condensation :
The aldol condensation also offer a convenient way to synthesize molecules with five and six membered ring.This can be done by an intramolecular aldol condensation using a dialdehyde, a keto aldehyde or a diketone as the substrate.The major product is formed by the attack of the enolate from the ketone side of the molecule that adds to the aldehyde group.The reason the aldehyde group undergoes addition preferentially may arise from the greater reactivity of aldehyde towards nucleophilic addition.In reaction of this type five membered rings from far more readily than seven membered rings and six membered rings are more favourable than four or eight membered rings when possible.
The true statement about the major product of CH3−O∣∣C−CH2−CH2−CH2−CH2−O∣∣C−H in reaction with aq.NaOH followed by heating is.
View SolutionIntramolecular aldol condensation :
The aldol condensation also offer a convenient way to synthesize molecules with five and six membered ring.This can be done by an intramolecular aldol condensation using a dialdehyde, a keto aldehyde or a diketone as the substrate.The major product is formed by the attack of the enolate from the ketone side of the molecule that adds to the aldehyde group.The reason the aldehyde group undergoes addition preferentially may arise from the greater reactivity of aldehyde towards nucleophilic addition.In reaction of this type five membered rings from far more readily than seven membered rings and six membered rings are more favourable than four or eight membered rings when possible.
1-Etylcyclopent-1-ene on reductive ozonolysis followed by aq. NaOH/Δ gives
View SolutionA company selected 4000 households at random and surveyed them to find out a relationship between income level and the number of televisions sets in a home. The information, so obtained is listed in the following table.
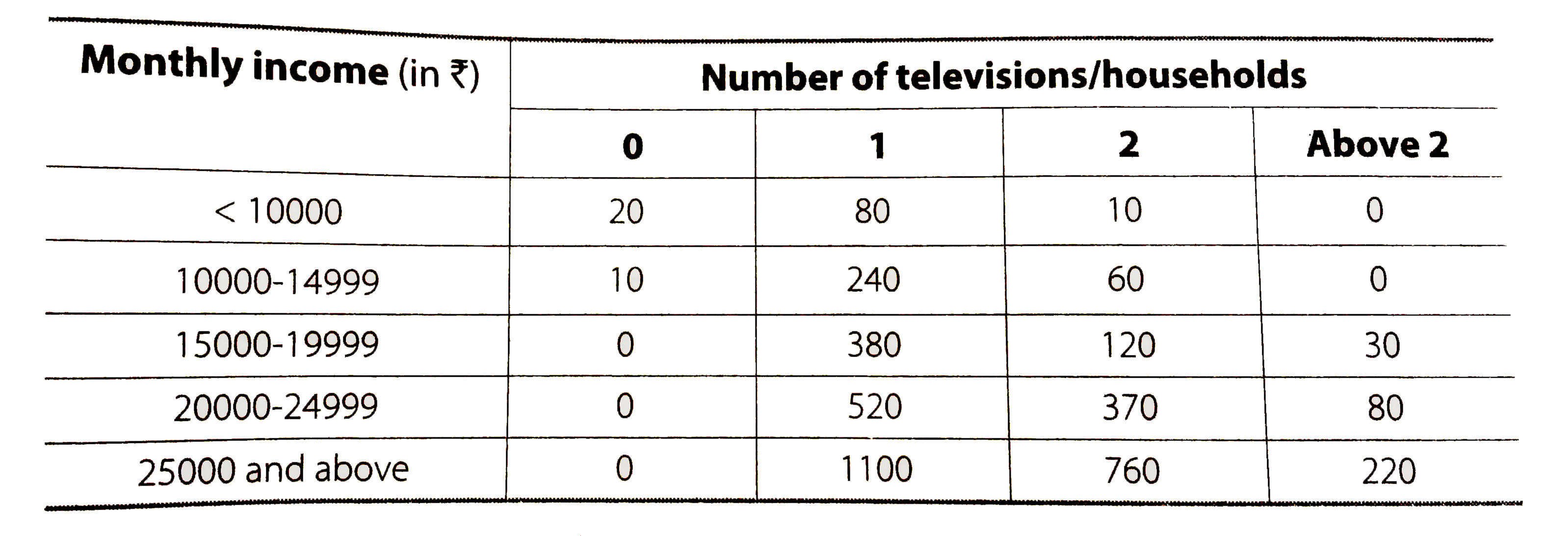
Find the probability
i) of a household earning Rs 10000- Rs 14999 per year and having exactly one television.
ii) of a household earning Rs 25000 and more per year owning 2 televisions.
iii) of a household not having any television.
View SolutionCompute the first quartile and third deciles from the following data :
Weekly Income (in Rs.)585960616263646566No. of workers23615105431
View Solution
