Question

Given $a\Delta ABC$ in which $\angle B={90}^{\circ }\text{and}AB=\sqrt{3}BC$. Prove that $\angle C={60}^{\circ }$

Explanation

Let D be the midpoint of the hypotenuse AC.
Join BD.
We have
`AC^(2)=AB^(2)+BC^(2)` [ by Pythagoras theorem]
`rArr AC^(2)=(sqrt(3)BC)^(2)+BC^(2) [ :. AB=sqrt(3)BC "given" ]`
`rArr AC^(2)=4BC^(2) rArr AC=2BC`
`rArr 2CD=2BC [ :. D` is the midpoint of AC]
`rArr CD= BC" ...(i)`

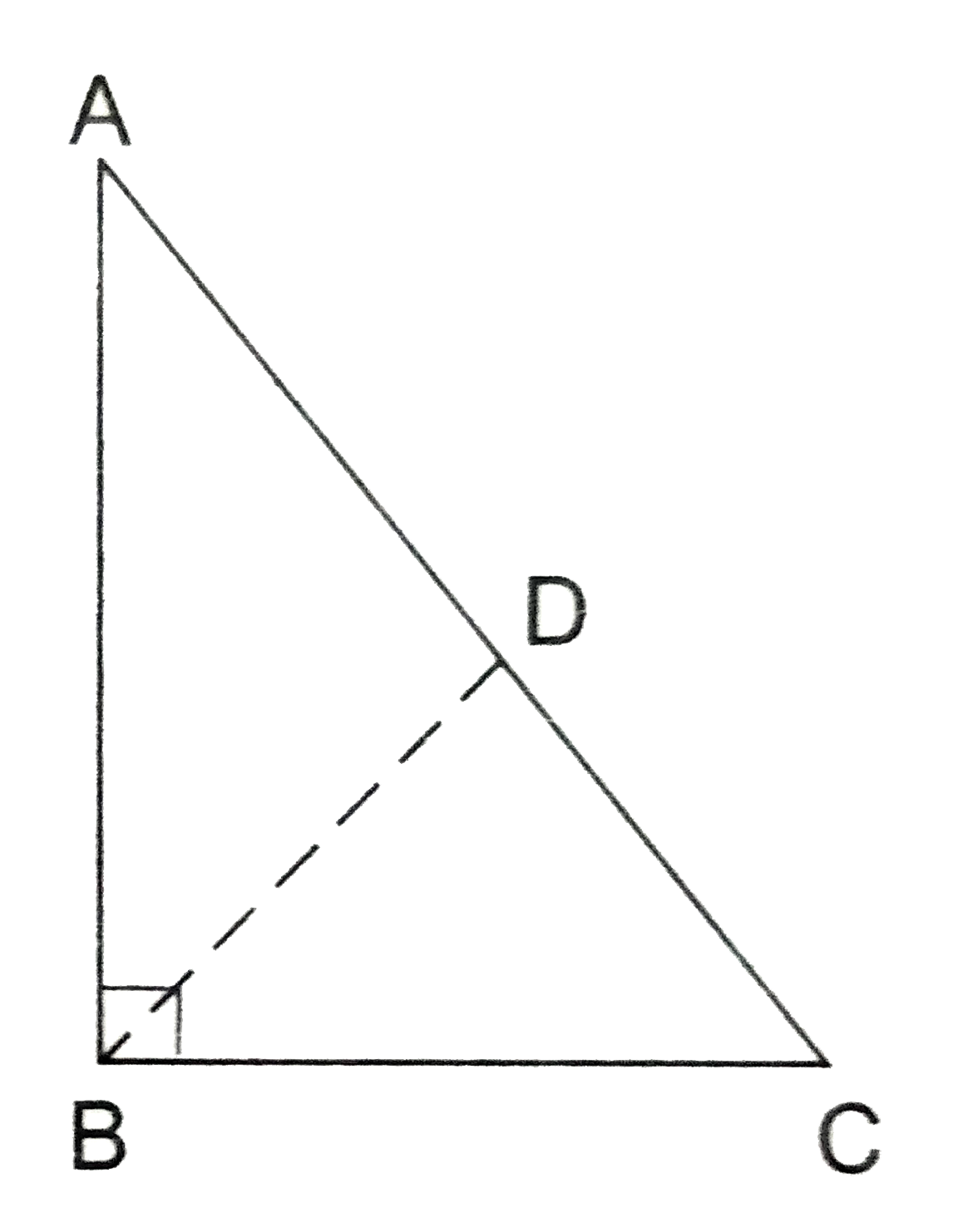
Also, we know tha the midpoint of the hypotenus of a right triangle is equidistant from the vertices
` :. BD=CD" "....(ii)`
From (i) and (ii), we get
`BC=BD=CD`
`:. Delta BCD` is equilateral and hence `angle C=60^(@)`