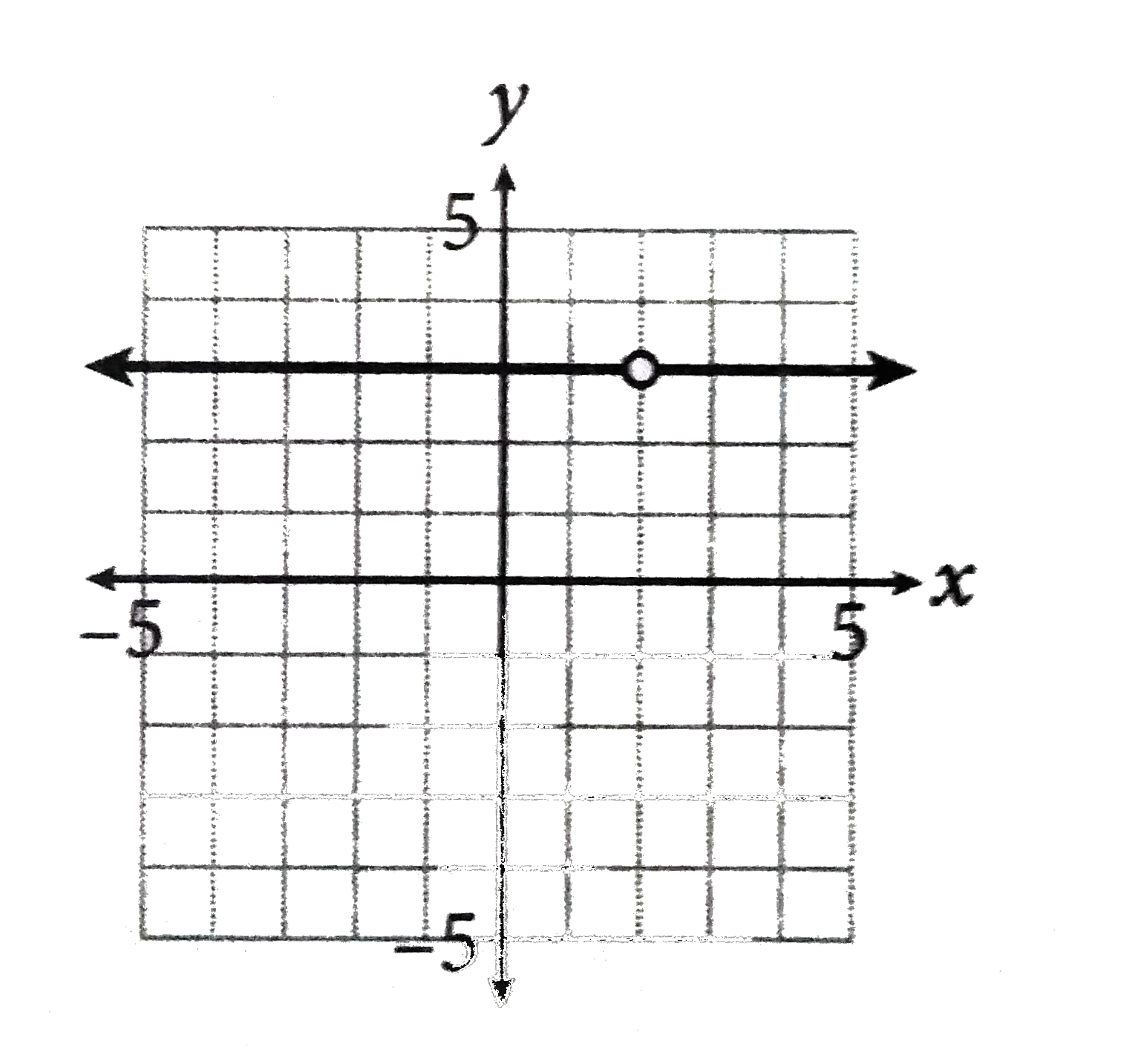
If a function p(x) has four distinct zeros, which of the following could represent the entire graph of p in a standard coordinate plane?

The correct Answer is:D
To solve the problem, we need to determine which graph represents a function p(x) that has four distinct zeros. A zero of a function is a value of x for which p(x)=0, and graphically, these zeros correspond to the points where the graph intersects the x-axis.
1. Understand the Concept of Zeros:
- Zeros of a function are the x-values where the function equals zero. In a graph, these are the points where the graph crosses the x-axis.
2. Identify the Requirement:
- We need to find a graph that intersects the x-axis at four distinct points. This means the graph must have four separate x-intercepts.
3. Analyze the Options:
- We will evaluate each option to see how many times it intersects the x-axis.
4. Evaluate Option A:
- Check how many times the graph intersects the x-axis.
- Result: It intersects the x-axis only two times.
- Conclusion: This option does not have four distinct zeros.
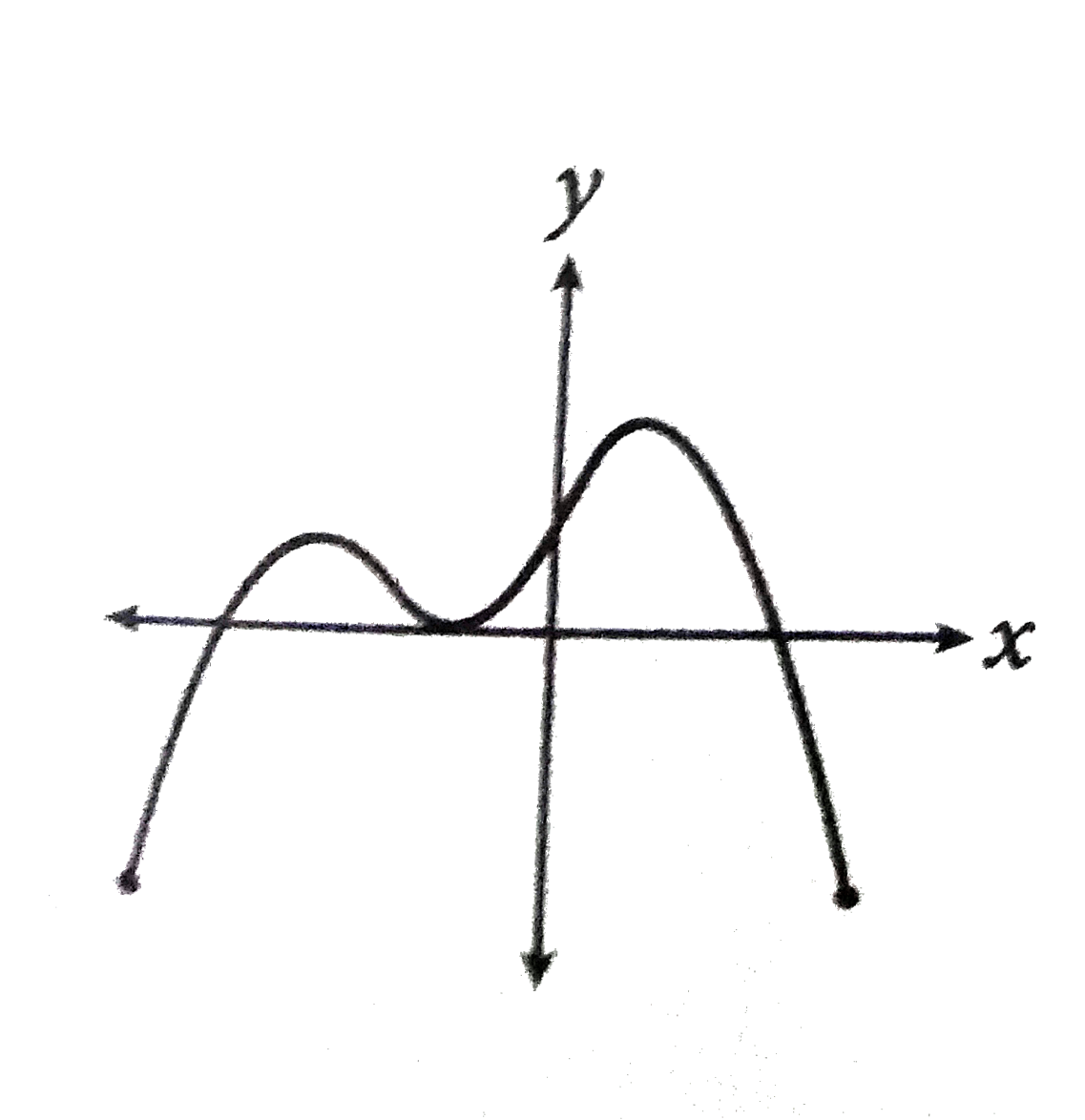
5. Evaluate Option B:
- Check how many times the graph intersects the x-axis.
- Result: It intersects the x-axis three times.
- Conclusion: This option does not have four distinct zeros.
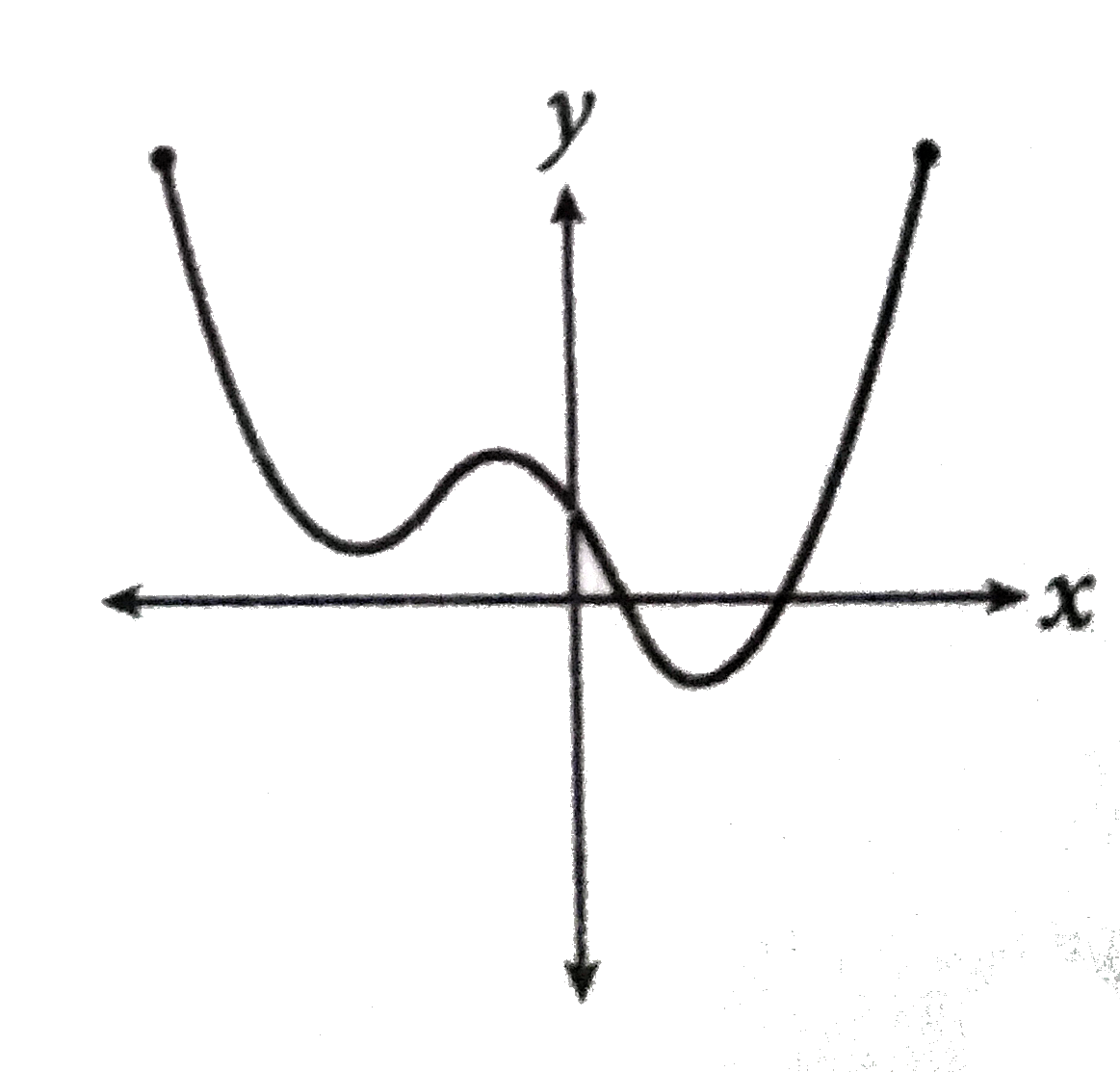
6. Evaluate Option C:
- Check how many times the graph intersects the x-axis.
- Result: It intersects the x-axis two times.
- Conclusion: This option does not have four distinct zeros.
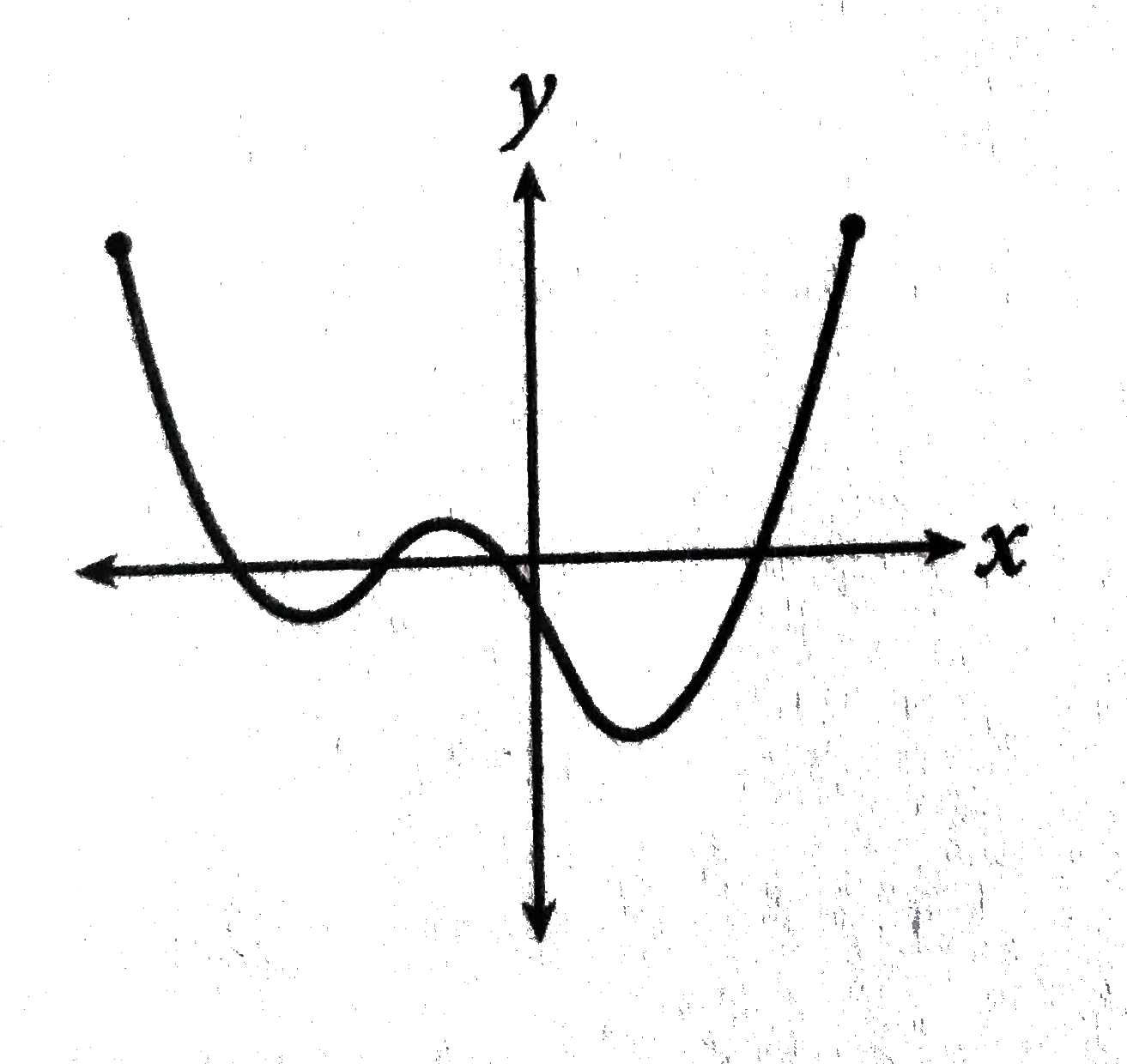
7. Evaluate Option D:
- Check how many times the graph intersects the x-axis.
- Result: It intersects the x-axis four times.
- Conclusion: This option has four distinct zeros.
8. Final Decision:
- Since only Option D intersects the x-axis four times, it is the correct representation of the function p(x) with four distinct zeros.
Conclusion:
The correct answer is Option D.
- Zeros of a function are the x-values where the function equals zero. In a graph, these are the points where the graph crosses the x-axis.
- We need to find a graph that intersects the x-axis at four distinct points. This means the graph must have four separate x-intercepts.
- We will evaluate each option to see how many times it intersects the x-axis.
- Check how many times the graph intersects the x-axis.
- Result: It intersects the x-axis only two times.
- Conclusion: This option does not have four distinct zeros.
- Check how many times the graph intersects the x-axis.
- Result: It intersects the x-axis three times.
- Conclusion: This option does not have four distinct zeros.
- Check how many times the graph intersects the x-axis.
- Result: It intersects the x-axis two times.
- Conclusion: This option does not have four distinct zeros.
- Check how many times the graph intersects the x-axis.
- Result: It intersects the x-axis four times.
- Conclusion: This option has four distinct zeros.
- Since only Option D intersects the x-axis four times, it is the correct representation of the function p(x) with four distinct zeros.
The correct answer is Option D.
Topper's Solved these Questions
Similar Questions
Knowledge Check
If the function f has three distinct zeros, which of the following represents the graph of f in the xy-plane?
A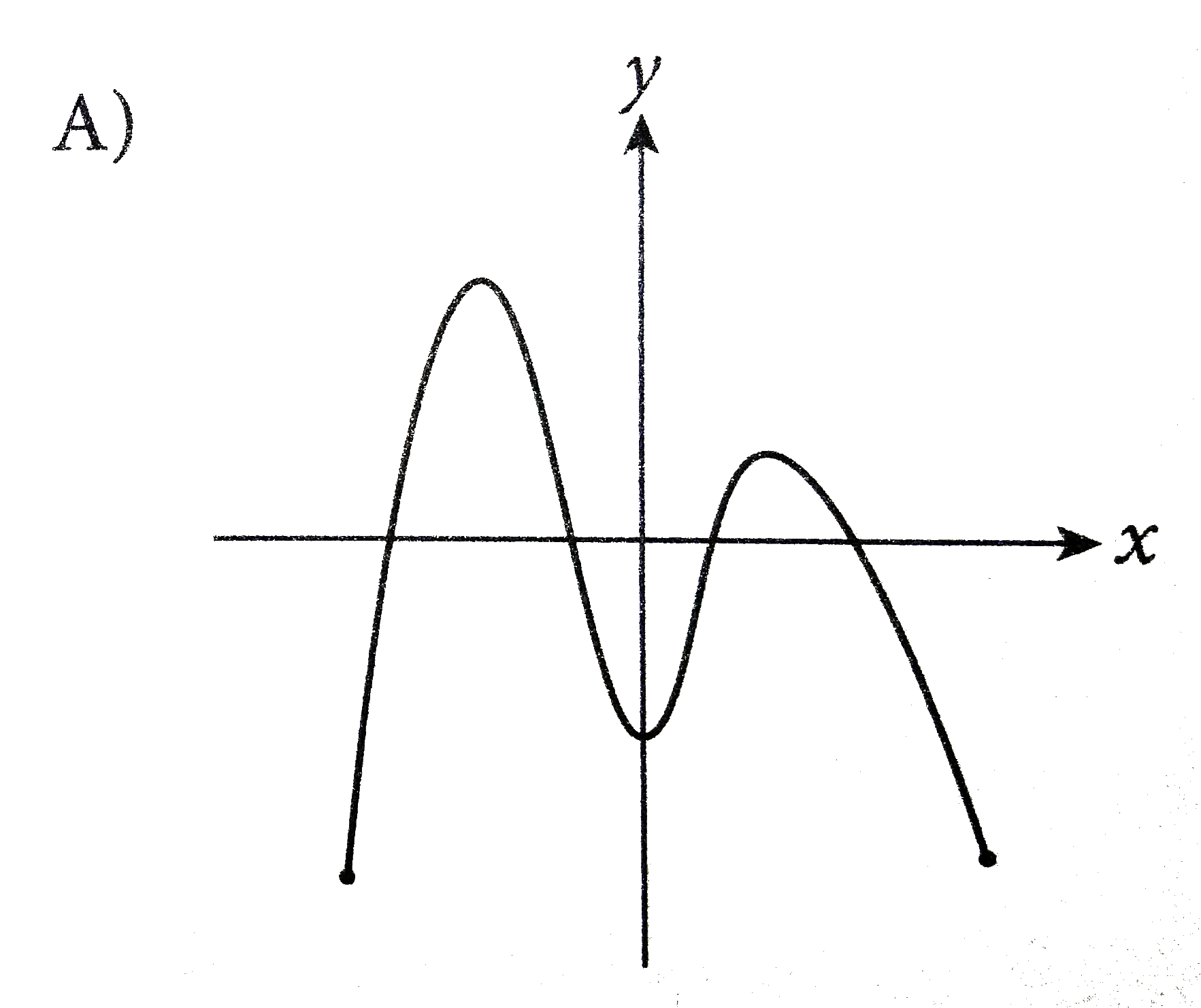 B
B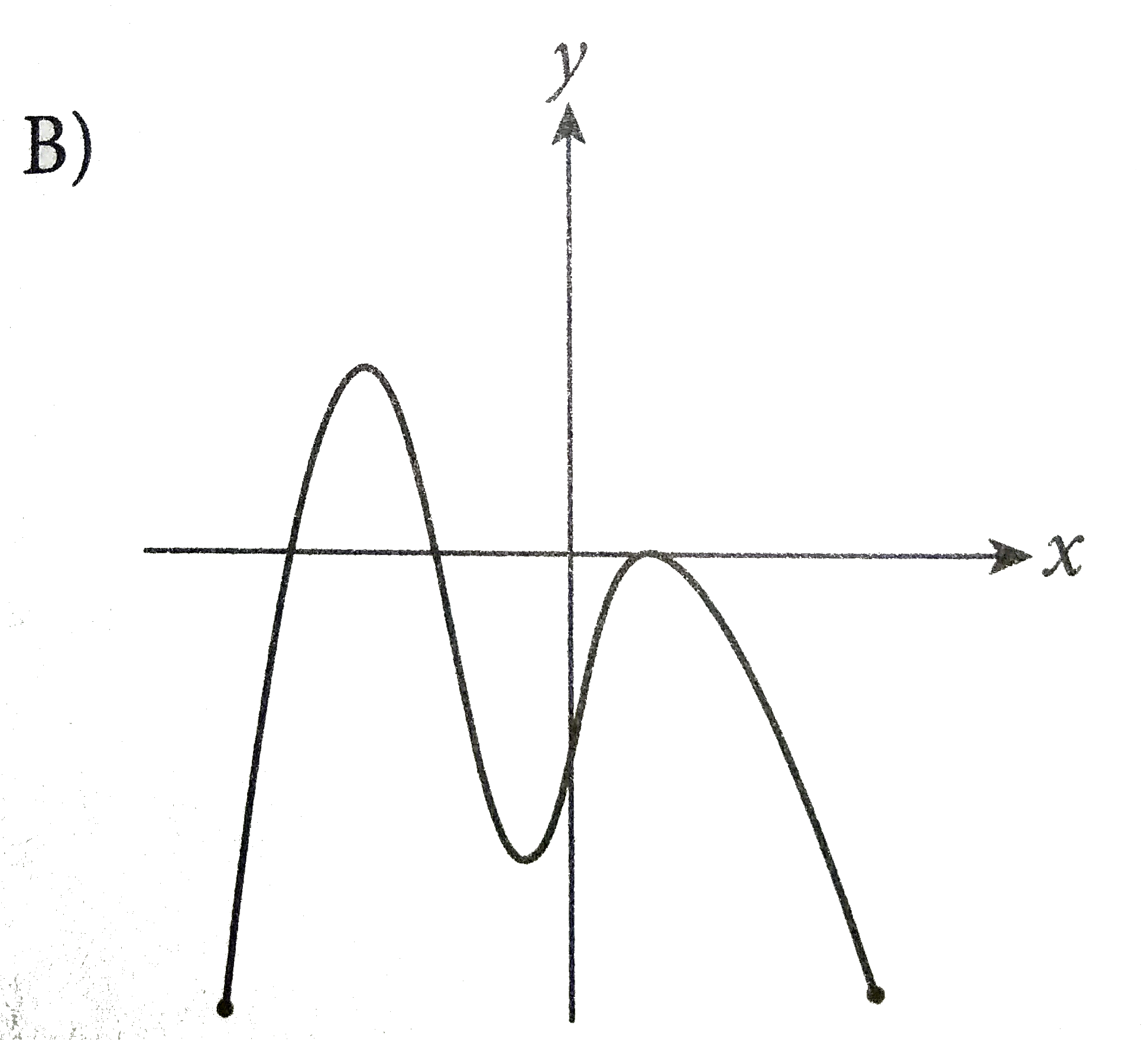 C
C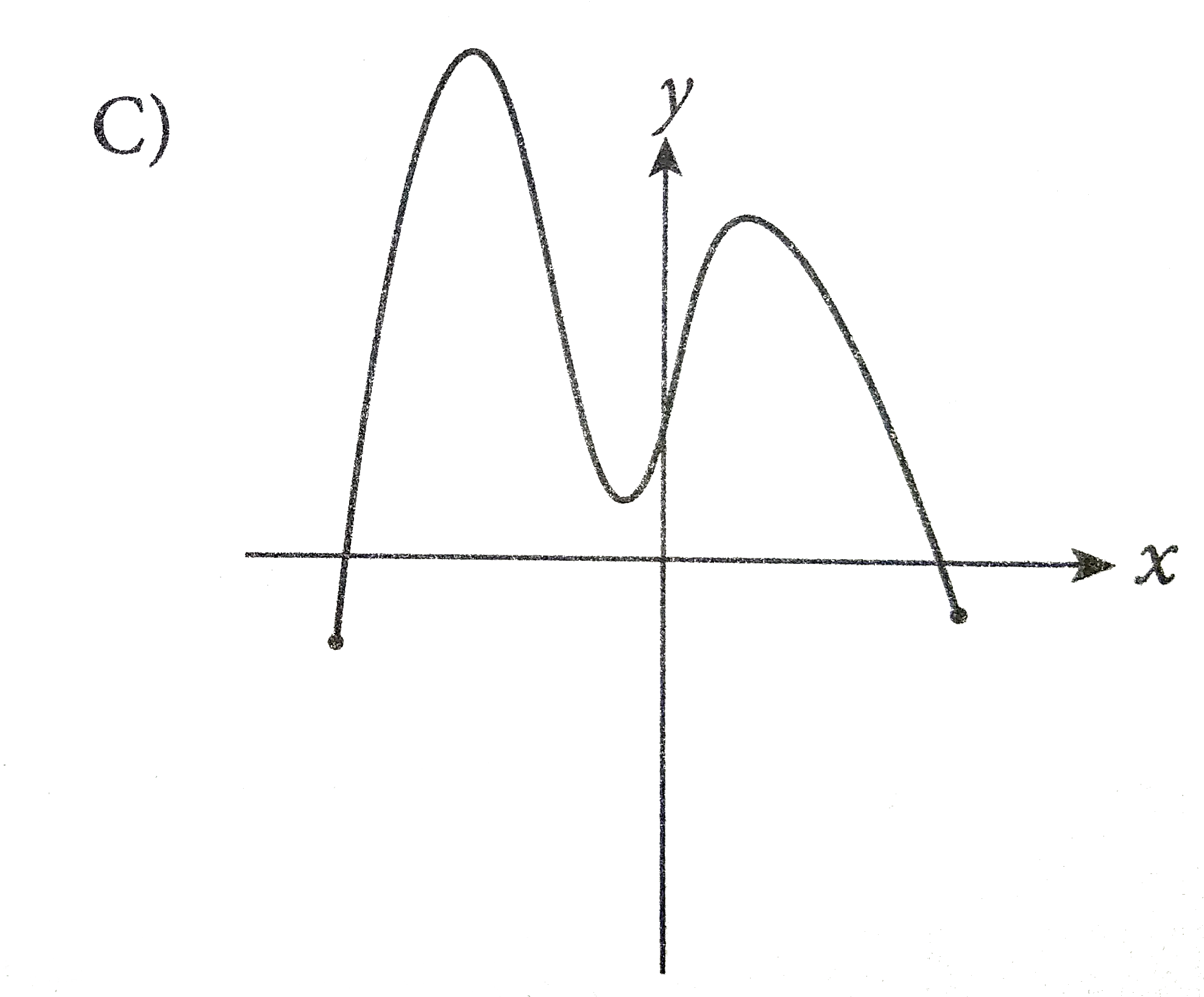 D
D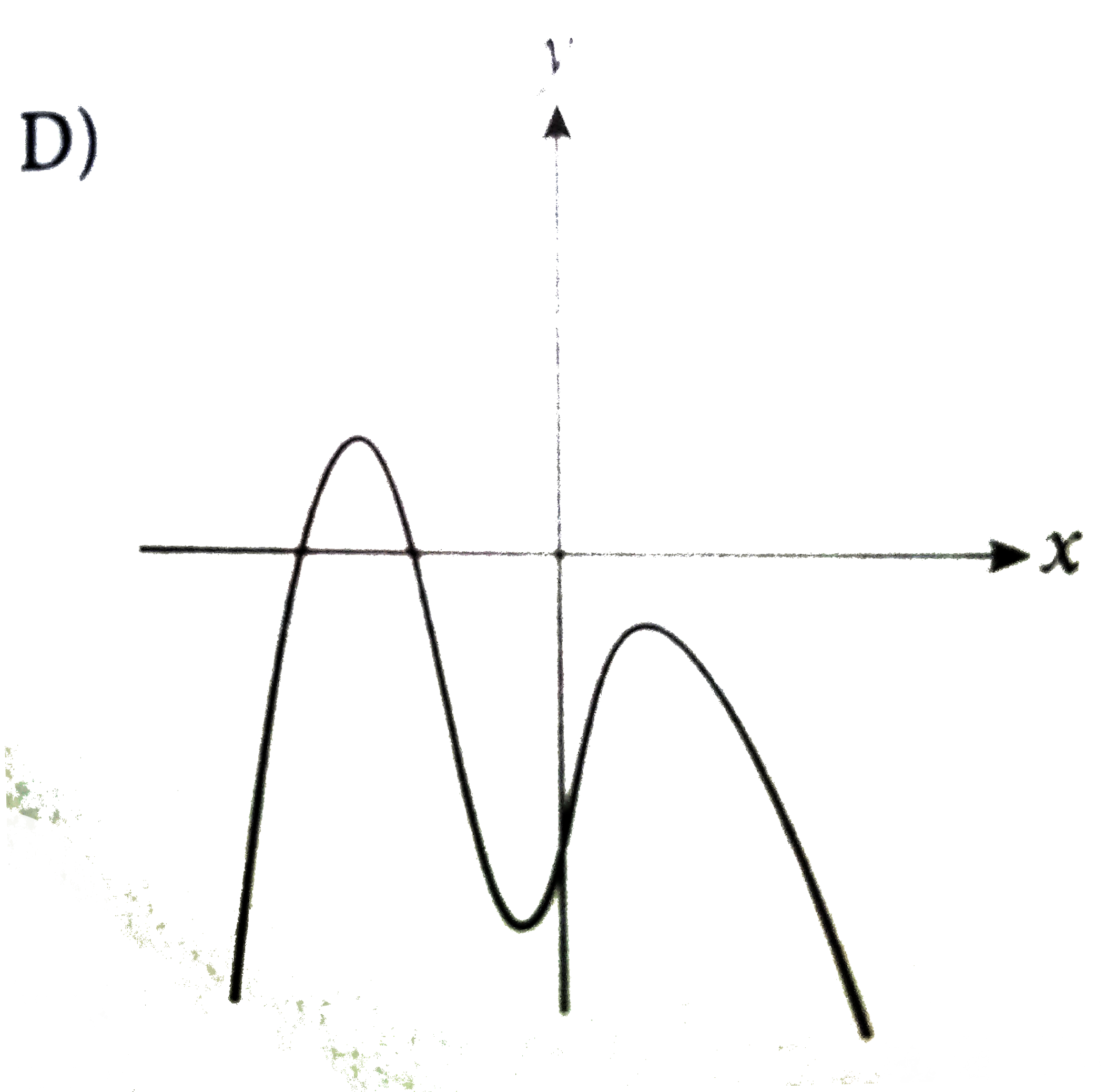
12 If the function f has five distinct zeros, which of the following could represent the complete graph of f in the xy‑plane?
A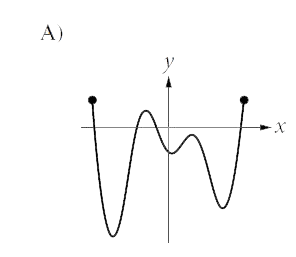 B
B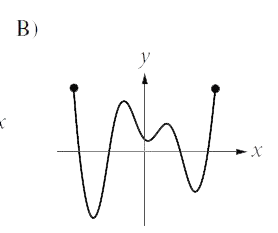 C
C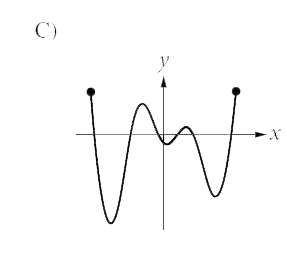 D
D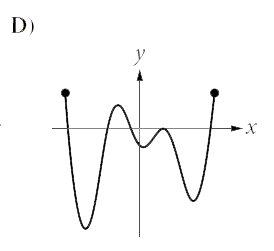
Which of the following is the equation of a circle in the standard (x,y) coordinate plane ?
A(x-3)+(y+6)=100By=x2+25Cx2=y2+25Dx2=36−y2
If function f has exactly two distinct real zeros, which of the following graphs could be the complete graph of f (x) ?
View SolutionWhich of the following P-V diagram represent the graph of isometric process?
View Solution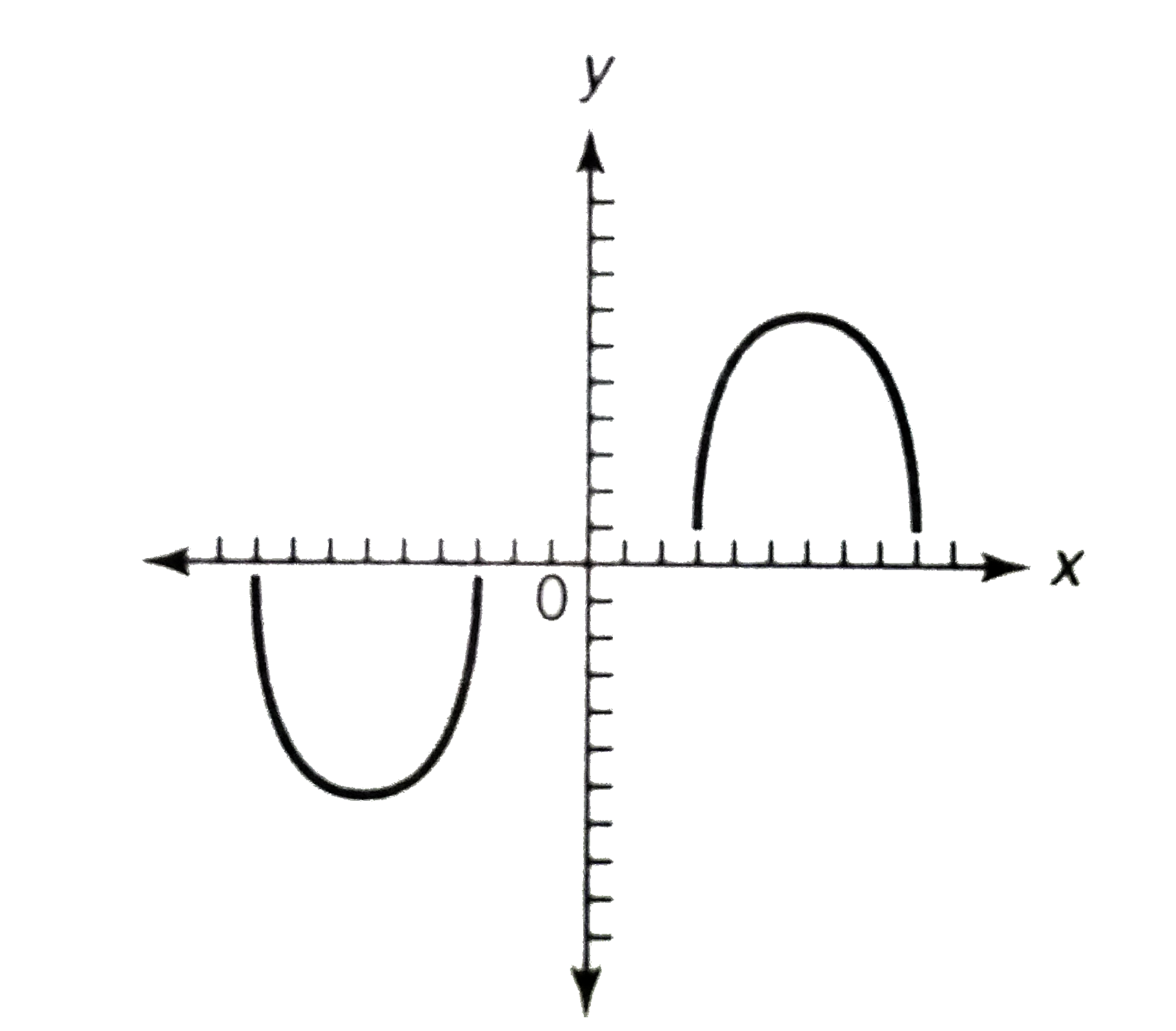
The graph of the function f is shown above. Which of the following could represent the graph of y=|f(x)|?
View Solutionf (x) = (x + 3)(x − k)
T he function f is defined above. If k is a positive integer, which of the following could represent the graph of y = f (x) in the xy-plane?
View Solution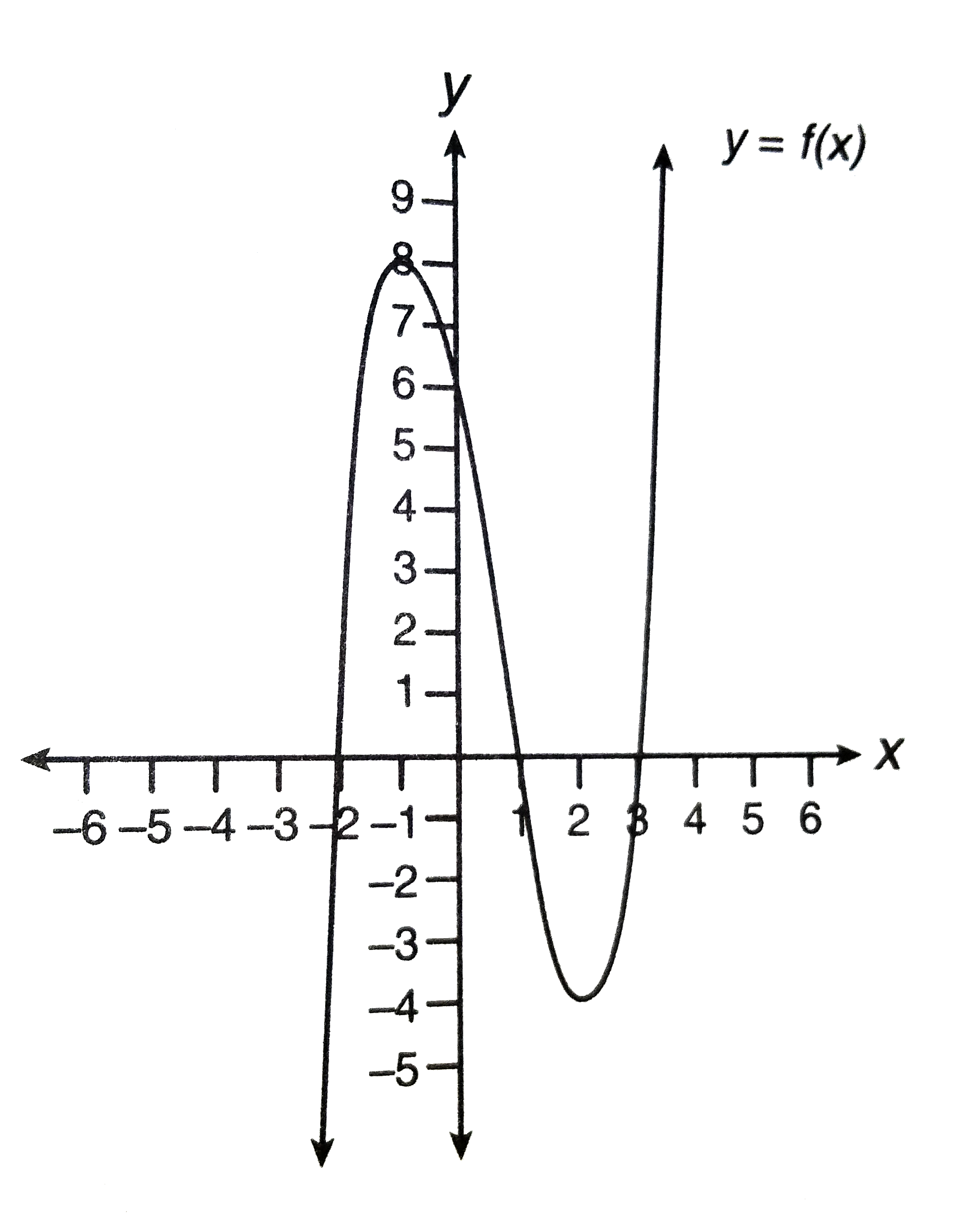
Which function could represents the graph above?
View Solution