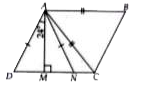
If ABCD is a parallelogram, ΔADNandΔABC are isosceles triangles, then find ∠BAC.
112∘
140∘
48∘
32∘
The correct Answer is:C
Step by step video, text & image solution for If ABCD is a parallelogram, Delta ADN and Delta ABC are isosceles triangles, then find angleBAC. by Maths experts to help you in doubts & scoring excellent marks in Class 8 exams.
Topper's Solved these Questions
- BOOK - SCIENCE OLYMPIAD FOUNDATION CHAPTER - IMO QUESTION PAPER 2018-19 SET- AEXERCISE - Everyday Mathematics10videos
IMO QUESTION PAPER 2018-19 SET- A
- BOOK - SCIENCE OLYMPIAD FOUNDATION CHAPTER - IMO QUESTION PAPER 2018-19 SET- AEXERCISE - Achievers Section5videos
IMO QUESTION PAPER 2018-19 SET- A
- BOOK - SCIENCE OLYMPIAD FOUNDATION CHAPTER - IMO QUESTION PAPER 2018-19 SET -B EXERCISE - ACHIEVERS SECTION5videos
IMO QUESTION PAPER 2018-19 SET -B
- BOOK - SCIENCE OLYMPIAD FOUNDATION CHAPTER - IMO QUESTION PAPER 2019-20 SET AEXERCISE - Achievers Section5videos
IMO QUESTION PAPER 2019-20 SET A
Similar Questions
Knowledge Check
- Question 1 - Select One
The area of a parallelogram ABCD is equal to that right angled isosceles triangle whose hypotenuse is l cm. If O is a point inside the parallelogram ABCD then sum of areas of ΔAOBandΔCOD is.
Al22cm2Bl24cm2Cl28cm2Dl216cm2 - Question 2 - Select One
In an isosceles triangle ABC, AB=AC and ∠A=3∠B. Find ∠C.
A36∘B32∘C28∘D40∘
In the given figure (not to scale), ABC is an isosceles triangle in which AB=AC. AEDC is a parallelogram. If ∠CDF=70∘ and ∠BFE=180∘, then find ∠FBA.
 View Solution
View SolutionFind the ratio of area of ΔADE to Δ ABC
 View Solution
View SolutionIn the given figure, ABCD is a parallelogram. Find the area of ΔAED
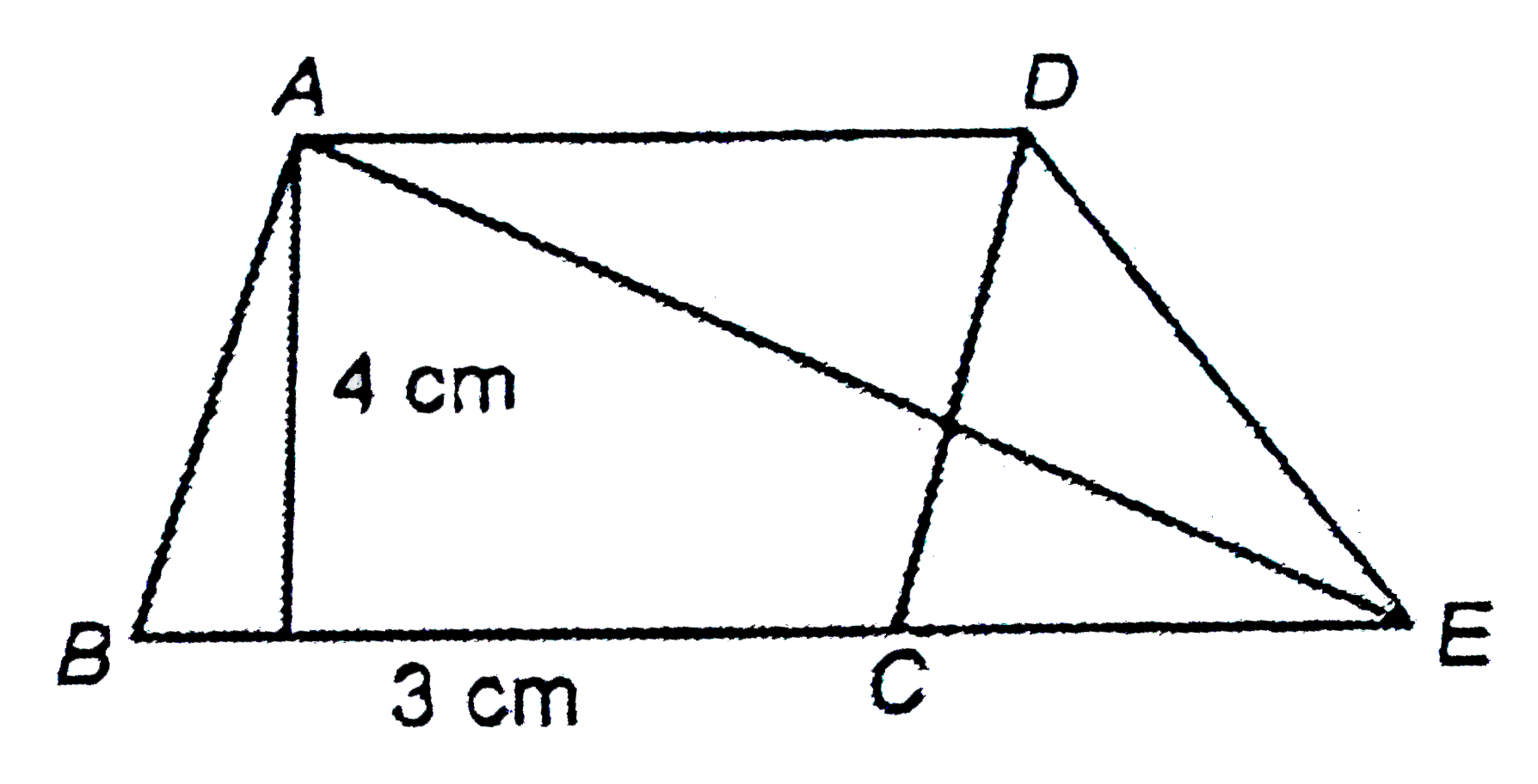 View Solution
View SolutionIn the adjoining figure, ΔABC is an isosceles triangle. Find the value of ∠BDC and ∠BEC.
 View Solution
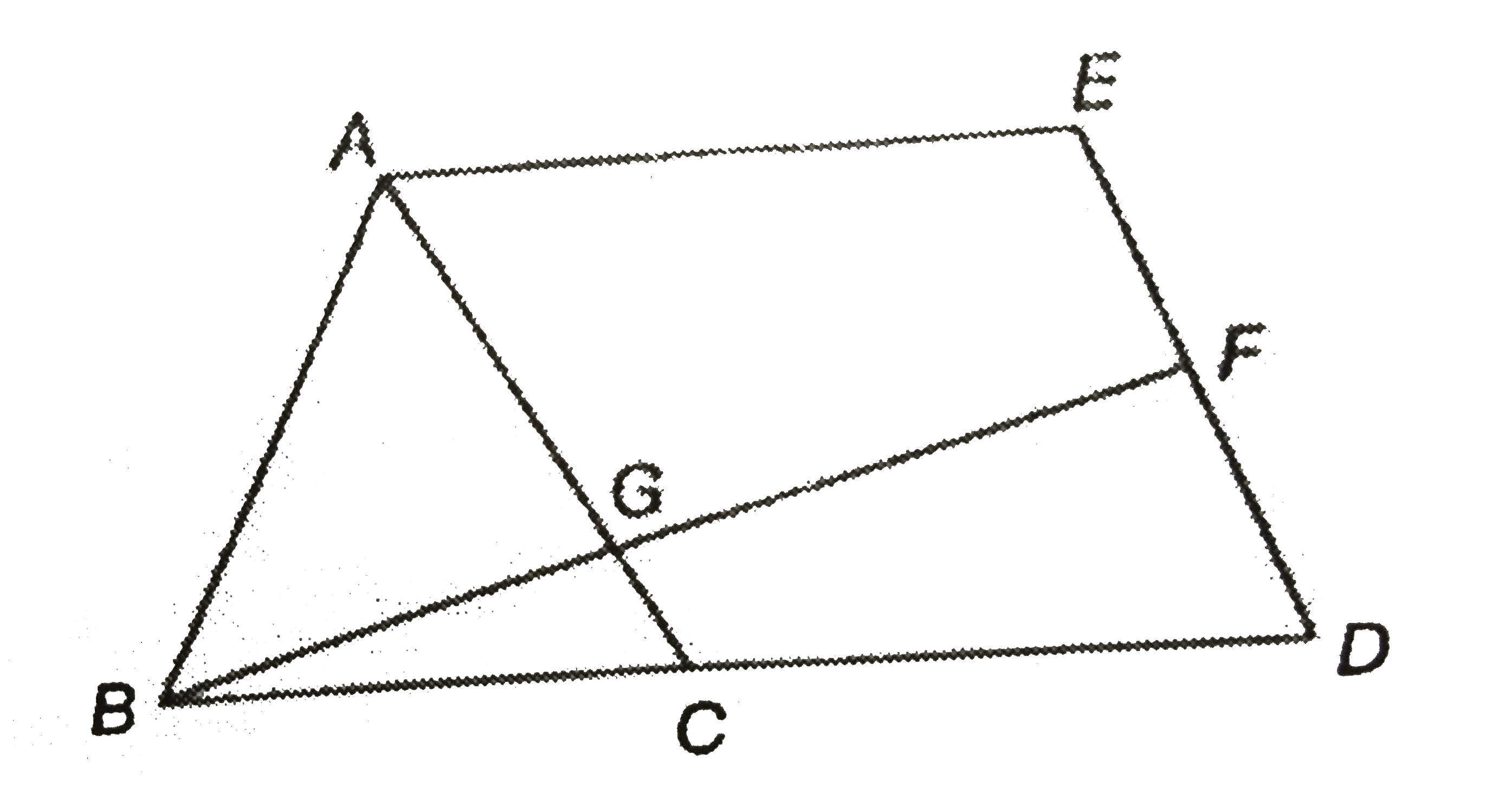
View SolutionIn the adjoning figure, ABCD is a parallelogram. Prove that : area of ΔBPC= area of ΔDPQ
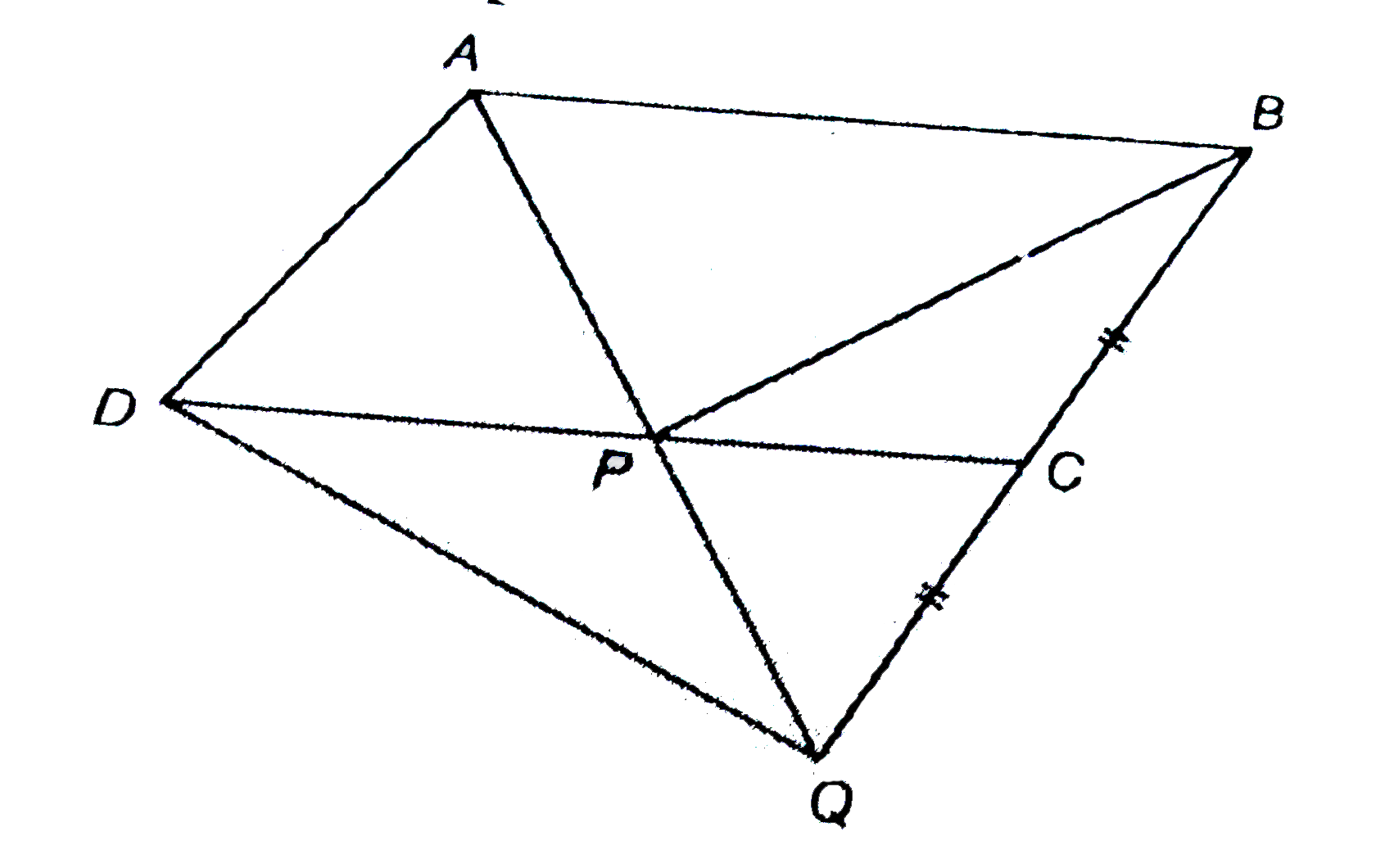 View Solution
View SolutionIn the figure given below, ABC is a triangle with AB = BC and D is an interior point of the triangle ABC such that ∠DAC=∠DCA.
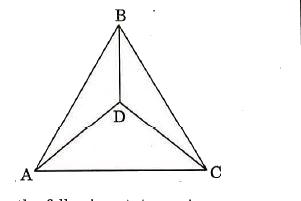
Consider the following statements :
1. Triangle ADC is an isosceles triangle.
2. Dis the centroid of the triangle ABC.
3. Triangle ABD is congruent to the triangle CBD.
Which of the above statements are correct ?View Solution