ifA={(x,y):x2+y2=4,x,y∈R}and
B={(x,y):Y=|x|,x,y∈R} then
A∩B=ϕ
A∩B is singlcton set
A∩B contains two elements only
A∩B contains three elements only .
The correct Answer is:C
To solve the problem, we need to find the intersection of the two sets A and B.
Step 1: Define the sets
Set A is defined by the equation x2+y2=4. This represents a circle centered at the origin (0,0) with a radius of 2.
Set B is defined by the equation y=|x|. This represents a V-shaped graph that opens upwards, consisting of two lines: y=x for x≥0 and y=−x for x<0.
Step 2: Graph the sets
To visualize the problem, we can sketch both graphs:
- The circle from set A will intersect the y-axis at (0,2) and (0,-2) and the x-axis at (2,0) and (-2,0).
- The V-shaped graph from set B will intersect the y-axis at (0,0) and will have lines extending outwards from this point.
Step 3: Find the intersection points
To find the intersection points, we need to solve the equations simultaneously.
1. Substitute y=|x| into the circle's equation x2+y2=4.
- For y=x (when x≥0):
x2+x2=4⟹2x2=4⟹x2=2⟹x=√2 (since x≥0)
Thus, y=√2. So one intersection point is (√2,√2).
- For y=−x (when x<0):
x2+(−x)2=4⟹2x2=4⟹x2=2⟹x=−√2 (since x<0)
Thus, y=−√2. So the other intersection point is (−√2,−√2).
Step 4: Conclusion
The intersection A∩B contains the points (√2,√2) and (−√2,−√2). Therefore, the intersection of sets A and B contains 2 elements.
Final Answer:
The intersection A∩B={(√2,√2),(−√2,−√2)}.
---
Set A is defined by the equation x2+y2=4. This represents a circle centered at the origin (0,0) with a radius of 2.
To visualize the problem, we can sketch both graphs:
- The circle from set A will intersect the y-axis at (0,2) and (0,-2) and the x-axis at (2,0) and (-2,0).
- The V-shaped graph from set B will intersect the y-axis at (0,0) and will have lines extending outwards from this point.
To find the intersection points, we need to solve the equations simultaneously.
- For y=x (when x≥0):
x2+x2=4⟹2x2=4⟹x2=2⟹x=√2 (since x≥0)
Thus, y=√2. So one intersection point is (√2,√2).
x2+(−x)2=4⟹2x2=4⟹x2=2⟹x=−√2 (since x<0)
Thus, y=−√2. So the other intersection point is (−√2,−√2).
The intersection A∩B contains the points (√2,√2) and (−√2,−√2). Therefore, the intersection of sets A and B contains 2 elements.
The intersection A∩B={(√2,√2),(−√2,−√2)}.
Topper's Solved these Questions
- SETSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - SETSEXERCISE - Chapter Test
30 Videos
- SETSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - SETSEXERCISE - Section I - Solved Mcqs
24 Videos
- SEQUENCES AND SERIESBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - SEQUENCES AND SERIESEXERCISE - Chapter Test
59 Videos
- STRAIGHT LINESBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - Chapter Test
34 Videos
Similar Questions
ifA={(x,y):x2+y2=4,x,y∈R}and
B={(x,y):x2+y2=9,x,y∈R},then
View SolutionIn some question of sets, we have to make the use of graphs
For example
A={(x,y):y=ex,x∈R}
B={{x,y}:y=−x.x∈R}
Find n(A∩B)
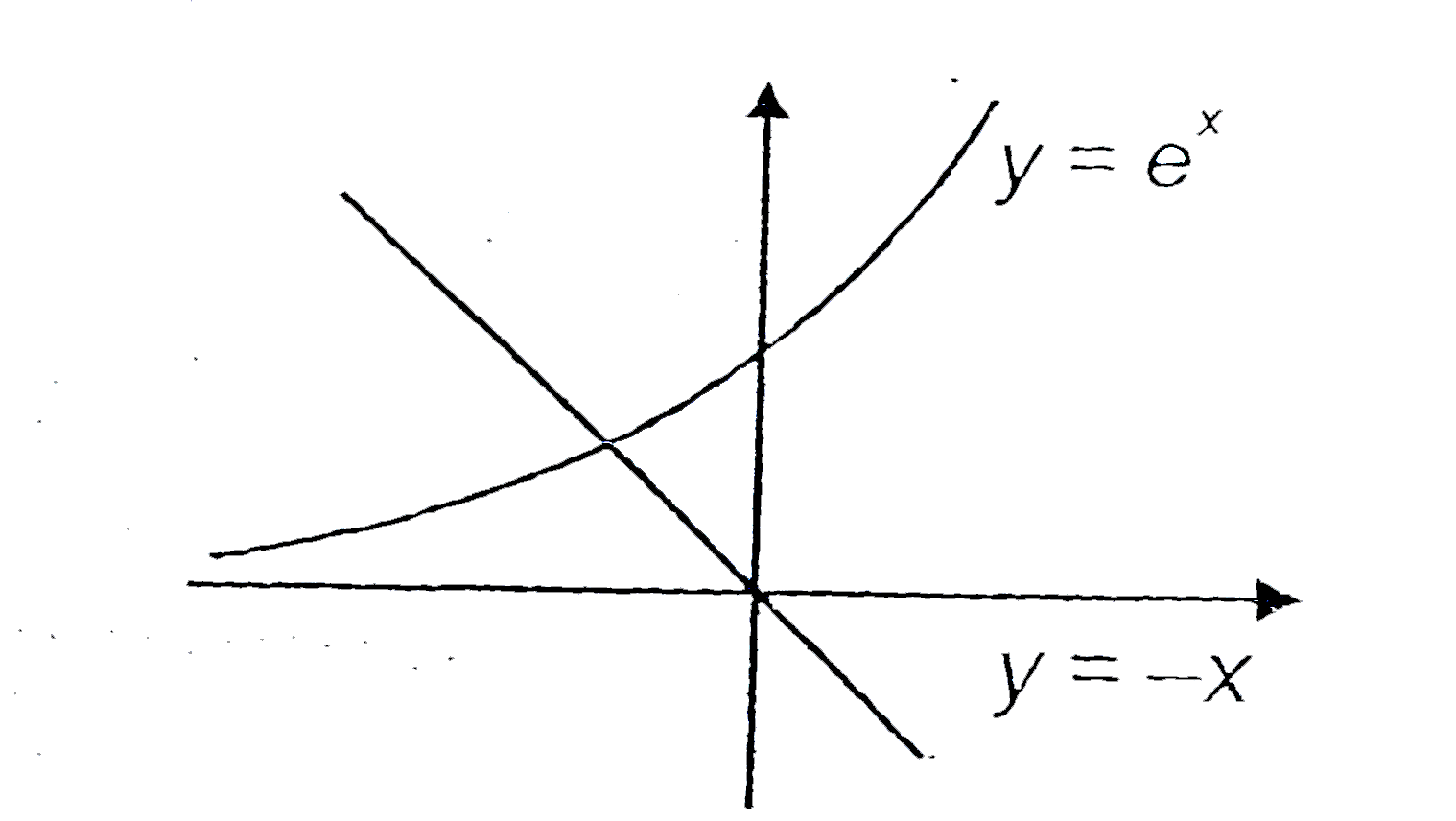
It is clear that y=exandy=−x intersect at one pont. Hence n(A∩B)=1
A={(x,y):x2+y2≤2,x,y∈R}
B={{x,y):y≥x2,x,y∈R}
View SolutionIf A={(x,y}: y=3x,y∈R} and B={{x,y}:y=x,x,y∈R} then which of the following is true?
View SolutionWhich of the following are function: {(x,y):y2=x,x,y∈R} b. {(x,y):y=|x|,x,y∈R} c. {(x,y):x2+y2=1,x,y∈R} d. {(x,y):x2−y2=1,x,y∈R}
View SolutionIf A={(x, y):y=1x, 0≠x∈R}and B={(x,y):y=−x, x∈R} , then write A∩B.
View SolutionIf A={(x, y):y=ex, x∈R}and B={(x,y):y=e−x, x∈R} , then write A∩B.
View SolutionIn some question of sets, we have to make the use of graphs
For example
A={(x,y):y=ex,x∈R}
B={{x,y}:y=−x.x∈R}
Find n(A∩B)
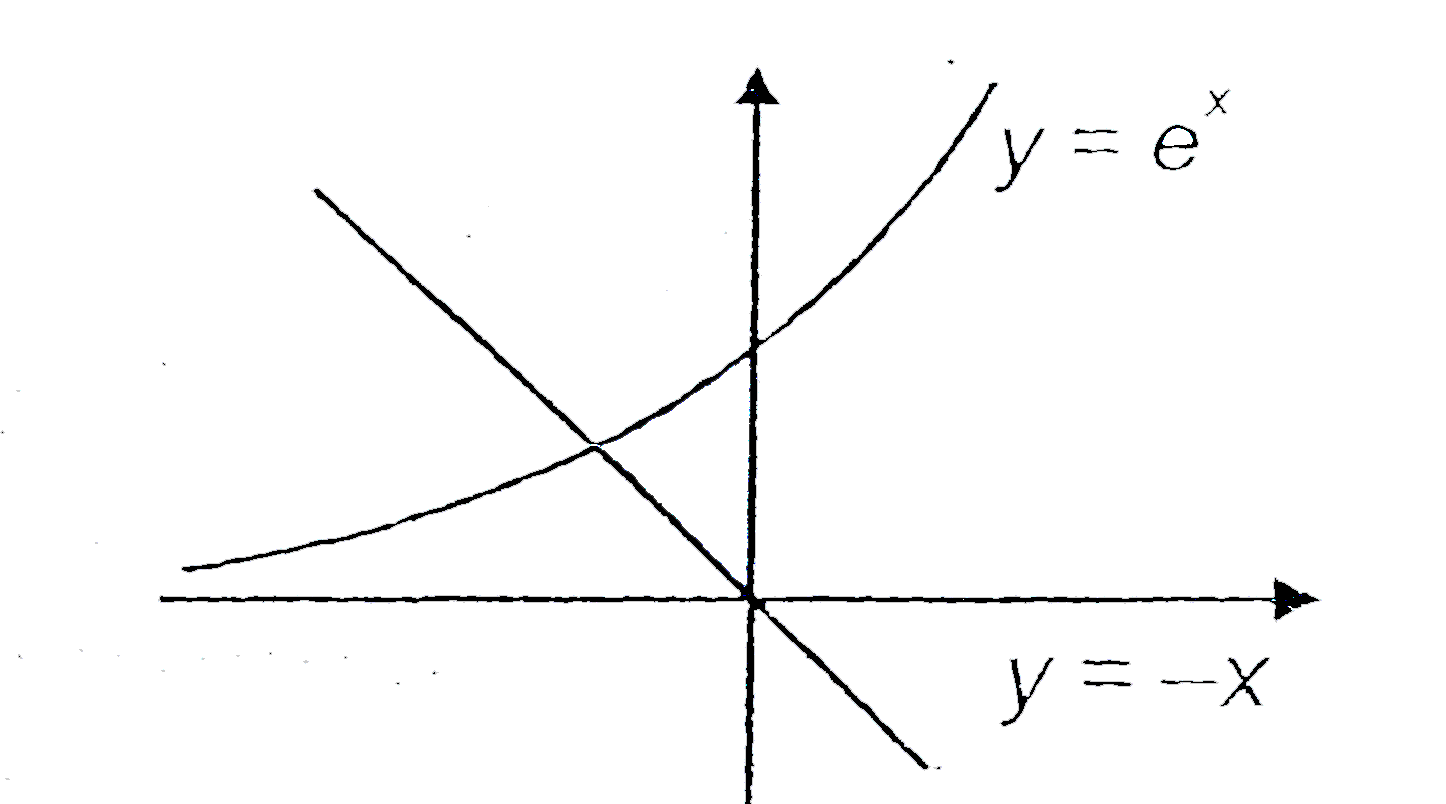
It is clear that y=exandy=−x intersect at one pont. Hence n(A∩B)=1
A:{(x,y):y=√4−x2,x∈[−2,2]}
B={(x,y):y=|x|,x∈R}
Then n(A∩B)
View SolutionifA={(x,y):x2+y2≤1,x,y∈R]and
B={(x,y):x2+y2≤x2+y2≤4,x,y∈R]then
View SolutionIf the sets A and B are defined are defined as A={(x,y):y=ex,x∈R},B={(x,y):y=x,x∈R}then
View SolutionA point chanrge of magnitude q=4.5nC is moving with speed v=3.6×107ms−1 parallel to the x-axis along the line y=3m. Find the magnetic field at the origin produced by this charge when the charge is at the point x=−4m, y=3m, as shown in Fig.

View Solution
