If f(x)=x3+4x2+λx+1 is a monotonically decreasing function of x in the largest possible interval (−2,−23). Then λ=4 (b) λ=2 λ=−1 (d) λ has no real valueSolution in Tamil
Here f'(x)≤0
⇒ 3x2+8x+λ0∀x∈(−2,−23)
Then the situations for f'(x) are as follows :
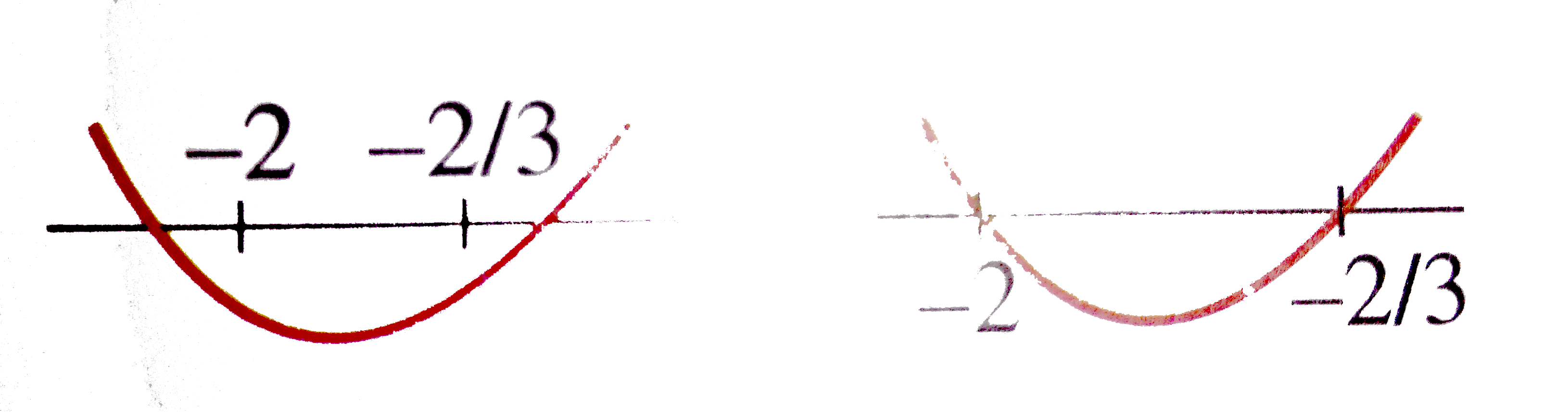
Given that f(x) decreases in the largest possible interval (−2,−23), then f'(x)=0 must have roots -2 and −2/3.
So the product of the roots is (−2)(−23)=λ3⇒λ=4.
Topper's Solved these Questions
- GRAPHS OF ELEMENTARY FUNCTIONS BOOK - CENGAGECHAPTER - GRAPHS OF ELEMENTARY FUNCTIONS EXERCISE - Exercise
34 Videos
- GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSBOOK - CENGAGECHAPTER - GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSEXERCISE - Exercises
4 Videos
- GRAPHS OF TRIGONOMETRIC FUNCTIONSBOOK - CENGAGECHAPTER - GRAPHS OF TRIGONOMETRIC FUNCTIONSEXERCISE - Exercise
22 Videos
Similar Questions
Draw the graph of y=1(1/x).
View SolutionSketch the graph of an example of a rational function f that satisfies all the given conditions.
(i) f(0)=0,f(1),limx→∞f(x)=0,f is odd
(ii) limx→0+f(x)=∞,limx→0−f(x)=−∞,limx→∞f(x)=1,limx→−∞f(x)=1
(iii) limx→−2f(x)=∞,limx→−∞f(x)=3,limx→∞f(x)=3,f(0)=0
View SolutionDraw the graph of y= cosec 2x−cot2x. Is f(x) periodic ? If yes, what is its fundamental period ?
View SolutionThe function f(x)=x2+λx has a (a)minimum at x=2ifλ=16 (b)maximum at x=2ifλ=16 (c)maximum for no real value of λ (d)point of inflection at x=1ifλ=−1
View SolutionIf f(x)=x3+4x2+λx+1 is a monotonically decreasing function of x in the largest possible interval (−2,−23). Then (a ) λ=4 (b) λ=2 (c) λ=−1 (d) λ has no real value
View SolutionThe values of λ for which the function f(x)=λx3−2λx2+(λ+1)x+3λ is increasing through out number line (a)λ∈(−3,3) (b) λ∈(−3,0) (c)λ∈(0,3) (d) λ∈(1,3)
View SolutionIf x=(4 lambda)/(1+lambda^(2)) and y=(2-2 lambda^(2))/(1+lambda^(2)) where lambda is a real parameter and Z=x^(2)+y^(2)-xy then possible values of Z can be
View SolutionFunction f(x)=cosx−2 λ x is monotonic decreasing when (a) λ>1/2 (b) λ<1/2 (c) λ<2 (d) λ>2
View SolutionIf f(x)=2x3+9x2+λx+20 is a decreasing function of x in the largest possible interval (-2,-1) then λ =
View SolutionIf aλ4+bλ3+cλ2+dλ+e=∣∣ ∣ ∣∣λ2−3λ−1λ+√3λ2+λ2λ−43λ+1λ2−3λ3λ+5λ−3∣∣ ∣ ∣∣ then |a| equals
View SolutionIf f(x)=x3+4x2+λx+1 is a monotonically decreasing function of x in the largest possible interval (−2,−23). Then (a ) λ=4 (b) λ=2 (c) λ=−1 (d) λ has no real value
View SolutionIf f(x)=x3+4x2+λx+1 is a monotonically decreasing function of x in the largest possible interval (−2,−23). Then (a)λ=4 (b) λ=2 λ=−1 (d) λ has no real value
View SolutionIf f(x)=x3+4x2+λx+1 is a monotonically decreasing function of x in the largest possible interval (−2,−23). Then λ=4 (b) λ=2 λ=−1 (d) λ has no real value
View SolutionFunction f(x)=cosx−2 λ x is monotonic decreasing when (a) λ>1/2 (b) λ<1/2 (c) λ<2 (d) λ>2
View SolutionIf f(x)=x3+4x2+λx+1 is a monotonically decreasing function of x in the largest possible interval (−2,−2/3) . Then
View SolutionIf f(x)=2x3+9x2+λx+20 is a decreasing function fo x in the largest possible interval (-2,-1) then λ =
View Solution
