Question

If $\left(\frac{x}{a}\right)+\left(\frac{y}{b}\right)=1$
and $\left(\frac{x}{c}\right)+\left(\frac{y}{d}\right)=1$
intersect the axes at four concylic points and $a^2+c^2=b^2+d^2,$
then these lines can intersect at, $(a,b,c,d>0)$

Options

A. (1,1)
B. (1,-1)
C. (2,-2)
D. (3,3)

Answer

A, B, C and D

Explanation

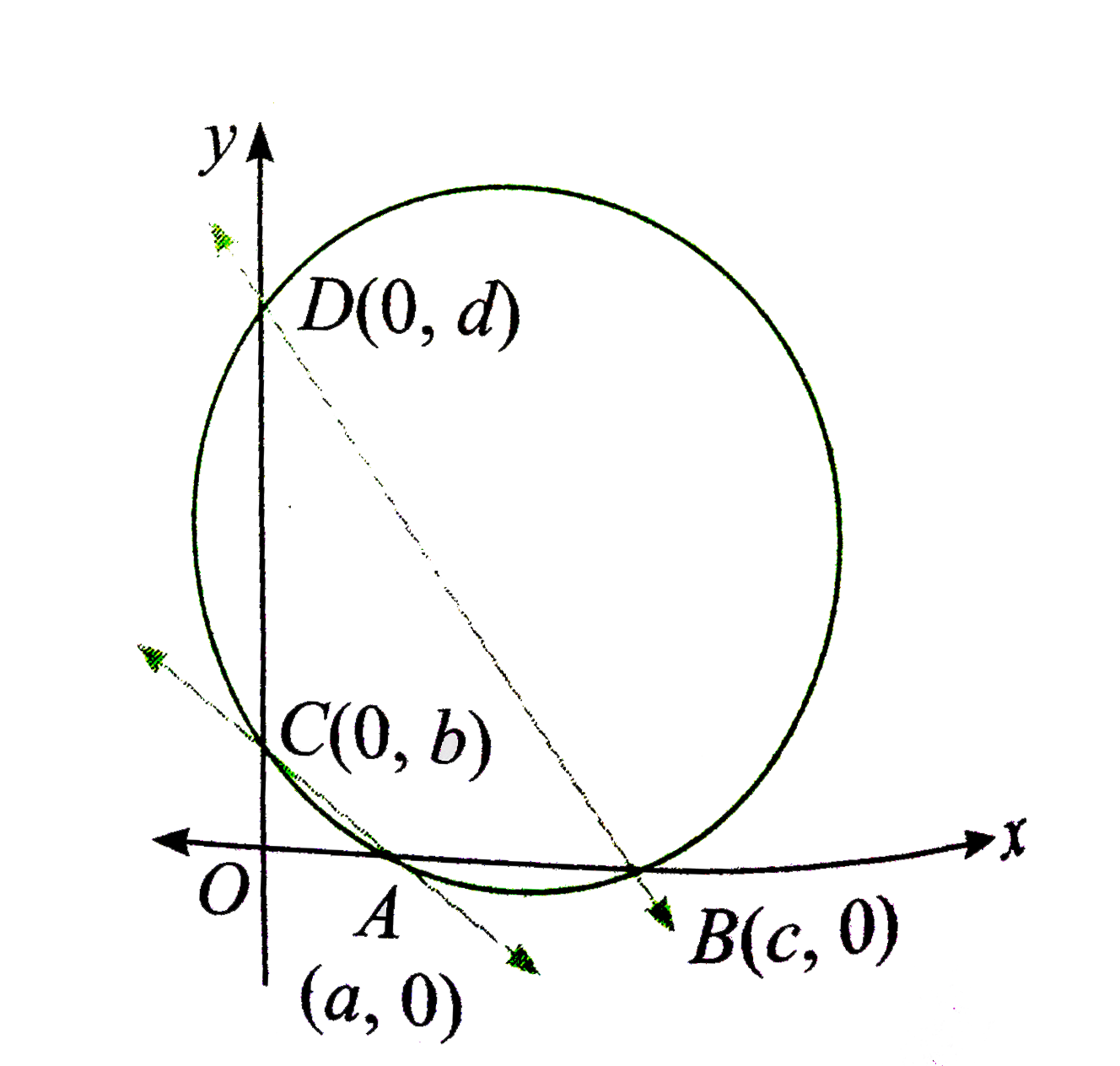
If points A, B,C,D are concyclic, then ac=bd. The coordinates of the points of intersection of lines are
`((ac(b-d))/(bc-ad), (bd(c-a))/(bc-ad))`
Let the coordinates of the point of intersection be (h,k). Then
`h= (ac(b-d))/(bc-ad), k= (bd(c-a))/(bc-ad)`
`"Given "c^(2) + a^(2) = b^(2)+d^(2). "Since "ac=bd," we have"`
`(c-a)^(2) = (b-d)^(2)`
`"or " (c-a)=+-(b-d)`
Then the locus of the points of intersection is `y=+-x.`