In a class of 175 students the following data shows the number of students opting one or more subjects Mathematics 100, physics 70, chemistry 28, physics and Chemistry 23, mathematics and chemistry 28 , mathematics and physics 30 , mathematics physics and chemistry 18. how many students have offered mathematics alone ?
35
48
60
22
The correct Answer is:C
To find the number of students who have opted for Mathematics alone, we will use the principle of inclusion-exclusion and the given data.
Step 1: Define the variables
Let:
- M: Number of students who opted for Mathematics = 100
- P: Number of students who opted for Physics = 70
- C: Number of students who opted for Chemistry = 28
- x: Number of students who opted for all three subjects (Mathematics, Physics, Chemistry) = 18
- y: Number of students who opted for both Mathematics and Physics but not Chemistry
- z: Number of students who opted for both Mathematics and Chemistry but not Physics
- d: Number of students who opted for both Physics and Chemistry but not Mathematics
Step 2: Set up equations based on the given data
From the problem, we have:
1. P∩C=23 (Physics and Chemistry)
- This gives us the equation: x+d=23
- Substituting x=18:
18+d=23⟹d=5
2. M∩C=28 (Mathematics and Chemistry)
- This gives us the equation: x+z=28
- Substituting x=18:
18+z=28⟹z=10
3. M∩P=30 (Mathematics and Physics)
- This gives us the equation: x+y=30
- Substituting x=18:
18+y=30⟹y=12
Step 3: Calculate the number of students who opted for Mathematics alone
The total number of students who opted for Mathematics can be expressed as:
M=b+y+z+x
Where b is the number of students who opted for Mathematics alone.
Substituting the known values:
100=b+12+10+18
Simplifying:
100=b+40
Thus,
b=100−40=60
Conclusion
The number of students who have opted for Mathematics alone is 60.
Let:
- M: Number of students who opted for Mathematics = 100
- P: Number of students who opted for Physics = 70
- C: Number of students who opted for Chemistry = 28
- x: Number of students who opted for all three subjects (Mathematics, Physics, Chemistry) = 18
- y: Number of students who opted for both Mathematics and Physics but not Chemistry
- z: Number of students who opted for both Mathematics and Chemistry but not Physics
- d: Number of students who opted for both Physics and Chemistry but not Mathematics
From the problem, we have:
1. P∩C=23 (Physics and Chemistry)
- This gives us the equation: x+d=23
- Substituting x=18:
18+d=23⟹d=5
- This gives us the equation: x+z=28
- Substituting x=18:
18+z=28⟹z=10
- This gives us the equation: x+y=30
- Substituting x=18:
18+y=30⟹y=12
The total number of students who opted for Mathematics can be expressed as:
M=b+y+z+x
Where b is the number of students who opted for Mathematics alone.
100=b+12+10+18
Simplifying:
100=b+40
Thus,
b=100−40=60
The number of students who have opted for Mathematics alone is 60.
Topper's Solved these Questions
- SETSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - SETSEXERCISE - Chapter Test
30 Videos
- SETSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - SETSEXERCISE - Section I - Solved Mcqs
24 Videos
- SEQUENCES AND SERIESBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - SEQUENCES AND SERIESEXERCISE - Chapter Test
59 Videos
- STRAIGHT LINESBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - Chapter Test
34 Videos
Similar Questions
A class has 175 students. The following data shows the number of students obtaining one or more subjects. Mathematics 100, Physics 70, Chemistry 40, Mathematics and Physics 30, Mathematics and Chemistry 28, Physics and Chemistry 23, Mathematics, Physics and Chemistry 18. How many students have offered Mathematics alone (a) 35 (c) 60 (b) 48 (d) 22
View SolutionIn a class of 55 students, the number of students studying different subjects are 23 in Mathematics, 24 in Physics, 19 in Chemistry, 12 in Mathematics and Physics, 9 in Mathematics and Chemistry, 7 in Physics and Chemistry and 4 in all the three subjects. Find the number of students who have taken exactly one subject.
View SolutionIn a class of 55 students the number of students studying subjects are , 23 iin Mathematics 24.in physics 19in chemisty 12 in Mathematics and physics ,9 in Mathematics and chemistry ,7 in physics and chemistry and 4 in all the three subjects.
the number of students who have taken ecactly one subject is
View SolutionFrom 50 students taking examinations in mathematics, physics and chemistry,37 passed mathematics, 24 physics and 43 chemistry. At most 19 passed mathematics and physics, at most 29 mathematics and chemistry and at most 20 physics and chemistry. Find the largest possible number that could have passed all three exams.
View SolutionFrom 50 students taking examinations in mathematics, physics and chemistry,37 passed mathematics, 24 physics and 43 chemistry. At most 19 passed mathematics and physics, at most 29 mathematics and chemistry and at most 20 physics and chemistry. Find the largest possible number that could have passed all three exams.
View SolutionA class has 175 students. The following table shows the number of students studying one or more of the following subjects in this case.
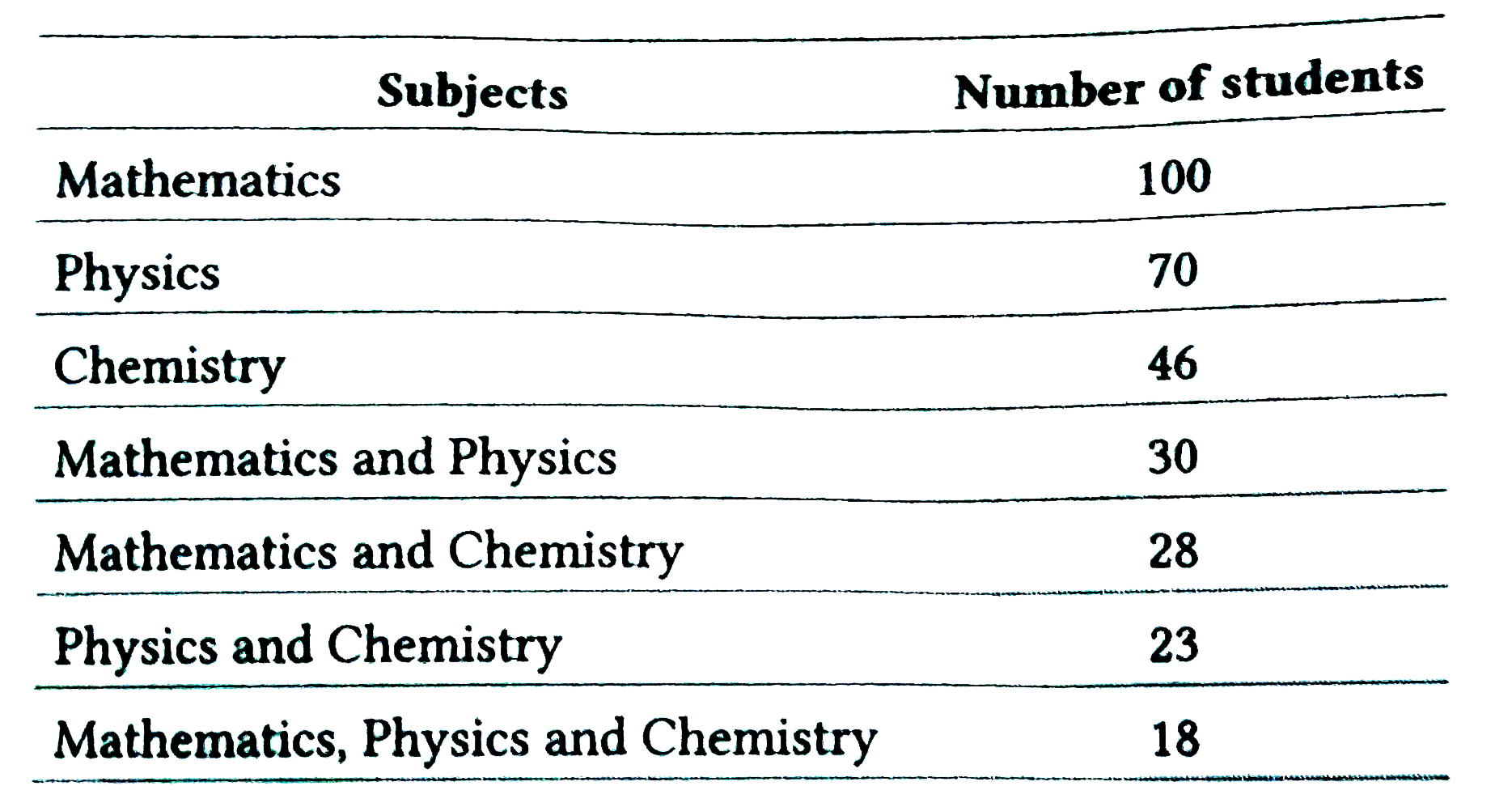
How many students are enrolled in Mathematics alone, Physics alone and Chemistry alone? Are there students who have not offered any one of these subjects?
View SolutionThere are 11 teachers who teach Mathematics or Physics in a school, Of these, 7 teach Mathematics and 3 teach both Mathematics and Physics. How many teach Physics?
View SolutionIf in a class there are 200 students in which 120 take Mathematics, 90 take Physics, 60 take Chemistry, 50 take Mathematics & Physics, 50 take Mathematics & Chemistry, 43 take Physics & Chemistry and 38 take Mathematics Physics & Chemistry, then the number of students who hace taken exactly one subject is
View SolutionThe number of ways of selecting 8 books from a library which has 9 books each of Mathematics, Physics, Chemistry and English is:
View SolutionIn a school there are 20 teachers who teach mathematics or physics. Of these, 12 teach mathematics and 4 teach both physics and mathematics. How many teach physics?
View Solution
