In if D is any point on the side BC, show that

Video Solution
Text Solution
GIVEN A in which D is a point on BC and AD is drawn.
TO PROVE
PROOF We know that in a triangle, the sum of any two sides is always greater than the third side.
So, in , we have
And, in , we have
Adding the corresponding sides of (i) and (ii), we get
Hence,
|
Topper's Solved these Questions
- CONGRUENCE OF TRIANGLES AND INEQUALITIES IN A TRIANGLEBOOK - RS AGGARWALCHAPTER - CONGRUENCE OF TRIANGLES AND INEQUALITIES IN A TRIANGLEEXERCISE - Exercise 9A
27 Videos
- CONGRUENCE OF TRIANGLES AND INEQUALITIES IN A TRIANGLEBOOK - RS AGGARWALCHAPTER - CONGRUENCE OF TRIANGLES AND INEQUALITIES IN A TRIANGLEEXERCISE - Exercise 9B
16 Videos
- CIRCLES BOOK - RS AGGARWALCHAPTER - CIRCLES EXERCISE - MULTIPLE CHOICE QUESTION(MCQ)
37 Videos
- COORDINATE GEOMETRYBOOK - RS AGGARWALCHAPTER - COORDINATE GEOMETRYEXERCISE - Multiple Choice Questions (Mcq)
22 Videos
Similar Questions
Knowledge Check
In ΔABC, AB=AC and D is a point on side AC such that BD = BC, If AB = 12.5 cm and BC = 5 cm, then what is the measure of DC ?
A2 cmB2.5 cmC3 cmD1.8 cmIn ΔABC,∠B=2∠C. D is a point on side BC such that AD bisects ∠BACandAD=CD. Find the value of ∠BAC.
A36∘B72∘C108∘D120∘In a ΔABC , point D is on side AB and point E is on side Ac , such that BCED is a trapezium . If DE : BC = 3 : 5 , then area (ΔADE): area (ΔBCED)=
A3:4B9:16C3:5D9:25
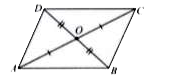
In the figure, show that 2(AC+BD)>AB+BC+CD+DA.
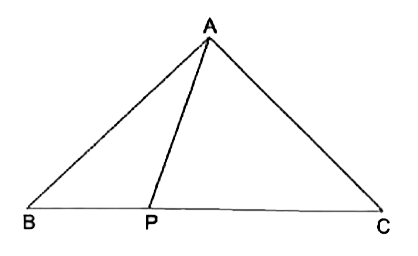
View SolutionIn the given figure ,P is a point on the side BC of △ABC.Prove tat (AB+BC+AC)GT2AP
View SolutionIn △ABC,AB>AC and D is any point on BC. Prove that, AB > AD.
View SolutionD is a point on side BC of ΔABC such that AD=AC. Show that AB>AD.
View SolutionD is a point on the side BC of a ΔABC such that AD bisects ∠BAC . Then
View Solution
