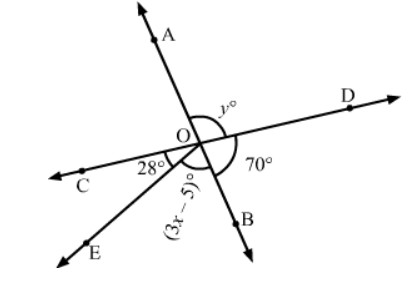
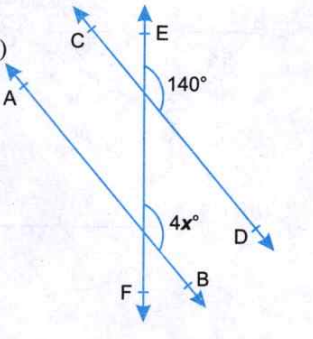
In Fig.98, If AB is parallel to CD and EF is a transversal, then x= (a) 19 (b) 29 (c) 39 (d) 49
To solve the problem step by step, we will use the properties of parallel lines and transversals.
1. Identify the Angles: Given that AB is parallel to CD and EF is a transversal, we can identify the angles formed by the transversal with the two parallel lines. Let's denote the angles as follows:
- Angle 1 = 7x−12
- Angle 2 = 4x+17
2. Use the Co-Interior Angles Property: Since AB is parallel to CD and EF is the transversal, the angles on the same side of the transversal (Angle 1 and Angle 2) are co-interior angles. According to the property of co-interior angles, their sum is equal to 180 degrees.
(7x−12)+(4x+17)=180
3. Combine Like Terms: Now, we will combine the like terms in the equation.
7x+4x−12+17=180
11x+5=180
4. Isolate the Variable: Next, we will isolate x by subtracting 5 from both sides of the equation.
11x=180−5
11x=175
5. Solve for x: Finally, divide both sides by 11 to find the value of x.
x=17511
x≈15.91
6. Check the Options: The calculated value of x is approximately 15.91, which does not match any of the provided options (19, 29, 39, 49). Therefore, it seems there might be an error in the options provided.
Final Answer:
The value of x is approximately 15.91, which is not listed among the options.
- Angle 1 = 7x−12
- Angle 2 = 4x+17
(7x−12)+(4x+17)=180
7x+4x−12+17=180
11x+5=180
11x=180−5
11x=175
x=17511
x≈15.91
The value of x is approximately 15.91, which is not listed among the options.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
In the figure, AB is parallel to CD. What is the value of x?
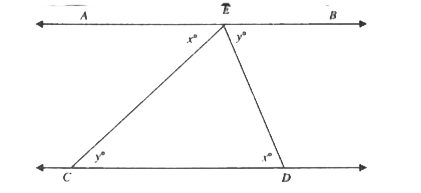 A36B45C60DCannot be determined
A36B45C60DCannot be determined
In Fig. AB∣∣DC . Find the value of x
View SolutionIn Fig. if AB∣∣CD , find the value of x .
View SolutionIn Fig.111, if ABCD then x= (a) 154 (b) 139 (c) 144 (d) 164
View SolutionIn each of the following figures, AB∣∣CD and EF is a transversal. Find the value of x in each case
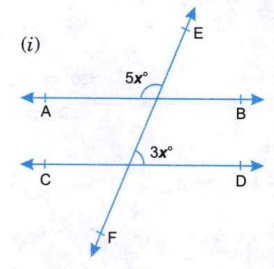 View Solution
View SolutionIn each of the following figures, AB∣∣CD and EF is a transversal. Find the value of x in each case
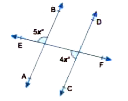 View Solution
View SolutionIn each of the following figures, AB∣∣CD and EF is a transversal. Find the value of x in each case
 View Solution
View SolutionIf 47 of 49% of x is 21, then x=
(a) 125
(b) 98
(c) 84
(d) 75View Solution