In Figure, ABCD is a trapezium in which ABCD and AD=BC. show that : ∠A=∠B (ii) ∠C=∠D ABC≅BAD diagonal AC=diagonalBD
Given ABCD is trapezium where AD=BC
To prove, ∠A=∠B
we can see that AECD is a parallelogram, so sum of adjacent angles=1800
∠A+∠E=1800<br>∠A+x=1800<br>∠A=1800−x=∠B
Hence proved.
(ii) To prove,∠C=∠D,
sum of adjacent angles in parallelogram is π
so,∠D∠C+1800−2x=1800
∠C+∠D=2x∠B+∠C=1800
1800−x+∠C=1800
∠C=x,∠D=x
∠C=∠D Hence proved.
(iii)△ABC=△BADside AB is common.
AD=BC(given)
so the angle including both the sides is also same.
∠A=∠B
△ABC=△BAD(By SAS congruent Rule)
Hence proved.
(iv) As △ABC=△BAD
The third side of both triangles i.e. diagonals are equal AC=BD
Topper's Solved these Questions
Similar Questions
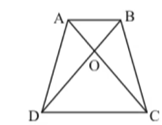
In Figure, ABCD is a trapezium in which AB∣∣CD . Prove that: ar(AOD)=ar(BOC).
 View Solution
View SolutionIn figure, ABC is a triangle in which ∠ABC=90∘and AD⊥BC. Prove that AC2=AB2+BC2−2BC.BD.
 View Solution
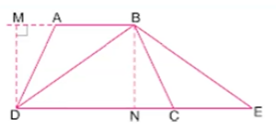
View SolutionIn Figure, ABCD is a trapezium in which AB∣∣DC, DC is produced to E such that CE=AB, prove that ar(ABD)=ar (BCE). Construction: Draw DM on BA produced and BN⊥DC
 View Solution
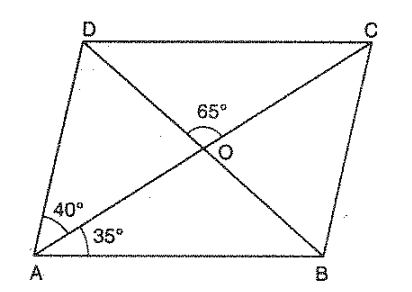
View SolutionIn Figure, ABCD is a parallelogram in which ∠DAO=400, ∠BAO=350 and ∠COD=650. Find: ∠ABO (ii) ∠ODC (iii) ∠ACB (iv) ∠CBD
 View Solution
View SolutionIn figure, ABC is triangle in which ∠ABC=90∘and AD⊥CBproduced. Prove that AC2=AB2+BC2−2BC.BD.
 View Solution
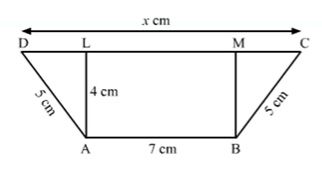
View SolutionIn Figure, ABCD is a trapezium in which AB=7cm, AD=BC=5cm, DC=x cm, and distance between AB and DC is 4cm . Find the value of x and area of trapezium ABCD
 View Solution
View SolutionIn Figure, ABD is a triangle right-angled at A and AC⊥BD. Show that
(i)AB2=BC.BD
(ii) AC2=BC.DC
(iii) AD2=BD.CD
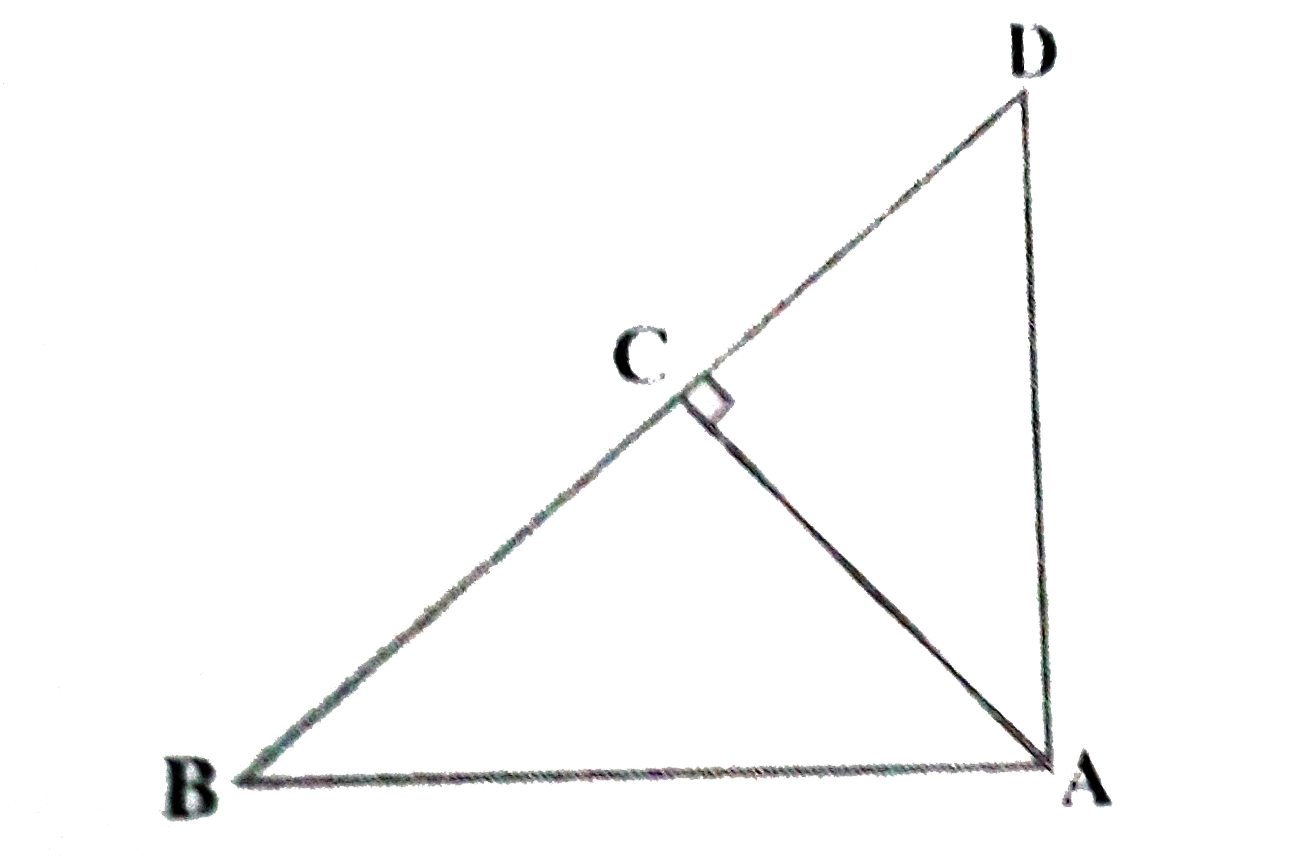 View Solution
View SolutionIn Figure, ABCD is a rectangle in which diagonal AC is produced to E. If ∠ECD=1460, find ∠AOB
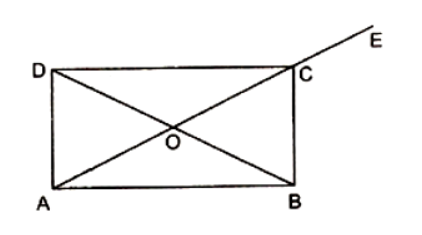 View Solution
View SolutionIn Figure, it is given that AB=BC and AD=EC. Prove that abe ≅CBD (ii) BD=BE
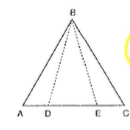 View Solution
View SolutionIn figure OA∗OB=OC∗OD. Show that ∠A=∠Cand ∠B=∠D.
View Solution

