Question

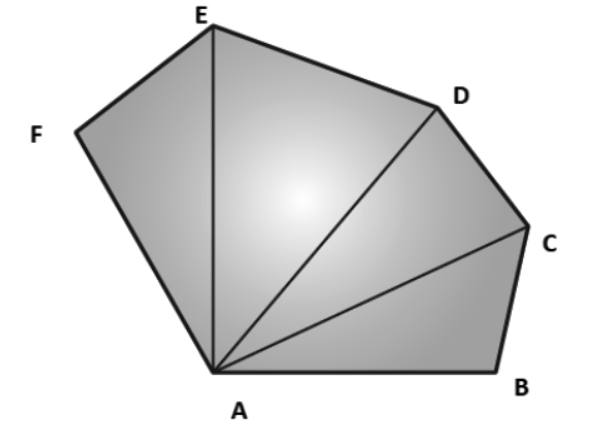
In five cornered fig. 18,$AD,AC$
are joined. Find
$\angle EAB+\angle ABC+\angle BCD+\angle CDE+\angle DEA$
.

Explanation

To solve the problem, we need to find the sum of the angles $$\angle EAB+\angle ABC+\angle BCD+\angle CDE+\angle DAE$$ in the given five-cornered figure (pentagon).

1. Identify the Angles:
- Let’s label the angles in the pentagon ABCDE:
- \( \angle EAB = \angle 1 + \angle 8 + \angle 9 \)
- \( \angle ABC = \angle 2 \)
- \( \angle BCD = \angle 3 + \angle 4 \)
- \( \angle CDE = \angle 5 + \angle 6 \)
- \( \angle DAE = \angle 7 \)

2. Use the Angle Sum Property of Triangles:
- In triangle ABC:
\( \angle 1 + \angle 2 + \angle 3 = 180^\circ \quad \text{(Equation 1)} \)
- In triangle ACD:
\( \angle 9 + \angle 5 + \angle 4 = 180^\circ \quad \text{(Equation 2)} \)
- In triangle ADE:
\( \angle 6 + \angle 7 + \angle 8 = 180^\circ \quad \text{(Equation 3)} \)

3. Add the Equations:
- Now, we will add all three equations:
\( (\angle 1 + \angle 2 + \angle 3) + (\angle 9 + \angle 5 + \angle 4) + (\angle 6 + \angle 7 + \angle 8) = 180^\circ + 180^\circ + 180^\circ \)
- This simplifies to:
\( \angle 1 + \angle 2 + \angle 3 + \angle 4 + \angle 5 + \angle 6 + \angle 7 + \angle 8 + \angle 9 = 540^\circ \)

4. Conclusion:
- Therefore, the sum of the angles \( \angle EAB + \angle ABC + \angle BCD + \angle CDE + \angle DAE \) is:
\( \angle EAB + \angle ABC + \angle BCD + \angle CDE + \angle DAE = 540^\circ \)

Final Answer:
The sum of the angles \( \angle EAB + \angle ABC + \angle BCD + \angle CDE + \angle DAE \) is \( 540^\circ \).