In parallelogram ABCD, E is the mid-point of AB and AP is parallel to EC which meets DC at point O and BC produced at P. Prove that :
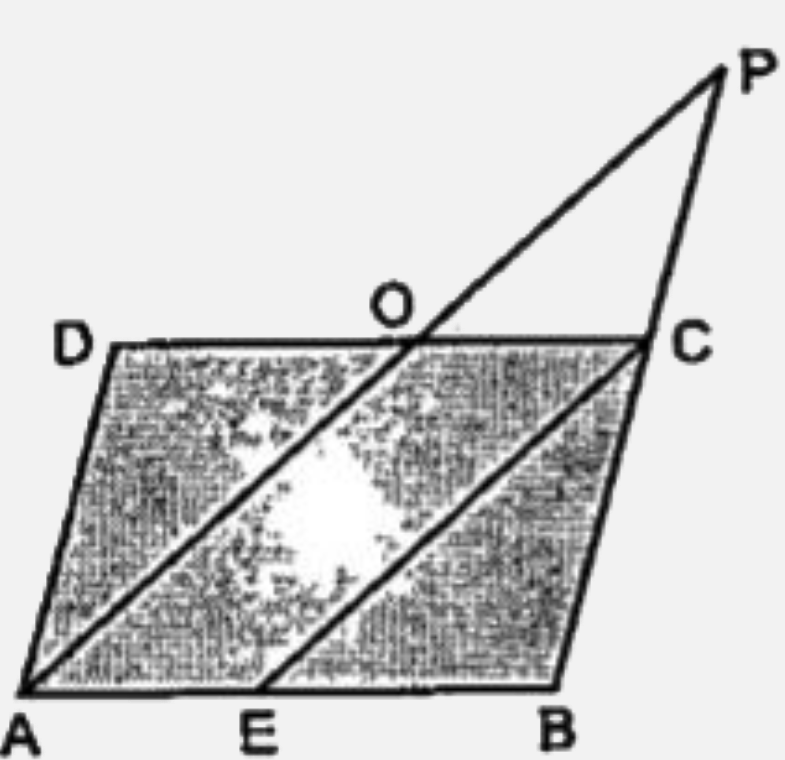
BP=2AD
Step by step video & image solution for In parallelogram ABCD, E is the mid-point of AB and AP is parallel to EC which meets DC at point O and BC produced at P. Prove that : BP=2AD by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Topper's Solved these Questions
- BOOK - ICSECHAPTER - MID-POINT THEOREMEXERCISE - 4 MARKS QUESTION15videos
MID-POINT THEOREM
- BOOK - ICSECHAPTER - MID-POINT AND ITS CONVERSE(INCLUDING INTERCEPT THEOREM)EXERCISE - EXERCISE 12(B)23videos
MID-POINT AND ITS CONVERSE(INCLUDING INTERCEPT THEOREM)
- BOOK - ICSECHAPTER - PYTHAGORAS THEORAMEXERCISE - QUESTIONS9videos
PYTHAGORAS THEORAM
Similar Questions
In parallelogram ABCD, E is the mid-point of AB and AP is parallel to EC which meets DC at point O and BC produced at P. Prove that:
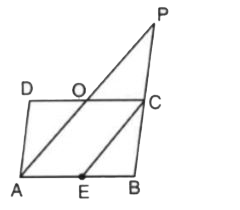
PB=2ADView SolutionIn parallelogram ABCD, E is the mid-point of AB and AP is parallel to EC which meets DC at point O and BC produced at P. Prove that:
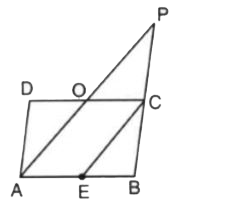
O is mid-point of AP.View SolutionIn parallelogram PQRS. L is mid-point of side Sr and SN is drawn parallel to LQ which meets RQ produced prove that :
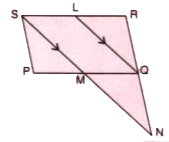
SN=2LQView SolutionIn parallelogram PQRS. L is mid-point of side SR and SN is drawn parallel to LQ which meets RQ produced prove that :
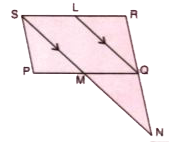
SP=12RNView SolutionIn parallelogram ABCD, E is the mid-point of AD and F is the mid-point of BC. Prove that BFDE is a parallelogram.
View SolutionIn parallelogram ABCD, E is mid-point of CD and through D, a line is drawr parallel to EB to meet CB produced at point G and to cut AB at point F. Prove that:
2×AD=GCView SolutionA parallelogram ABCD has P the mid-point of DC and Q a point of AC such that CQ=14AC.PQ produced meets BC at R. Prove that:
PR=12DB.View SolutionIn parallelogram ABCD, E is mid-point of CD and through D, a line is drawn parallel to EB to meet CB produced at point G and to cut AB at point F. Prove that :
DG = 2EBView SolutionA parallelogram ABCD has P the mid-point of DC and Q a midpoint of AC such that CQ=14AC.PQ produced meets BC at R. Prove that:
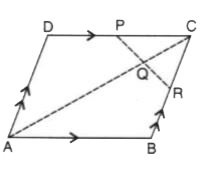
R is the mid-point of BCView SolutionIn the following figure, M is mid-point of BC of a parallelogram ABCD. DM intersects the diagonal AC at P and AB produced at E. Prove that : PE = 2 PD .
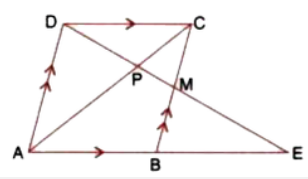 View Solution
View Solution
