In the adjoining figure ADE and ABC are two similar triangles, point D divides AB in the raito 2:1 and point E divides AC in the ratio 1:2 . If the area of △ADE is 23 square units, then find the ar(□DECB).
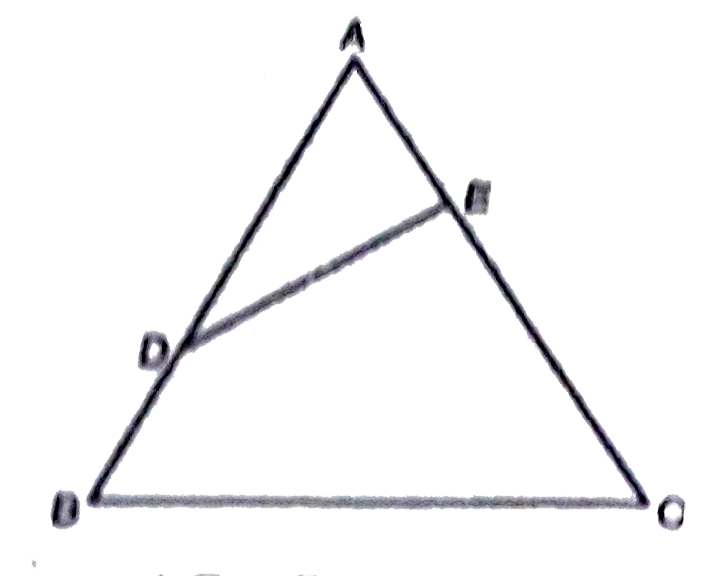
We have
ADDB=21andAEEC=12
Let AD = 2x ⇒DB=xandletAE=y⇒EC=2y⇒AC=3y
ADDB≠AEEC
DEcancel||BC.
∠D≠∠Band∠E≠∠C.
But since △ADEand△ABC are similar and ∠A is common to both the triangles.
△ADEand△ABC
∠A=∠A (common)
and ∠D=∠C (obvious)
△ADE~△ACB (AA corollary)
ADAC=DECB=AEAB⇒2x3y=y3x⇒6x2=3xy⇒2x=y
ar(△ADE)ar(△(ACB))=AD2AC2
if two triangles are similar then ratio of their areas is equal to the ratio of square of their corresponding sides)
4x29y2=(2x)29y2=y29y2=19
23ar(△ACB)=19
ar(△ACB)=23×9=209 sq units
ar(square DECB)=ar(△ABC)−ar(△ADE)
207-23= 184 sq units.
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLES EXERCISE - Problems From NCERT/ Exemplar14videos
TRIANGLES
- BOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLES EXERCISE - Exercise 6a23videos
TRIANGLES
- BOOK - NAGEEN PRAKASHANCHAPTER - STATISTICS EXERCISE - Revision Exercise Long Answer Questions5videos
STATISTICS
- BOOK - NAGEEN PRAKASHANCHAPTER - VOLUME AND SURFACE AREA OF SOLIDSEXERCISE - Revisions Exercise Long Answer Questions5videos
VOLUME AND SURFACE AREA OF SOLIDS
Similar Questions
Let ABC be a triangle. If P is a point such that AP divides BC in the ratio 2:3,BP divides CA in the ratio 3:5 then the ratio in which CP divides AB is
View SolutionIn the adjoining figure D, E and F are the mid - points of the sides BC, AC and AB respectively to triangle. Show that ∠EDF=∠A, ∠DEF=∠B and ∠DFE=∠C:
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
In the adjoining figure, P and Q are two points on equal sides AB and AC of a isosceles triangle ABC such that AP = AQ, then
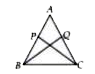 ABQ = CPB∠ABQ=∠ACPC∠BAQ=∠CPADboth (a) and (b)
ABQ = CPB∠ABQ=∠ACPC∠BAQ=∠CPADboth (a) and (b) - Question 2 - Select One
ABCD is a square , E is a point on AB such that BE=17 cm. The area of triangle ADE is 84 cm2 . What is the area of square ?
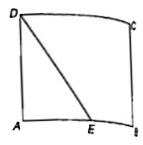 A400cm2B625cm2C729cm2D576cm2
A400cm2B625cm2C729cm2D576cm2
In ΔABCAD divides BC in 1 : 3 find the ratio of triangle ADB and ADC ?
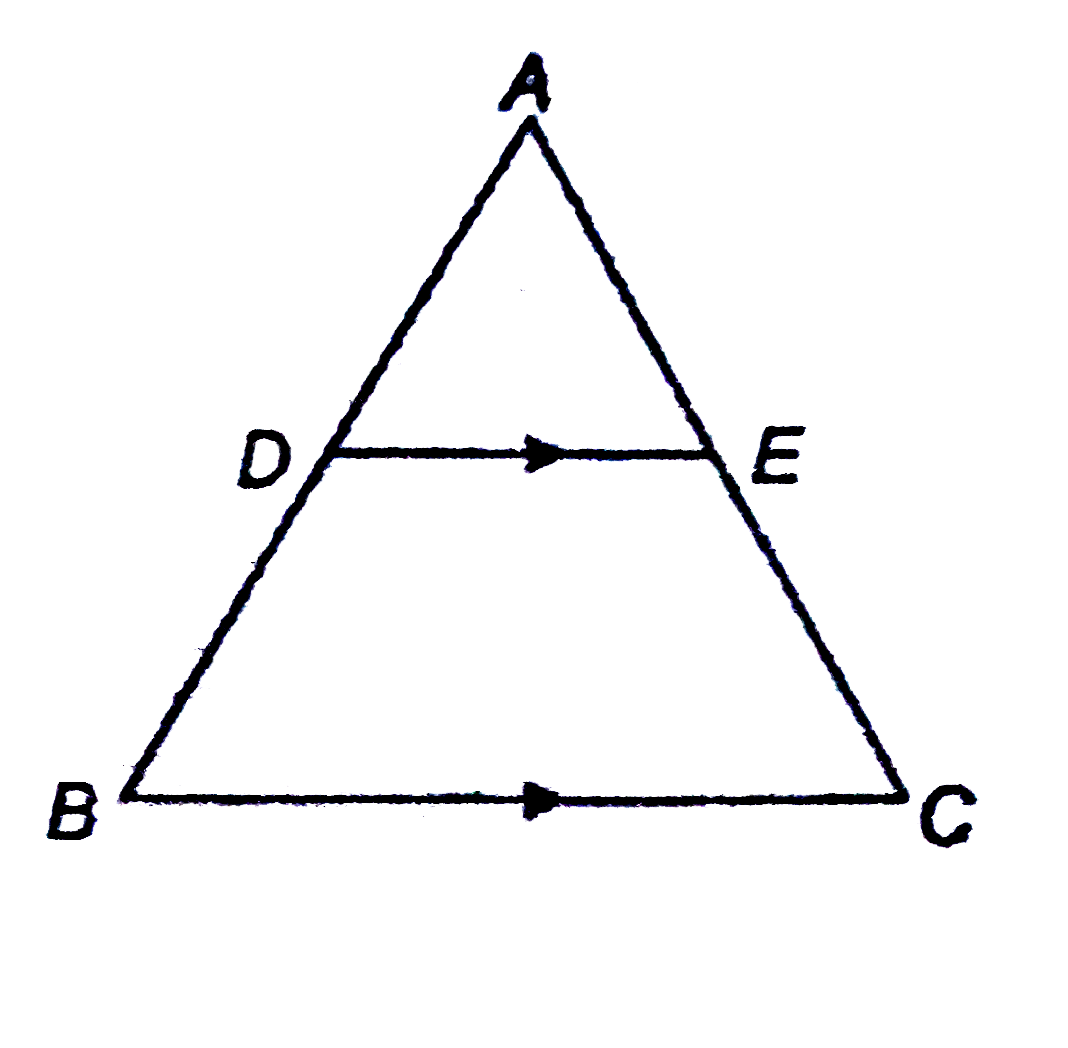
View SolutionIn the adjoining figure DE||BE and D divides AB in the ratio 2:3 find.
(i) AEEC (ii) AEAC
 View Solution
View SolutionIn the adjoining figure, ABC is a triangle and D is any point in its interior. Show that BD+DC<AB+AC.
 View Solution
View SolutionIn the adjoining figure, ABC is a triangle and D is any point in its interior. Show that (BD+DC)<(AB+AC).
 View Solution
View SolutionIn the adjoining figure D, E and F are the mid-points of the sides BC, AC and AB respectively. △DEF is congruent to triangle :
 View Solution
View SolutionA line perpendicular to the in segment joining the points (1,0) and (2,3) divides it in the ratio 1:n . Find the equation of the line.
View Solution

