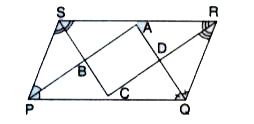
In the alongside diagram, the bisectors of interior angles of the parallelogram PQRS enclose a quadrilateral ABCD.
Show that:
(i)
(ii)
(iii)
(iv)
(v)
(vi) ABCD is a rectangle
thus, the bisectors of the angles of a parallelogram enclose a rectangle.
Step by step video solution for In the alongside diagram, the bisectors of interior angles of the parallelogram PQRS enclose a quadrilateral ABCD. Show that: (i) anglePSB+angleSPB=90^(@) (ii) anglePBS=90^(@) (iii) angleABC=90^(@) (iv) angleADC=90^(@) (v) angleA=90^(@) (vi) ABCD is a rectangle thus, the bisectors of the angles of a parallelogram enclose a rectangle. by Maths experts to help you in doubts & scoring excellent marks in Class 8 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
ABCD is a cyclic quadrilateral such that ∠A=90∘,∠B=70∘,∠C=95∘and∠D=105∘.
View SolutionConstruct a quadrilateral ABCD in which AB = 4.5 cm , BC = 3.8 cm ∠BCD=90∘∠BAD=60∘and∠ABC=120∘
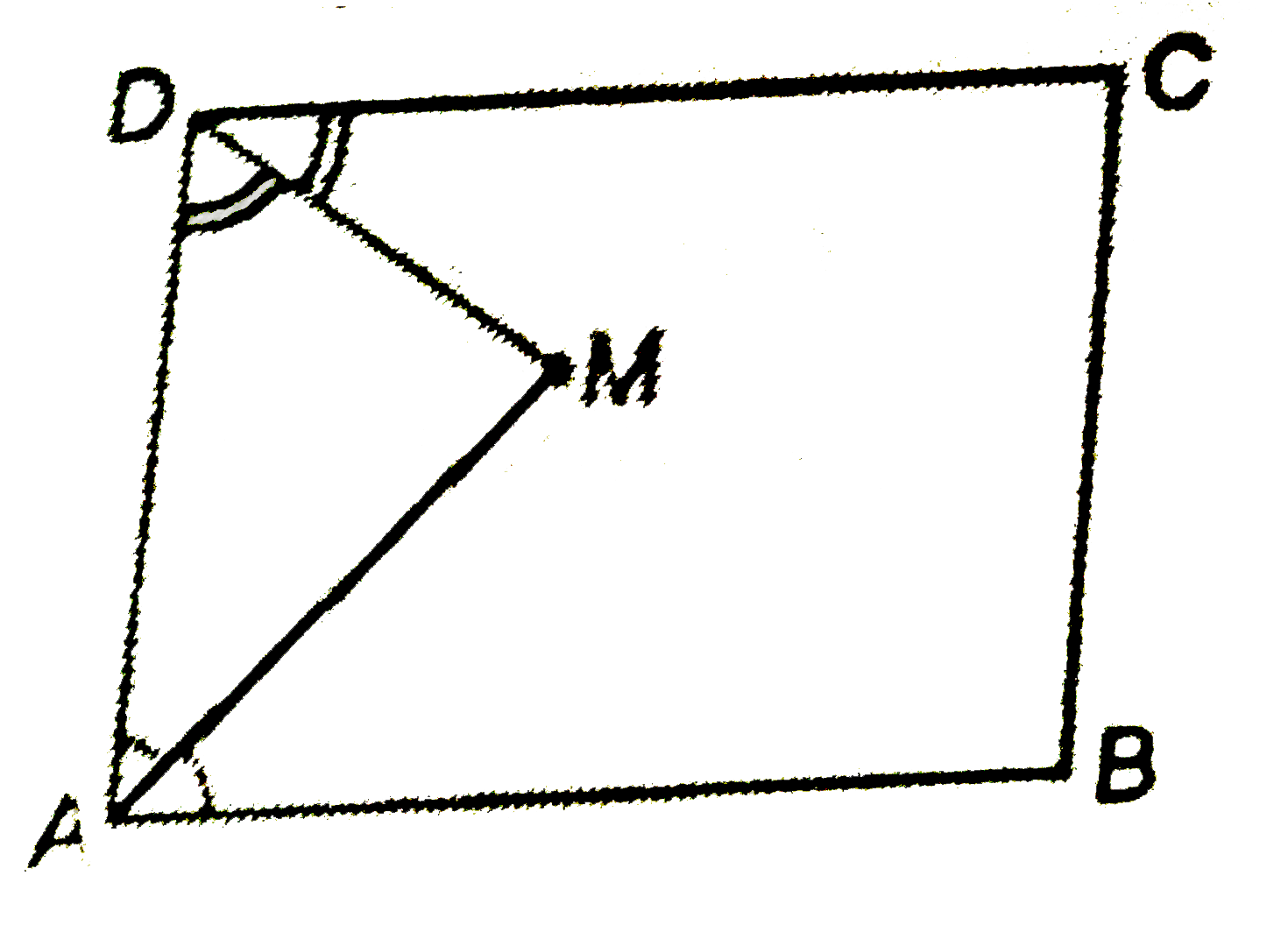
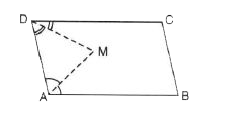
View SolutionIn the given figure, AM bisects angle A and DM bisects angle D of parallelogram ABCD. Prove that ∠AMD=90∘.
View SolutionThe bisectors of any two adjacent angles of a parallelogram intersect at:
View SolutionIn parallelogram ABCD, the bisector of angle A meets DC at P and AB = 2AD.
Prove that :
(i) BP bisects angle B.
(ii) Angle APB = 90∘
View SolutionIn the figure, given below, AM bisects angle A and DM bisects angle D of parallelogram ABCD. Prove that: ∠AMD=90∘
View SolutionIn a quadrilateral ABCD, ∠A+∠D=90∘. Prove that
AC2+BD2=AD2+BC2
View SolutionShow that, in a parallelogram ABCD, the internal and external bisectors of ∠Aand∠B from a rectangle.
View SolutionIn parallelogram ABCD, the bisectors of adjacent angles A and D intersect each other at point P. prove that ∠APD=90∘.
View SolutionIn parallelogram ABCD, the bisector of angle A meets DC at P and AB= 2AD. Prove that:
Angle APB= 90∘
View Solution
