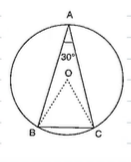
In the given figure, $∠ B A C = 30^{\circ}$ . Show that BC is equal to the radius of the circumcircle of $Δ A B C$ whose centre is O.

Video Solution

Text Solution

Verified by Experts

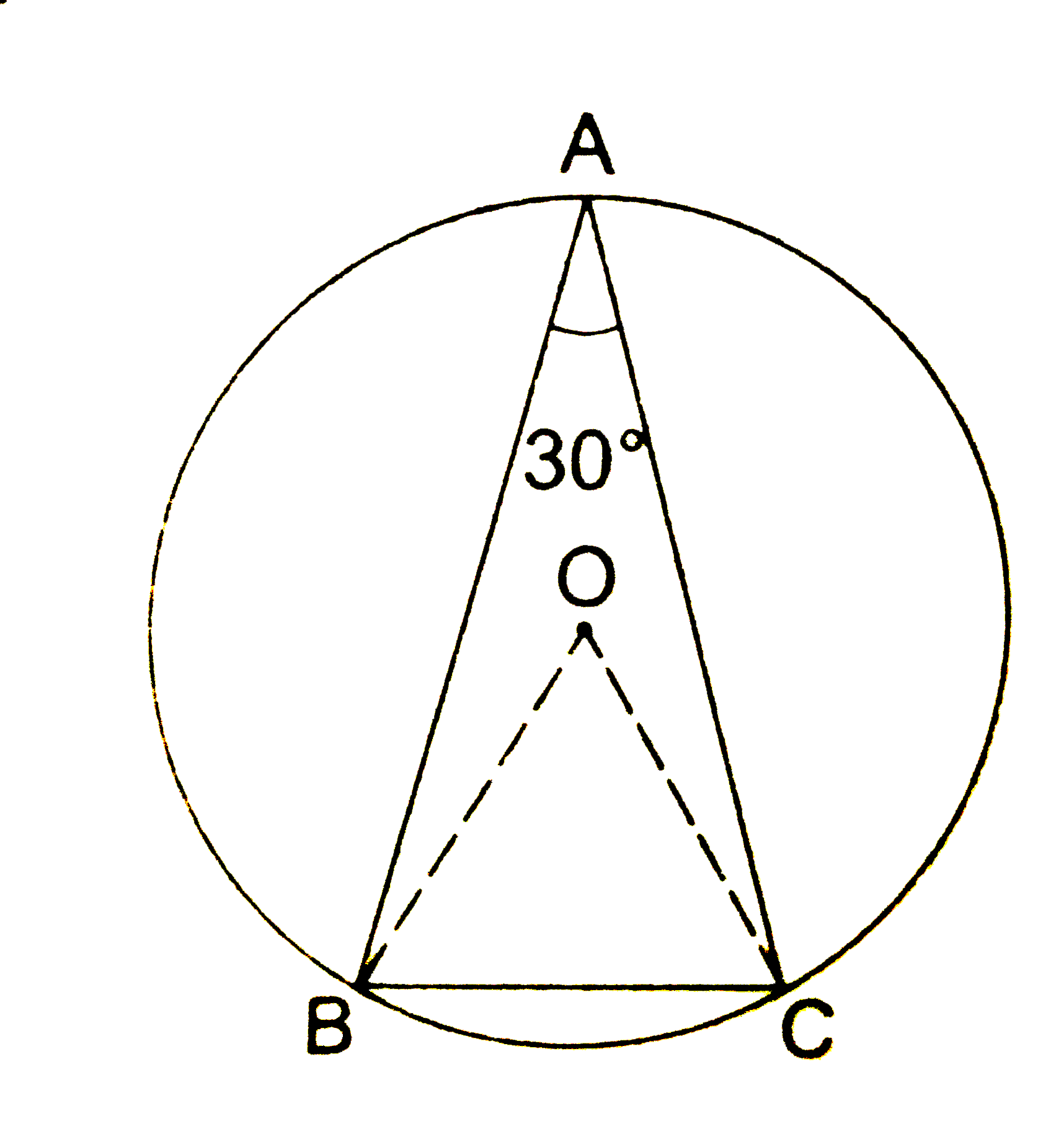
Join OB and OC.
$∠ B O C = 2 ∠ B A C = 2 \times 30^{\circ} = 60^{\circ}$
$O B = O C \Rightarrow ∠ O B C = ∠ O C B = x^{\circ}$ ( say )
$∴$ in $∠ O B C$ , we have
$∠ B O C + ∠ O B C + ∠ O C B = 180^{\circ} \Rightarrow 60^{\circ} + 2 x^{\circ} = 180^{\circ} \Rightarrow x = 60^{\circ}$
Thus, each angle of $Δ B O C$ is $60^{\circ}$
$∴ Δ B O C$ is an equilateral triangle
$∴ O B = O C = B C$
Hence, BC is the radius of the circumcircle.

Updated on:21/07/2023