Let ABC be a triangle such that AB=BC. Let F be midpoint of AB and X be a poin on BC such that FX is perpendicular to AB. If BX =3XC then the ratio BC/AC equals
√3
√2
√32
1
The correct Answer is:C
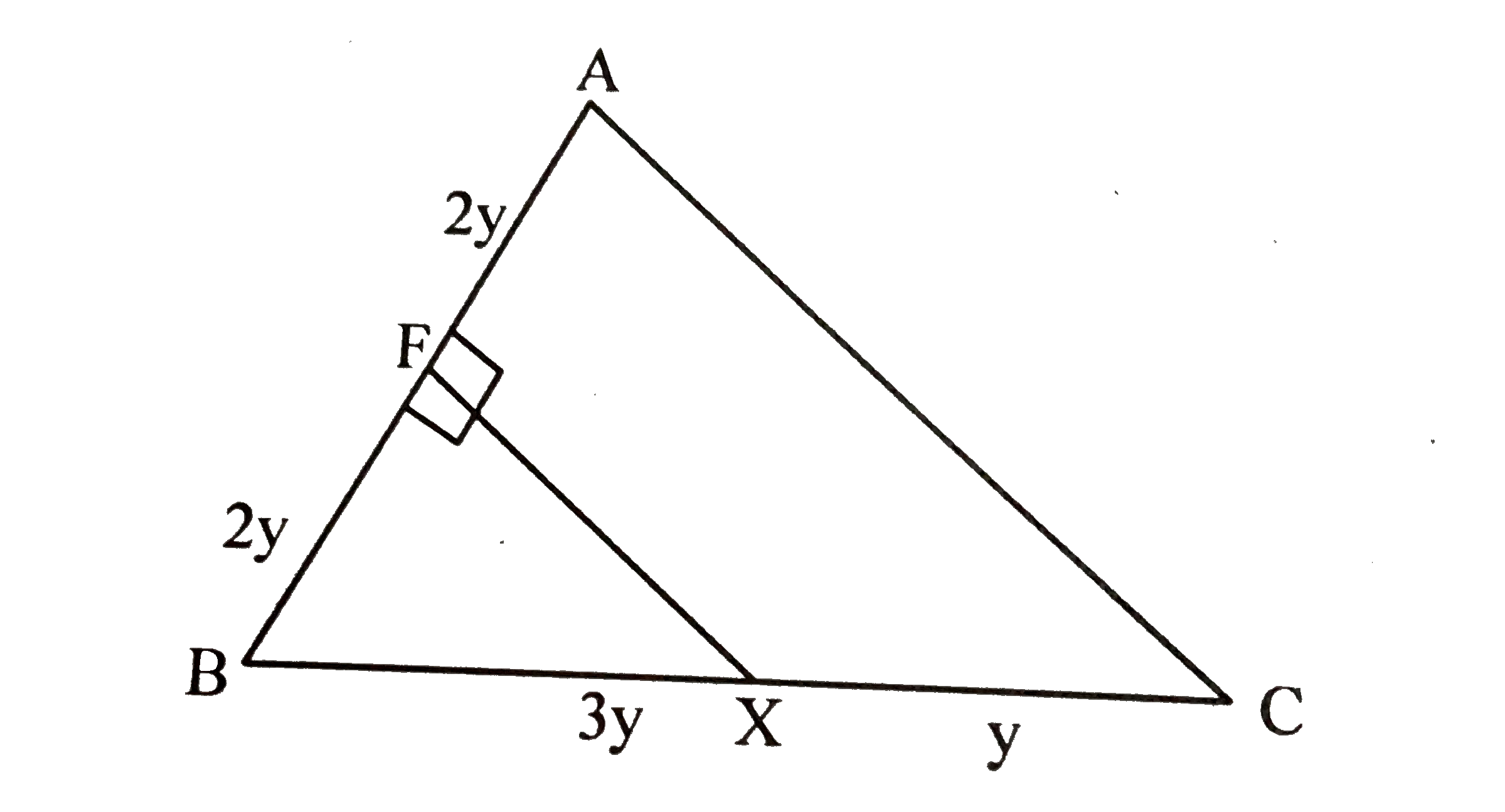
∴CosB=23
Also cosB=16y2+16y2−AC22.4y.4y
23=32y2−AC232y2
64y2=96y2−3Ac2
3AC2=32y2
AC=4√2√3y
∴BCAC=4y(4√2y√3)=√3√2
Topper's Solved these Questions
- KVPYBOOK - KVPY PREVIOUS YEARCHAPTER - KVPYEXERCISE - PART-II MATHEMATICS
10 Videos
- KVPYBOOK - KVPY PREVIOUS YEARCHAPTER - KVPYEXERCISE - Part A - Mathematics
20 Videos
- KVPYBOOK - KVPY PREVIOUS YEARCHAPTER - KVPYEXERCISE - PART-2(MATHEMATICS)
10 Videos
- KVPY 2021BOOK - KVPY PREVIOUS YEARCHAPTER - KVPY 2021EXERCISE - PART II MATHEMATICS
4 Videos
Similar Questions
Let ABC be a triangle with AB=AC. If D is the midpoint of BC,E is the foot of the perpendicular drawn from D to AC,andF is the midpoint of DE, then prove that AF is perpendicular to BE.
View Solutionlet ABC be a triangle with AB=AC. If D is the mid-point of BC, E the foot of the perpendicular drawn from D to AC, F is the mid-point of DE. Prove that AF is perpendicular to BE.
View Solution
Knowledge Check
In triangle ABC, AD is prependicular to BC and DE is perpendicular to AB
 AabcdprqqBabcdqrpsCabcdspqrDabcdrpsq
AabcdprqqBabcdqrpsCabcdspqrDabcdrpsqLet ABC be an acute-angled triangle and let D be the midpoint of BC. If AB = AD, then tan(B)/tan(C) equals
A√2B√3C2D3In ΔABC,AB=AC,Ois a point on BC such that BO = CO and OD is perpendicular to AB and OE is perpendicular to AC. If ∠BOD=30∘ then measure of ∠AOE is
A45∘B60∘C75∘D30∘
AB is a straight line, C and D are points on the same side of AB such that AC is perpendicular to AB and DB is perpendicular to AB. Let AD and BC meet at E.
What is AEAD+DEBC equal to ?
View SolutionLet ABC be an isosceles triangle with AB=AC and let D,E,F be the mid-points of BC,CA and AB respectively. Show that AD⊥FE and AD is bisected by FE.
"
View SolutionIf ΔABC is an isosceles triangle such that AB=AC, then altitude AD from A on BC bisects BC (Fig.43).
View SolutionLet ABC be a triangle such that AB = 15 and AC = 9. The bisector of ∠BAC meets BC in D. If ∠ACB=2∠ABC, then BD is
View SolutionIn triangle ABC,D is the midpoint of AB, E is the midpoint of DB and F is the midpoint of BC. If the area of ΔABC is 96, the area of ΔAEF is
View Solution
