Question

Let C be the circle $x^2+y^2=1$ in the xy-plane . For each $t\ge 0$, let $L_t$ be the line passing through (0,1) and (t,0) . Note than $L_t$ interesects C in two points, one of which is (0,1). Let $Q_t$ be the other point. As t varies between 1 and $1+\sqrt{2}$ , the collection of points $Q_1$ sweeps out an arc on C. The angle subtended by this arc at (0,0) is

Options

A. `(pi)/(8)`
B. `(pi)/(4)`
C. `(pi)/(3)`
D. `(3pi)/(8)`

Answer

B

Explanation

`x^(2)+y^(2)=1`
`L_(t)(x)/(t)+(y)/(1)=1`
`y=1-(x)/(t)`
`x^(2)+1+(x^(2))/(t^(2))-(2x)/(t)=1`
`x^(2)(1+(1)/(t^(2)))-(2x)/(t)=0`
`{:(x=0",",|," "x(1+1/(t^(2))) =2/t),(y=1," "x=(2t)/(t^(2)+1), y=1-2/(t^(2)-1),),((0","1)," "y=(t^(2)-1)/(t^(2)+1),):}`
`Q_(1)((2t)/(1+t^(2)),(t^(2)-1)/(t^(2)+1))`
`1letle1+sqrt(2) " " t=tan theta" " Q_(1)(sin2 theta,-cos 2 theta)`
`theta in(45^(@),67(1^(@))/(2)) " " ` lies on circle C

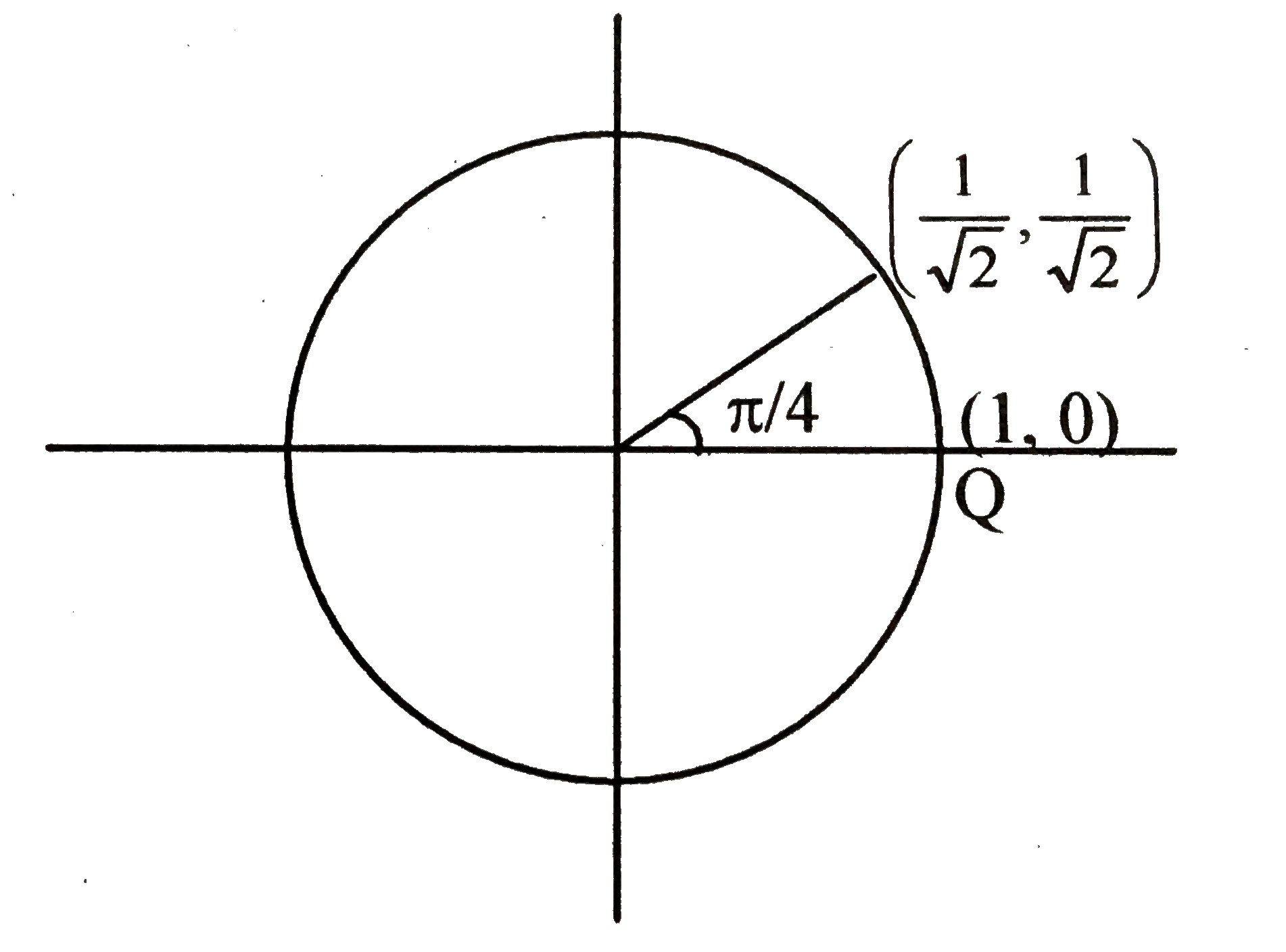
so angle center =`(pi)/(4)`