is the centre of a circle of radius 8 cm. The tangent at a point on the circle cuts a line through at such that . Find .
To solve the problem, we need to find the length of OB given that O is the center of a circle with a radius of 8 cm, AB=15 cm, and A is a point on the circle where the tangent at A meets the line through O at point B.
1. Identify the Given Data:
- Radius of the circle, OA=8 cm.
- Length of the tangent segment, AB=15 cm.
2. Draw the Diagram:
- Draw a circle with center O and radius OA.
- Mark point A on the circumference of the circle.
- Draw a tangent line at point A which meets the line OB at point B.
3. Understand the Relationship:
- The radius OA is perpendicular to the tangent line at point A. Therefore, ∠OAB=90∘.
4. Apply the Pythagorean Theorem:
- In the right triangle OAB:
OB2=OA2+AB2
- Substitute the known values:
OB2=82+152
- Calculate OA2 and AB2:
OA2=64(since 82=64)
AB2=225(since 152=225)
5. Sum the Squares:
- Now, add the two results:
OB2=64+225=289
6. Find OB:
- Take the square root of both sides to find OB:
OB=√289=17 cm
Final Answer:
Thus, the length of OB is 17 cm.
---
- Radius of the circle, OA=8 cm.
- Length of the tangent segment, AB=15 cm.
- Draw a circle with center O and radius OA.
- Mark point A on the circumference of the circle.
- Draw a tangent line at point A which meets the line OB at point B.
- The radius OA is perpendicular to the tangent line at point A. Therefore, ∠OAB=90∘.
- In the right triangle OAB:
OB2=OA2+AB2
- Substitute the known values:
OB2=82+152
- Calculate OA2 and AB2:
OA2=64(since 82=64)
AB2=225(since 152=225)
- Now, add the two results:
OB2=64+225=289
- Take the square root of both sides to find OB:
OB=√289=17 cm
Thus, the length of OB is 17 cm.
Topper's Solved these Questions
Similar Questions
A line through the centre O of a circle of radius 7 cm cuts the tangent, at a point P on the circle, at Q such that PQ=24cm . Find OQ .
View SolutionIf the tangent at a point P to a circle with centre O cuts a line through O at Q such that PQ=24cm and OQ=25cm . Find the radius of the circle.
View SolutionIn Figure, O is the centre of circle of radius 28 cm. Find the area of minor segment ASB .
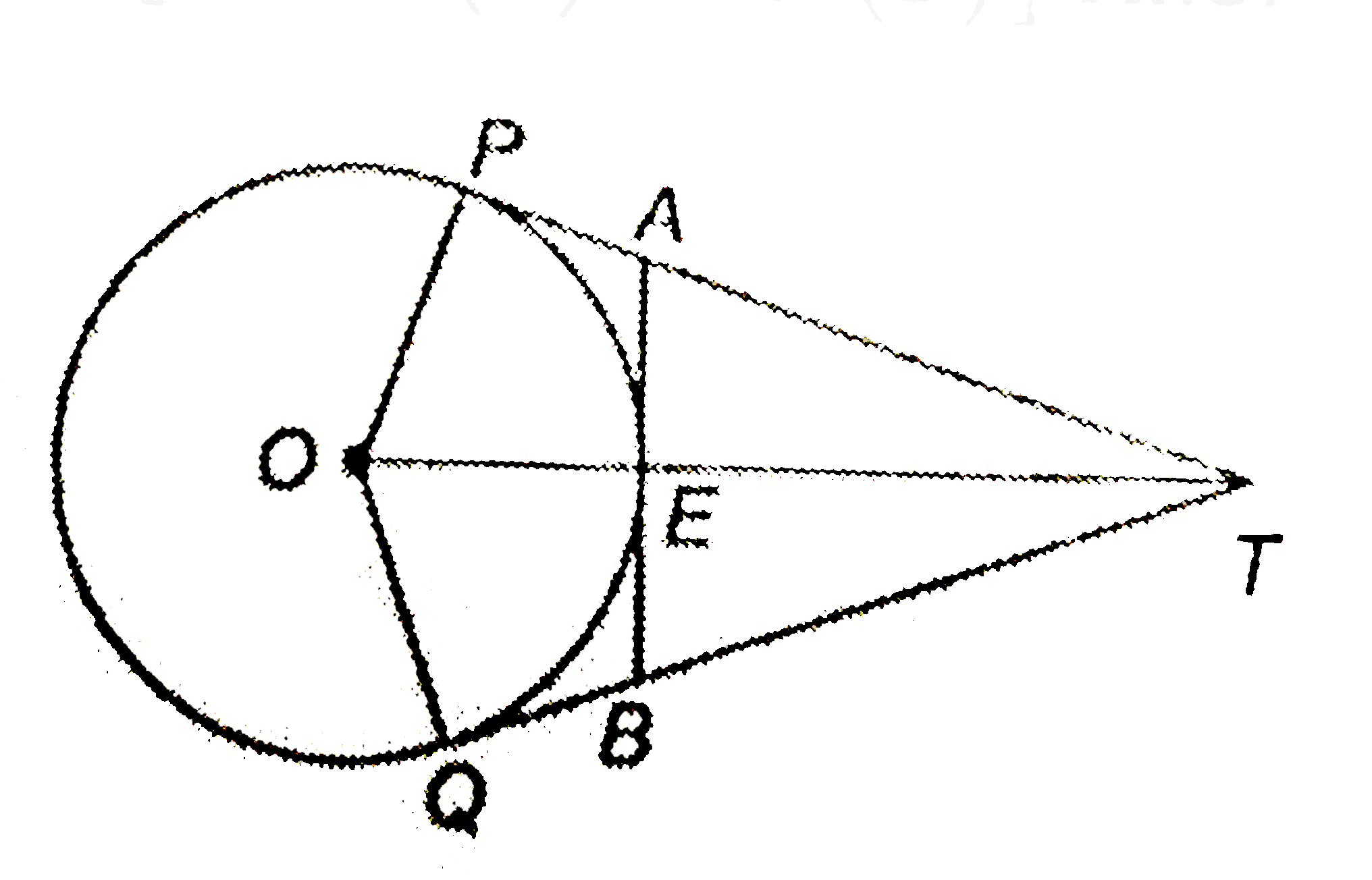
View SolutionO is the centre of a circle of radius 5cm. T is a point such that OT=13cm and OT intersects the circle at E, find the length AB.
 View Solution
View SolutionA tangent PQ at a point P of a circle of radius 5 cm meets a line through the centre O at a point Q such that OQ=12cm . Length PQ is
View SolutionA tangent PQ at a point P of a circle of radius 5 cm meets a line through the centre O at a point Q so that OQ=13cm . Find the length of PQ .
View SolutionA tangent PQ at a point P of a circle of radius 5 cm meets a line through the centre O at a point Q so that OQ=12cm. Length PQ is :
(A) 12 cm (B) 13 cm (C) 8.5 cm (D) √119cm.View SolutionO is the centre of a circle of radius 5cm. T is a point such that OT=13cm and OT intersects the circle at E. If AB is the tangent to the circle at E, find length of AB.
View SolutionTake a point O on the plane of the paper. With O as centre draw a circle of radius 3 cm. Take a point P on this circle and draw a tangent at P .
View SolutionA is a point at a distance 13 cm from the centre O of a circle of radius 5 cm. AP and AQ are the tangents to the circle at P and Q. If a tangent BC is drawn at a point R lying on the minor arc PQ to intersect AP at B and AQ at C, find the perimeter of the ΔABC
View Solution

