Two concentric circles are of radii 5 cm and 3 cm. Find the length of the chord of the larger circle which touches the smaller circle.
To find the length of the chord of the larger circle that touches the smaller circle, we can follow these steps:
1. Identify the Radii of the Circles:
- The radius of the larger circle (R) = 5 cm
- The radius of the smaller circle (r) = 3 cm
2. Draw the Diagram:
- Draw two concentric circles with a common center O.
- Mark the radius of the larger circle as OP (5 cm) and the radius of the smaller circle as OQ (3 cm).
- Draw a chord PQ of the larger circle that touches the smaller circle at point R.
3. Understand the Geometry:
- The line segment OR (where R is the point of tangency) is perpendicular to the chord PQ.
- Therefore, OR is the radius of the smaller circle, which is 3 cm.
4. Use the Pythagorean Theorem:
- In triangle OPR, where OP is the radius of the larger circle, OR is the radius of the smaller circle, and PR is half the length of the chord PQ.
- According to the Pythagorean theorem:
OP2=OR2+PR2
- Substitute the known values:
52=32+PR2
5. Calculate PR:
- Calculate the squares:
25=9+PR2
- Rearranging gives:
PR2=25−9=16
- Taking the square root:
PR=√16=4 cm
6. Find the Length of Chord PQ:
- Since PR is half of PQ:
PQ=2×PR=2×4=8 cm
Final Answer:
The length of the chord of the larger circle that touches the smaller circle is 8 cm.
---
- The radius of the larger circle (R) = 5 cm
- The radius of the smaller circle (r) = 3 cm
- Draw two concentric circles with a common center O.
- Mark the radius of the larger circle as OP (5 cm) and the radius of the smaller circle as OQ (3 cm).
- Draw a chord PQ of the larger circle that touches the smaller circle at point R.
- The line segment OR (where R is the point of tangency) is perpendicular to the chord PQ.
- Therefore, OR is the radius of the smaller circle, which is 3 cm.
- In triangle OPR, where OP is the radius of the larger circle, OR is the radius of the smaller circle, and PR is half the length of the chord PQ.
- According to the Pythagorean theorem:
OP2=OR2+PR2
- Substitute the known values:
52=32+PR2
- Calculate the squares:
25=9+PR2
- Rearranging gives:
PR2=25−9=16
- Taking the square root:
PR=√16=4 cm
- Since PR is half of PQ:
PQ=2×PR=2×4=8 cm
The length of the chord of the larger circle that touches the smaller circle is 8 cm.
Topper's Solved these Questions
Similar Questions
Two concentric circles are of radii 5 cm. and 3 c. Find the length of the chord of the larger circle which touches the cmaller circle.
View SolutionTwo concentric circles are of radii 13 cm and 5 cm. Find the length of the chord of the larger circle which touches the inner circle.
View SolutionTwo concentric circles are of diameters 30cm and 18cm. Find the length of the chord of the larger circle which touches the smaller circle.
View SolutionIf the radii of two concentric circles are 4 cm and 5 cm, then find the length of each chord of one circle which is tangent to the other circle.
View SolutionIf radii of two concentric circles are 4 cm and 5 cm, then length of each chord of one circle which is tangent to the other circle, is
View SolutionTwo concentric circle of radii 3cm and 5cm are given. Find the chord BC which touches the inner circle at P.
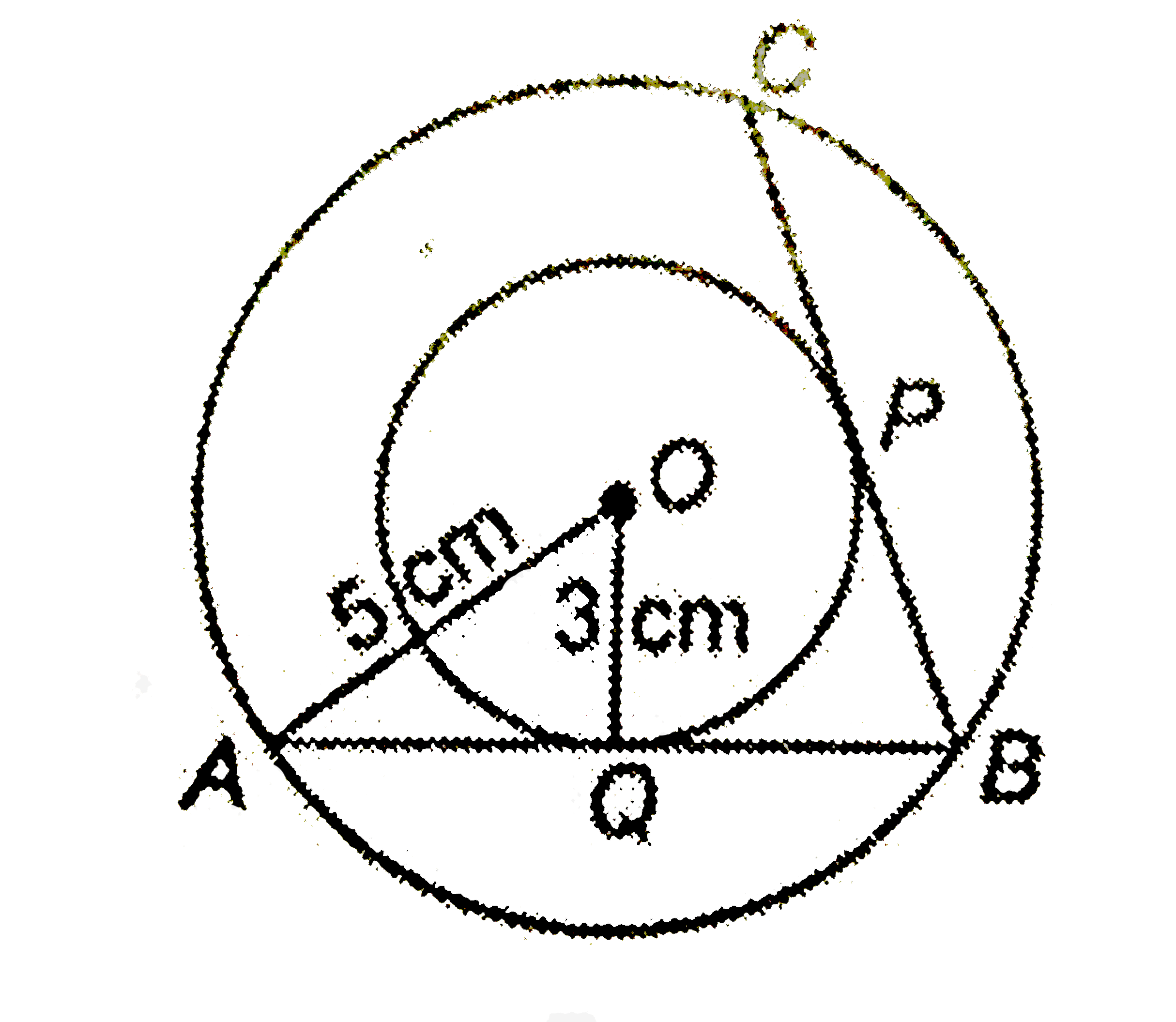
View SolutionA circle has radius 5cm. What is the length of its longest chord?
View SolutionTwo concentric circles of radii 3cmand5cm are given. Then length of chord BC which touches the inner circle at P is equal to (a) 4 cm (b) 6cm (c) 8 cm (d) 10 cm
View SolutionIf radii of the two concentric circles are 15cm and 17cm, then the length of each chord of one circle which is tangent to other is: 8cm (b) 16cm (c) 30cm (d) 17cm
View SolutionIf radii of the two concentric circles are 15cm and 17cm, then the length of each chord of one circle which is tangent to other is: 8cm (b) 16cm (c) 30cm (d) 17cm
View Solution