P is any point in the interior of a triangle ABC.
Prove that : PA+PB<AC+BC
Step by step video & image solution for P is any point in the interior of a triangle ABC. Prove that : PA+PB lt AC +BC by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
O is any point in the interior of a triangle ABC.
Prove that :
OB+OC<AB+AC.
 _Q01.png" width="60%">
_Q01.png" width="60%">
View SolutionLet O be any point in the interior of ΔABC, prove that :
AB+BC+CA<2(OA+OB+OC)
View SolutionP is any point inside the triangle ABC. Prove that : ∠BPC>∠BAC.
View SolutionIf P is any point in the interior of a parallelogram ABCD , then prove that area of the triangle APB is less than half the are of parallelogram.
View SolutionIf P is any point in the interior of a parallelogram ABCD, then prove that area of the triangle APB is less than half the area of parallelogram.
View SolutionIf D any point on the BC of an isosceles triangle ABC then prove that AB>AD and AC>AD.
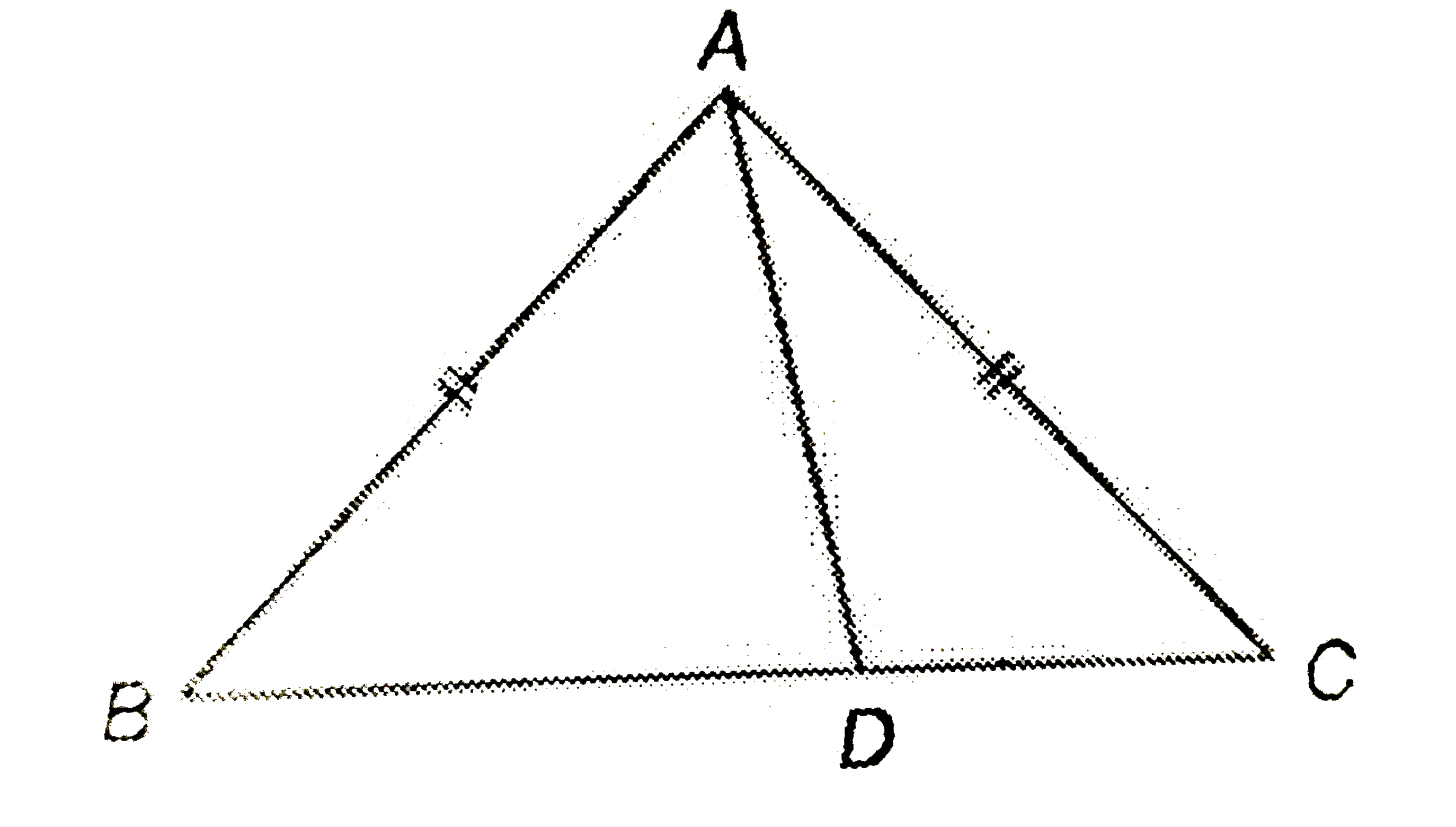
View SolutionAD is a median of triangle ABC. Prove that : AB+BC+AC>2AD
View SolutionD is a point in side BC of triangle ABC. If AD>AC, show that AB>AC.
View SolutionIn the adjoining figure, P is an interior point of ΔABC. Then prove that :
AB+BC+CA<2(PA+PB+PC)

View SolutionIn Figure, PQR is a triangle and S is any point in its interior, show that SQ+SR<PQ+PR. Given : S is any point in the interior of PQR To Prove : SQ+SR<PQ+PR Construction: Produce QS to meet PR in T
View Solution
