Prove that the circle drawn on any one of the equal sides of an isosceles triangle as diameter bisects the base.
Video Solution
Text Solution
Given : in which and a circle is drawn with as diameter, intersecting at .
To Prove :
Construction : Join .
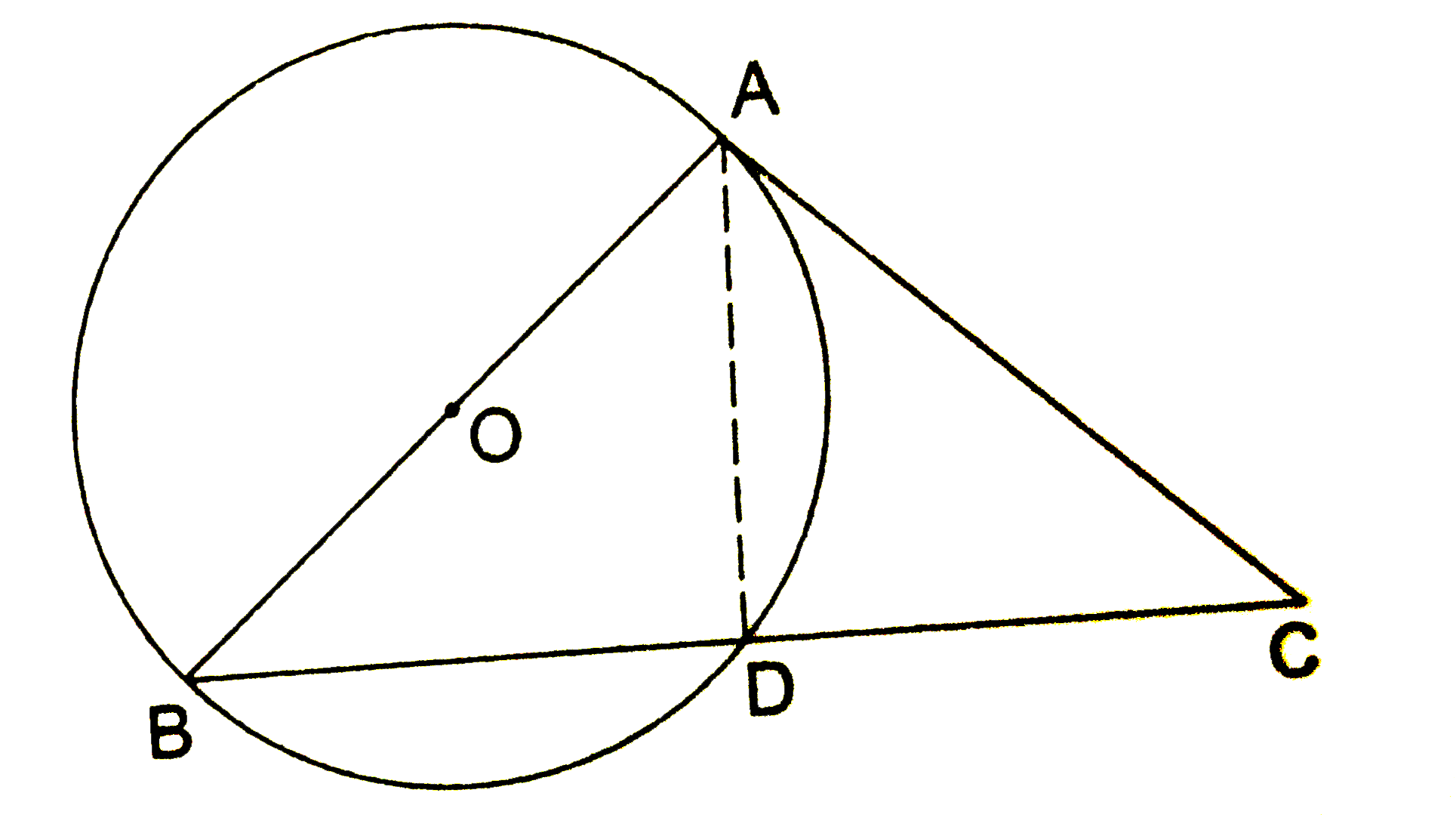
Proof : We known that an angle in a semicircle is a right angle.
....(i)
Also, DBC being a straight line, we have
[ using (i) ]
....(i)
Now, in ADB and , we have
AB AC [given]
[each equal to
[common ]
[ by - congurence]
Hence ,
|
Similar Questions
Knowledge Check
Each of the equal sides of an isosceles triangle is 13 cm and its base is 24 cm. The area of the triangle is
A156 cm2B78 cm2C60 cm2D120 cm2Each of equal sides of an isosceles triangle is 13 cm. If the length of base is 24 cm then its area is
A50cm2B60cm2C55cm2D65cm2
Prove that the perpendiculars drawn from the vertices of equal angles of an isosceles triangle to the opposite sides are equal.
View SolutionProve using vectors: The median to the base of an isosceles triangle is perpendicular to the base.
View SolutionProve that the bisector of the vertical angle of an isosceles triangle bisects the base at right angles.
View SolutionFind the area of an isosceles triangle having the base x cm and one side y cm
View SolutionThe line drawn from the midpoint of one side of a triangle parallel to another side bisects the third side.
View SolutionUsing Theorem 6.1, prove that a line drawn through the mid-point of one side of a triangle parallel to another side bisects the third side. (Recall that you have proved it in Class IX).
View Solution
