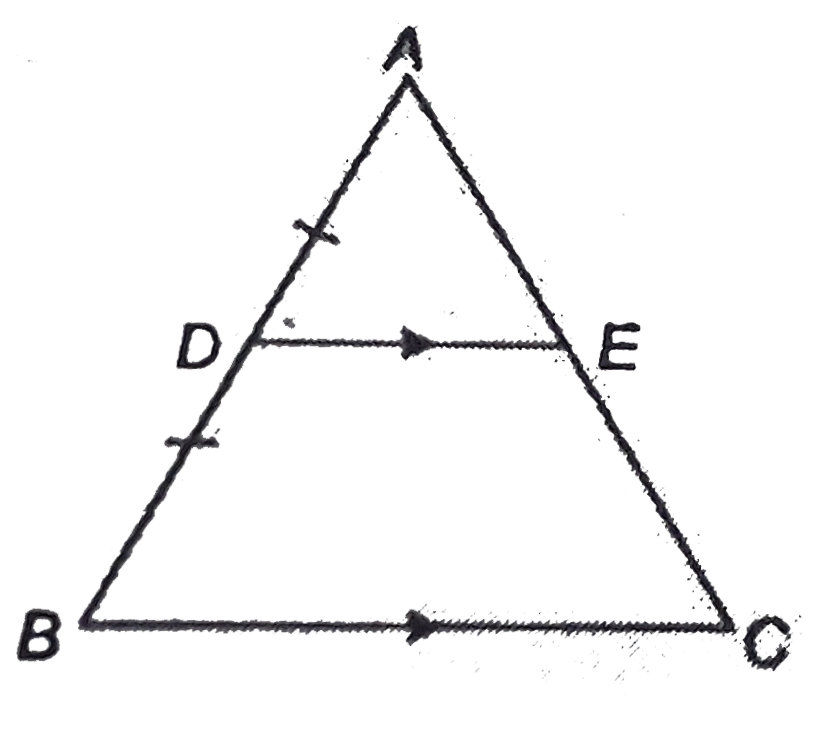
The line drawn from the midpoint of one side of a triangle parallel to another side bisects the third side.
To solve the problem, we need to prove that if a line is drawn from the midpoint of one side of a triangle parallel to another side, it bisects the third side. Let's denote the triangle as △ABC where AB is one side, AC is another side, and BC is the third side.
1. Identify the Triangle and Midpoint:
Let D be the midpoint of side AB.
Hint: Remember that the midpoint divides the segment into two equal parts.
2. Draw a Line Parallel to Another Side:
Draw a line DE from point D that is parallel to side AC.
Hint: Use the properties of parallel lines which state that corresponding angles are equal.
3. Use the Basic Proportionality Theorem (Thales' Theorem):
According to Thales' theorem, if a line is drawn parallel to one side of a triangle, it divides the other two sides proportionally. Therefore, since DE is parallel to AC, we have:
BDDC=ABAC
Hint: This theorem is crucial for establishing the proportionality of segments in triangles.
4. Establishing the Ratio:
Since D is the midpoint of AB, we can say that BD=DA. Thus, we can denote BD=DA=x. Therefore, AB=2x.
Hint: Always express lengths in terms of a variable to simplify calculations.
5. Apply the Proportionality:
Now, substituting into the ratio we have:
xDC=2xAC
Cross-multiplying gives:
x⋅AC=2x⋅DC
Hint: Cross-multiplication is a useful technique for solving proportions.
6. Simplifying the Equation:
Dividing both sides by x (assuming x≠0):
AC=2⋅DC
This implies that DC is half of AC.
Hint: Simplifying equations helps in reaching conclusions more easily.
7. Conclusion:
Since D is the midpoint of AB and DE is parallel to AC, it follows that DE bisects BC at point E. Therefore, E is the midpoint of BC.
Hint: The conclusion ties back to the original statement of the problem, confirming the relationship.
Final Statement:
Thus, we have proved that the line drawn from the midpoint of one side of a triangle parallel to another side bisects the third side.
Let D be the midpoint of side AB.
Draw a line DE from point D that is parallel to side AC.
According to Thales' theorem, if a line is drawn parallel to one side of a triangle, it divides the other two sides proportionally. Therefore, since DE is parallel to AC, we have:
BDDC=ABAC
Since D is the midpoint of AB, we can say that BD=DA. Thus, we can denote BD=DA=x. Therefore, AB=2x.
Now, substituting into the ratio we have:
xDC=2xAC
Cross-multiplying gives:
x⋅AC=2x⋅DC
Dividing both sides by x (assuming x≠0):
AC=2⋅DC
This implies that DC is half of AC.
Since D is the midpoint of AB and DE is parallel to AC, it follows that DE bisects BC at point E. Therefore, E is the midpoint of BC.
Thus, we have proved that the line drawn from the midpoint of one side of a triangle parallel to another side bisects the third side.
Similar Questions
Using Theorem 6.1, prove that a line drawn through the mid-point of one side of a triangle parallel to another side bisects the third side. (Recall that you have proved it in Class IX).
View SolutionProve using similar triangles, that a line drawn through the mid-point of one side of a triangle parallel to another side, bisects the third side.
View Solution
Knowledge Check
The line segment joining the midpoints of any two sides of a triangle is parallel to the third side and equal to half of it.
In the given figure, the side AC of ΔABC is produced to E such that CE = FAC. If D is the midpoint of BC and ED produced meets AB at F and CP, DQ are drawn parallel to BA, then FD=
 A12FEB13EFC2FEDFE
A12FEB13EFC2FEDFE
Theorem 8.10 : The line drawn through the mid-point of one side of a triangle, parallel to another side bisects the third side.
View SolutionThe line drawn through the mid-point of one side of a triangle, parallel to another side, intersects the third side at its mid-point.
View SolutionThe line drawn through the mid-point of one side of a triangle is parallel to another side and intersects the third side at its mid-point.
View SolutionThe line joining the mid-points of two sides of a triangle is parallel to the third side.
View Solutionprove that the line segment joining the mid-points of two sides of a triangle is parallel to the third side.
View SolutionProve that the line segment joining the mid points of two sides of a triangle is parallel to its third side.
View SolutionProve that the line joining the middle points of the two sides of a triangle is parallel to the third side.
View Solution
