Shade the regions where points satisfy |x−y|<1. Solution in Bengali
|x−y|<1
∴ −1<x−y<1 (i)
Points satisfying (i) lying the region common to two regions satisfied by the inequalities x−y>−1andx−y<1.
For x−y+1>0, the half region is one in which (0,0) lies as this point satisfies x−y+1>0.
For x−y−1<0, the half region is one in which (0,0) lies as this point satisfies x−y−1<0.
Hence the common region is as shown in the following figure.
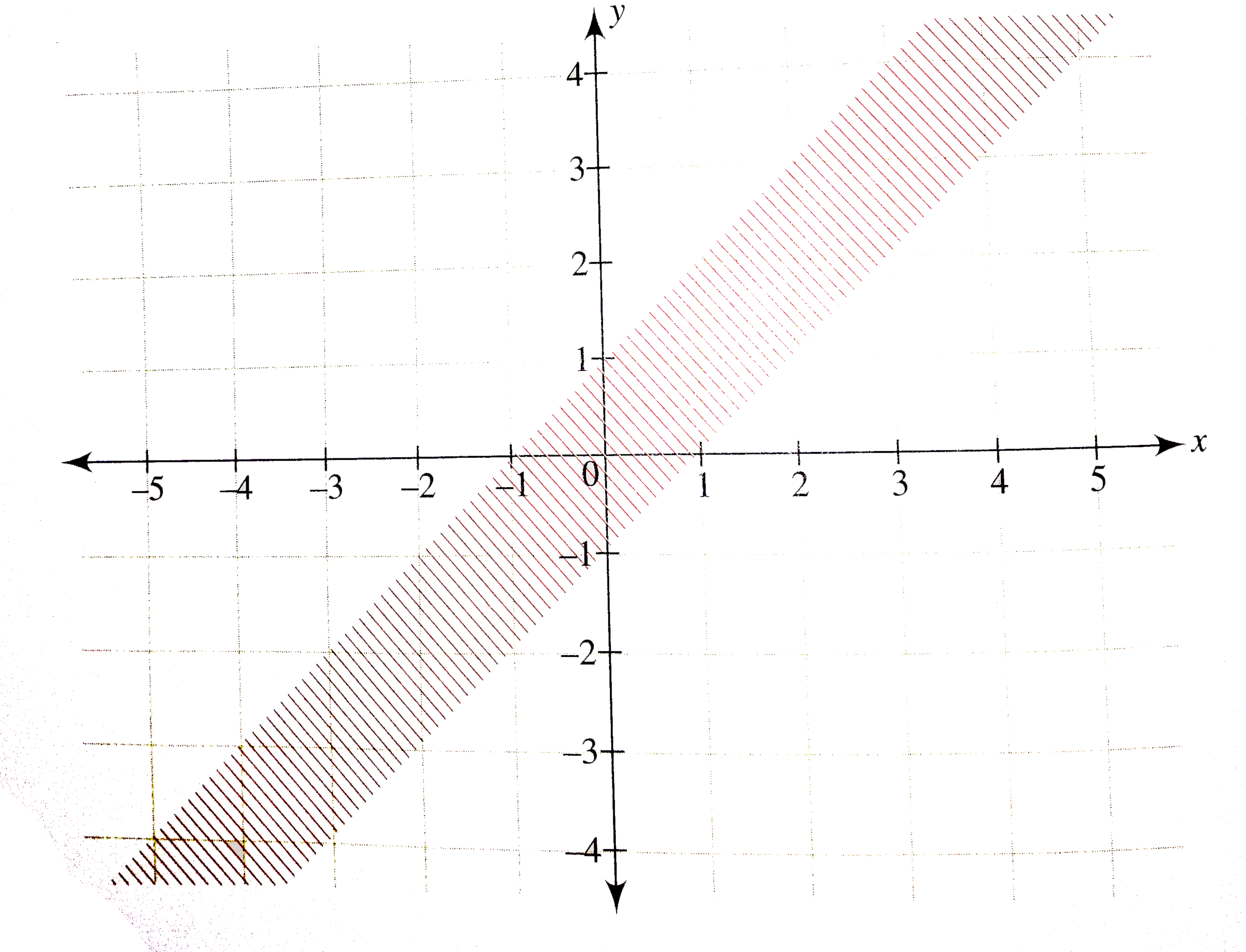
Topper's Solved these Questions
- GRAPHS OF ELEMENTARY FUNCTIONS BOOK - CENGAGE PUBLICATIONCHAPTER - GRAPHS OF ELEMENTARY FUNCTIONS EXERCISE - EXERCISES
34 Videos
- GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSBOOK - CENGAGE PUBLICATIONCHAPTER - GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSEXERCISE - Exercises
17 Videos
- GRAPHS OF TRIGONOMETRIC FUNCTIONSBOOK - CENGAGE PUBLICATIONCHAPTER - GRAPHS OF TRIGONOMETRIC FUNCTIONSEXERCISE - Exercises
22 Videos
Similar Questions
Draw the graph of y=1(1/x).
View SolutionDraw the graph of y=(x−1)(x2−x+1).
View SolutionDraw the graph of y= cosec 2x−cot2x. Is f(x) periodic ? If yes, what is its fundamental period ?
View SolutionShade the regions where points satisfy |x−y|<1.
View SolutionSketch the region satisfying |x|<|y|.
View SolutionPlot the region of the points P (x,y) satisfying |x|+|y|<1.
View SolutionThe area (in sq. units ) of the region A={(x,y):(x−1)[x]≤y≤2√x,0≤x≤2] where [t] denotes the greatest integer function is
View SolutionThe set of point (x,y) satisfying x+y ≤1,−x−y≤1 lie in the region bounded by the two straight lines passing through the respective pair of points.
View SolutionShade the regions where points satisfy |x−y|<1.
View SolutionSketch the region satisfying |x|<|y|.
View SolutionPlot the region of the points P (x,y) satisfying |x|+|y|<1.
View SolutionThe area of the region described by A={(x,y):x2+y2≤1andy2≤1−x} is
View SolutionShade the region given by inequality
x+y<5
View SolutionShade the regions where points satisfy |x-y| lt 1 .
View SolutionSketch the region satisfying |x| lt |y| .
View Solution
