Show that if the diagonals of a quadrilateral bisect each other at right angles, then it is a rhombus.

Video Solution

Text Solution

Verified by Experts

Given : A quadrialteral ABCD whose diagonals AC and BD bisect each other at right angles.
$i . e ., O A = O C and O B = O D$
$and ∠ A O D = ∠ A O B = ∠ C O D = ∠ B O C = 90^{\circ}$

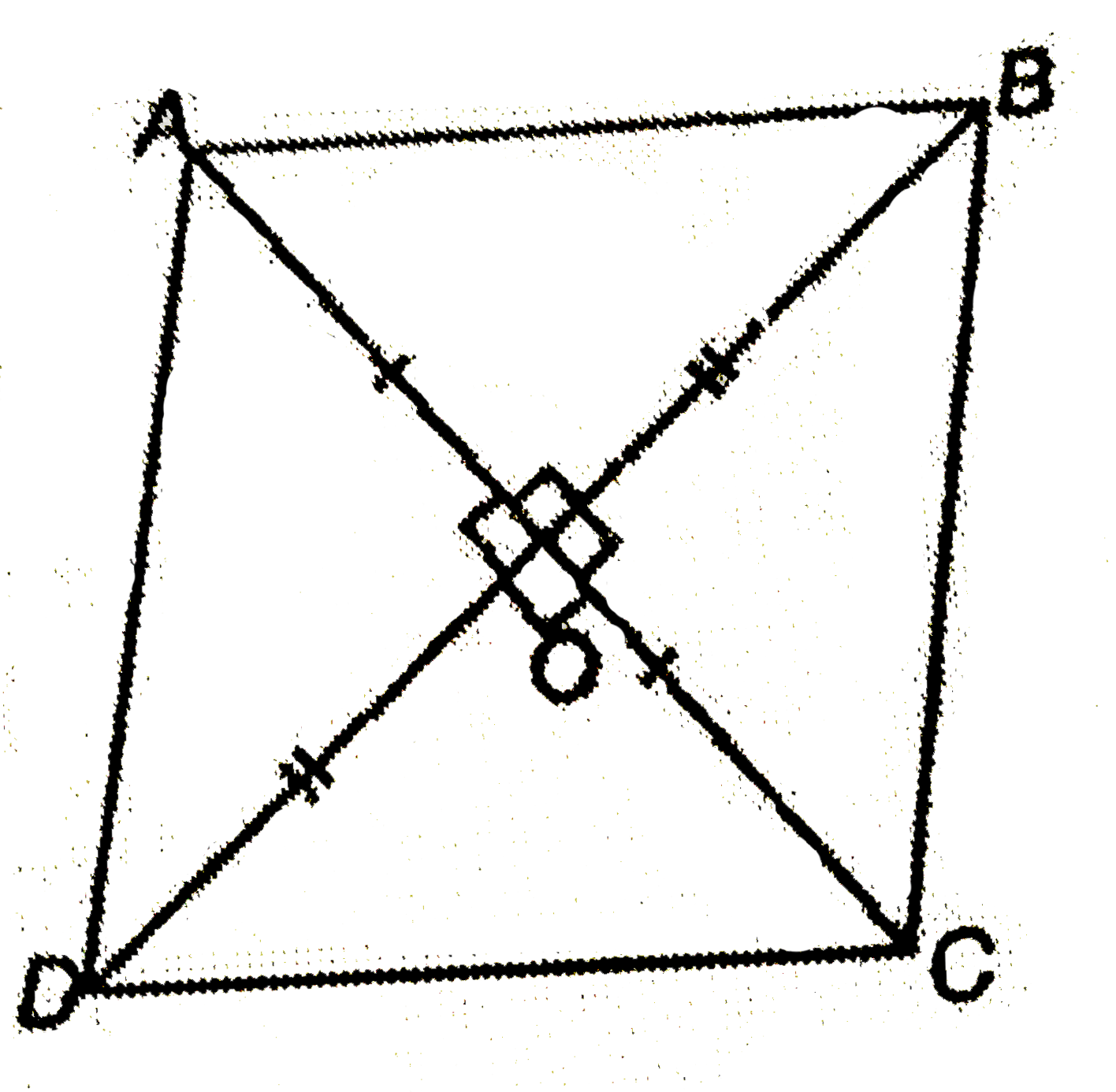
To prove : ABCD is a rhombus.
Proof. In $Δ O A B and Δ O D C,$ we have
$∵ ⎧ ⎪ ⎨ ⎪ ⎩ \begin{matrix} O A = O C & (given) O B = O D & (given) ∠ A O B = ∠ C O D & (vertically opposite angles) \end{matrix}$
$∴ Δ O A B ≅ Δ O C D (by SAS rule)$
$∴ A B = C D (c.p.c.t) ... (1)$
$∵ ⎧ ⎪ ⎨ ⎪ ⎩ \begin{matrix} O A = O C & (given) O D = O B & (given) ∠ A O D = ∠ B O C & (vertically oposite angles) \end{matrix}$
$∴ Δ O A D ≅ Δ O C B (by SAS rule)$
$∴ A D = B C (c . p . c . t .) ... (2)$
Similarly, we can prove that
$\begin{matrix} A B = A D C D = B C \end{matrix} ... (3)$
Hence, from (1), (2) and (3), we get
$A B = B C = A D = C D$
Hence, ABCD is a rhombus.

Updated on:21/07/2023