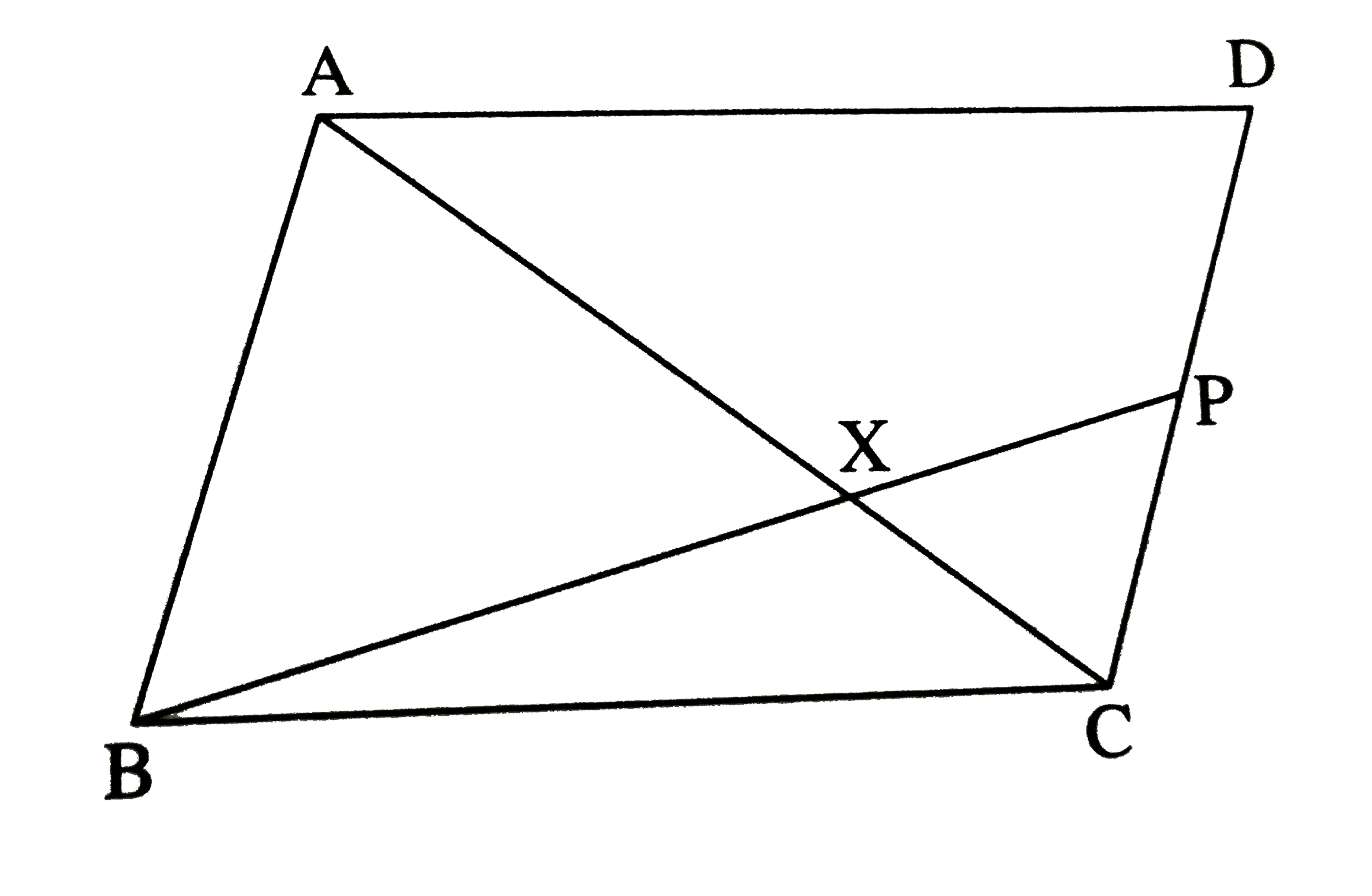
□ABCD is a parallelogram. P is any point on side BC. Find two pairs of triangles with equal areas.
The correct Answer is:i. A(ΔABC)=A(ΔBDC)
ii. A(ΔABC)=A(ΔABD)
Step by step video & image solution for squareABCD is a parallelogram. P is any point on side BC. Find two pairs of triangles with equal areas. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SIMILARITY BOOK - TARGET PUBLICATIONCHAPTER - SIMILARITY EXERCISE - Additonal Problems for Practice(Based on Practice Set 1.2)
11 Videos
- SIMILARITY BOOK - TARGET PUBLICATIONCHAPTER - SIMILARITY EXERCISE - Additonal Problems for Practice(Based on Practice Set 1.3)
7 Videos
- SIMILARITY BOOK - TARGET PUBLICATIONCHAPTER - SIMILARITY EXERCISE - Multiple Choice Questions
28 Videos
- QUESTION FROM STD. IXBOOK - TARGET PUBLICATIONCHAPTER - QUESTION FROM STD. IXEXERCISE - Statistics
14 Videos
- STATISTICSBOOK - TARGET PUBLICATIONCHAPTER - STATISTICSEXERCISE - Problem Set-6
21 Videos
Similar Questions
Knowledge Check
ABCD is a parallelogram, E and F are the mid-points of BC and CD. Find the ratio of area of parallelogram ABCD and ΔAEF
A2:1B4:1C8:3D8:1ABCD is a parallelogram and m ∠DAB=30∘, BC=20 cm and AB=20 cm. Find the area of parallelogram:
A150cm2B200cm2C400cm2D260cm2The area of a parallelogram PQRS is 36cm2. M is any point on the side RS. The area of ΔPMQ is.
A18cm2B9cm2C36cm2D12cm2
In the adjoining figure, ABCD is a parallelogram and P is any points on BC. Prove that
ar(△ABP)+ar(△DPC)=ar(△PDA).
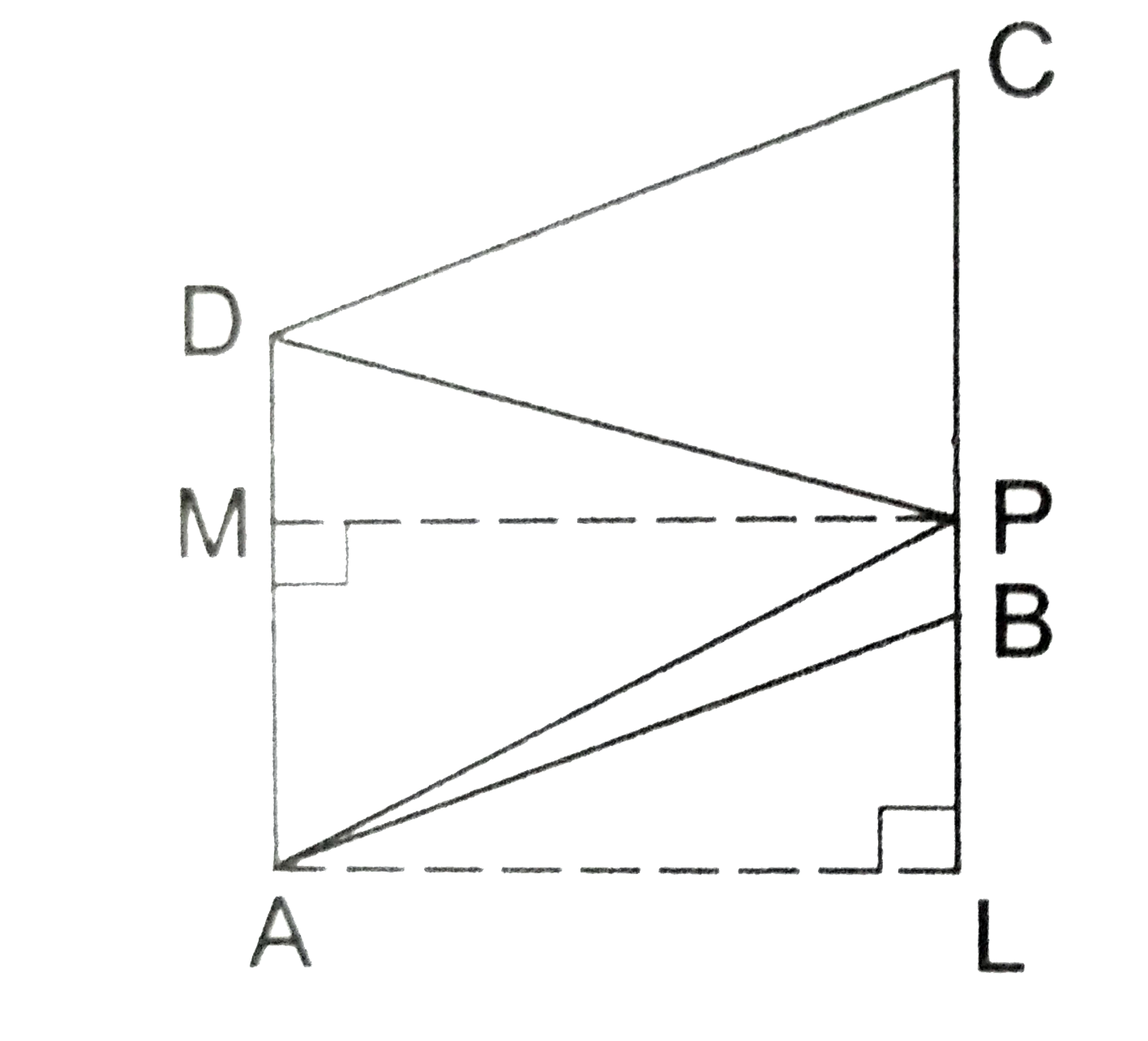
View SolutionABCD is a parallelogram. P and Q are mid points of BC & CD. Find the area of ΔAPQ if area of ΔABC is 12.
View SolutionA diagonal of a parallelogram divides it into two triangles of equal area.
View SolutionABCD is a parallelogram. E is a point on BC such that BE : EC = m:n. If AE and DB intersect in F, then what is the ratio of the area of triangle FEB to the area of triangle AFD?
View SolutionABCD is a parallelogram. E is a point on extended side AB such that AB = BE. By joining D to E it intersects side BC at P. Find length of PC if BC = 15 cm.
View Solution