State whether the statements are true (T) or false (F).
The area of a trapezium become 4 times if its height gets doubled.
To determine whether the statement "The area of a trapezium becomes 4 times if its height gets doubled" is true or false, we can analyze the formula for the area of a trapezium.
1. Understand the Formula for the Area of a Trapezium:
The area A of a trapezium can be calculated using the formula:
A=12×(a+b)×h
where a and b are the lengths of the two parallel sides, and h is the height.
2. Consider Doubling the Height:
If we double the height h, the new height becomes 2h. We can substitute this into the area formula:
A′=12×(a+b)×(2h)
3. Simplify the New Area:
Now, simplify the expression for the new area A′:
A′=12×(a+b)×2h=(a+b)×h
4. Relate the New Area to the Original Area:
The original area A was:
A=12×(a+b)×h
Now, we can see that:
A′=2×A
This means that when the height is doubled, the area becomes twice the original area, not four times.
5. Conclusion:
Therefore, the statement "The area of a trapezium becomes 4 times if its height gets doubled" is False.
Final Answer:
False (F)
The area A of a trapezium can be calculated using the formula:
A=12×(a+b)×h
where a and b are the lengths of the two parallel sides, and h is the height.
If we double the height h, the new height becomes 2h. We can substitute this into the area formula:
A′=12×(a+b)×(2h)
Now, simplify the expression for the new area A′:
A′=12×(a+b)×2h=(a+b)×h
The original area A was:
A=12×(a+b)×h
Now, we can see that:
A′=2×A
This means that when the height is doubled, the area becomes twice the original area, not four times.
Therefore, the statement "The area of a trapezium becomes 4 times if its height gets doubled" is False.
False (F)
Topper's Solved these Questions
- MENSURATIONBOOK - NCERT EXEMPLARCHAPTER - MENSURATIONEXERCISE - APPLICATIONS, GAMES AND PUZZLES
13 Videos
- MENSURATIONBOOK - NCERT EXEMPLARCHAPTER - MENSURATIONEXERCISE - THINK AND DISCUSS
2 Videos
- MENSURATIONBOOK - NCERT EXEMPLARCHAPTER - MENSURATIONEXERCISE - THINK AND DISCUSS
2 Videos
- LINEAR EQUATIONS IN ONE VARIABLEBOOK - NCERT EXEMPLARCHAPTER - LINEAR EQUATIONS IN ONE VARIABLEEXERCISE - THINK AND DISCUSS
3 Videos
- PLAYING WITH NUMBERS BOOK - NCERT EXEMPLARCHAPTER - PLAYING WITH NUMBERS EXERCISE - Think and Discuss
1 Videos
Similar Questions
Knowledge Check
State whether the following statements are true or false :
AAn electric motor converts mechanical energy into electrical energy.BAn electric generator works on the principle of electromagnetic induction.CThe field at the centre of a long circular coil carrying current will be parallel straight lines.DA wire with a green insulation is usually the live wire of an electric supply.
state whether the statement are true (T) or false (F).
Every kite is a trapezium.
View SolutionState whether the statements are true or falsea×b=b×a
View SolutionState whether the statements are true or falsea÷a=b÷a
View SolutionState whether the statements are true (T) or (F) false.
Every square is a trapezium.
View SolutionState whether the statements are true (T) or false (F).
1L = 1000 cm3
View SolutionState whether the statements are true (T) or (F) false.
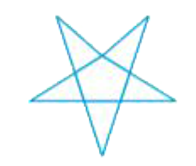 is a polygon.
is a polygon.
View SolutionState whether the statements are true or false−3×3=−12−3
View Solution
