The cable of a uniformly loaded suspension bridge hangs in the form of a parabola. The roadway which is horizontal and 100m long is supported by vertical wires attached to the cable, the longest wire being 30m and the shortest being 6m. Find the length of which is at 18m apart from the middle point of cable.
To solve the problem step by step, we need to analyze the given information about the suspension bridge and the parabolic shape of the cable.
Step 1: Understand the setup
The cable of the suspension bridge hangs in the shape of a parabola. The horizontal roadway is 100 m long, and the lengths of the vertical wires attached to the cable vary. The longest wire is 30 m, and the shortest wire is 6 m.
Step 2: Determine the coordinates of the points
- The midpoint of the cable (the vertex of the parabola) is at the origin (0, 0).
- The longest wire (30 m) is at the end of the roadway, which is 50 m from the center (half of 100 m). Thus, its coordinates are (50, 30).
- The shortest wire (6 m) is at the other end of the roadway, which is also 50 m from the center, giving it coordinates (50, 6).
Step 3: Find the coordinates of the point on the parabola
The difference in height between the longest and shortest wires is:
30−6=24 m
Thus, the height of the cable at the midpoint (50 m from the origin) is 24 m. Therefore, the coordinates of this point on the parabola are (50, 24).
Step 4: Write the equation of the parabola
The general form of a parabola that opens upwards is given by:
x2=4ay
Since the point (50, 24) lies on this parabola, we can substitute these values into the equation:
502=4a⋅24
Calculating gives:
2500=96a
From this, we can solve for a:
a=250096
Step 5: Find the height of the cable at a distance of 18 m from the center
Now we need to find the height of the cable at a point that is 18 m away from the center. The x-coordinate for this point is:
x=18
We substitute x=18 into the parabola equation:
182=4a⋅y1
Substituting a:
324=4⋅250096⋅y1
Simplifying gives:
324=1000096⋅y1
Now, solving for y1:
y1=324⋅9610000
Calculating y1:
y1=3110410000=3.1104 m
Step 6: Calculate the total length of the wire
The total length of the wire at this point is the sum of the shortest wire (6 m) and the height of the cable at that point (3.1104 m):
Total length=6+3.1104=9.1104 m
Final Answer
The length of the wire that is 18 m apart from the middle point of the cable is approximately 9.11 m.
The cable of the suspension bridge hangs in the shape of a parabola. The horizontal roadway is 100 m long, and the lengths of the vertical wires attached to the cable vary. The longest wire is 30 m, and the shortest wire is 6 m.
- The midpoint of the cable (the vertex of the parabola) is at the origin (0, 0).
- The longest wire (30 m) is at the end of the roadway, which is 50 m from the center (half of 100 m). Thus, its coordinates are (50, 30).
- The shortest wire (6 m) is at the other end of the roadway, which is also 50 m from the center, giving it coordinates (50, 6).
The difference in height between the longest and shortest wires is:
30−6=24 m
Thus, the height of the cable at the midpoint (50 m from the origin) is 24 m. Therefore, the coordinates of this point on the parabola are (50, 24).
The general form of a parabola that opens upwards is given by:
x2=4ay
Since the point (50, 24) lies on this parabola, we can substitute these values into the equation:
502=4a⋅24
Calculating gives:
2500=96a
From this, we can solve for a:
a=250096
Now we need to find the height of the cable at a point that is 18 m away from the center. The x-coordinate for this point is:
x=18
We substitute x=18 into the parabola equation:
182=4a⋅y1
Substituting a:
324=4⋅250096⋅y1
Simplifying gives:
324=1000096⋅y1
Now, solving for y1:
y1=324⋅9610000
Calculating y1:
y1=3110410000=3.1104 m
The total length of the wire at this point is the sum of the shortest wire (6 m) and the height of the cable at that point (3.1104 m):
Total length=6+3.1104=9.1104 m
The length of the wire that is 18 m apart from the middle point of the cable is approximately 9.11 m.
Topper's Solved these Questions
Similar Questions
The cable of a uniformly loaded suspension bridge hangs in the form of a parabola. The roadway which is horizontal and 100m long is supported by vertical wires attached to the cable, the longest wire being 30m and the shortest being 6m. Find the length of the supporting wire attached to the roadway 18m from the middle.
View Solutionover the towers of a bridge a cable is hung in the form of a parabola, have their tops 30 meters above the road way are 200 meters apart. If the cable is 5 meters above the road way at the centre of the bridge, then the length of the vertical supporting cable 30 meters from the centre is
View SolutionIf the distribution of weight is uniform, then the rope of the suspended bridge takes the form of parabola.The height of the supporting towers is 20m, the distance between these towers is 150m and the height of the lowest point of the rope from the road is 3m. Find the equation of the parabolic shape of the rope considering the floor of the parabolic shape of the rope considering the floor of the bridge as X-axis and the axis of the parabola as Y-axis. Find the height of that tower which supports the rope and is at a distance of 30 m from the centre of the road.
View SolutionA lift of mass m is supported by a cable that can with stand a force of 3mg. Find the shortest distance in which the lift can be stopped when it is descending with a speed of g/4.
View SolutionA metal wire of length L is suspended vertically from a rigid support. When a bob of mass M is attached to the lower end of wire, the elongation of the wire is l:
View SolutionA guy attached wire to a vertical pole of height 18m is 24 m long and has a stake attached to the other end. How far from the base of the pole should the stake be driven so that the wire will be taut?
View Solution1m long wire is folded in form of a circular coil and 100 mA electric current is flowing in it, then magnetic field at a point 1m away from its centre on its axis
View SolutionA potentiometer wire is 5 m long and a potential difference of 6V is maintained between its ends. Find the emf of a cell which balance against a length of 180 cm of the potentiometer wire.
View SolutionA field is in the form of a rectangle of length 18m and width 15m. A pit, 7.5m long, 6m broad and 0.8m deep, is dug in a corner of the field and the earth taken out is spread over the remaining area of the field. Find cut the extent to which the level of the field has been raised.
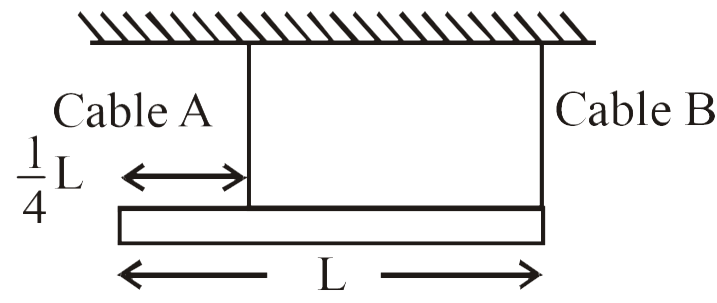
View SolutionA uniform bar of mass M and length L is horizontally suspended from the ceiling by two vertical light cables as shown. Cable A is connected 14th distance from the left end of the bar. Cable B is attached at the far right end of the bar. What is the tension in cable A?
 View Solution
View Solution

