The diagonals AC and BD of a rhombus intersect at (5,6). If A≡(3,2), then find the equation of diagonal BD.Solution in Tamil
The correct Answer is:2y+x-17=0
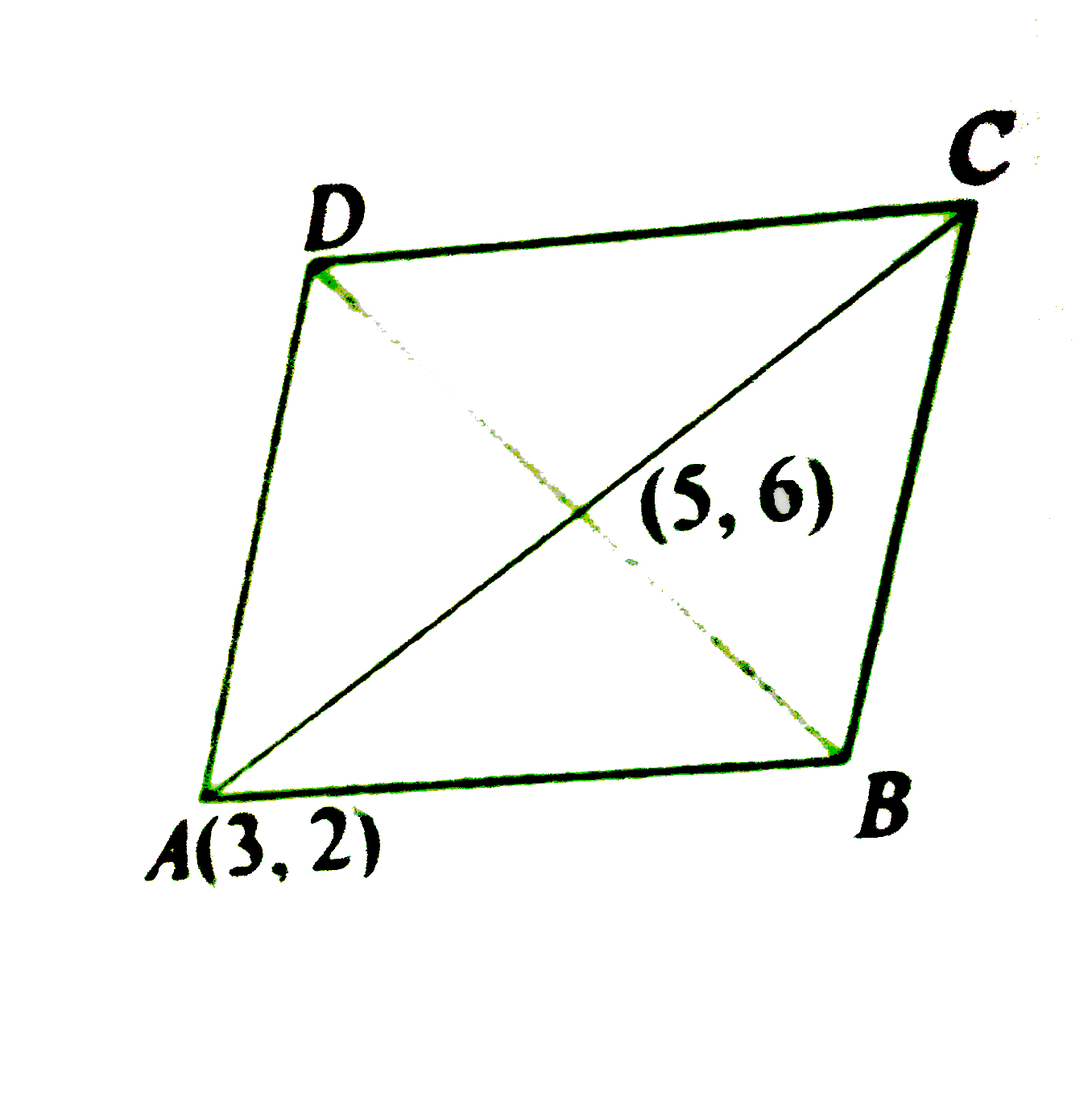
mAC=6−25−3=2
or mBD=−12
Thus, the equation of BD is
(y−6)=−12(x−5)
i.e., 2y+x-17=0
Topper's Solved these Questions
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise 2.2
4 Videos
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise 2.3
7 Videos
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - JEE Advanced Previous Year
4 Videos
- STRAIGHT LINEBOOK - CENGAGECHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type
8 Videos
- THEORY OF EQUATIONSBOOK - CENGAGECHAPTER - THEORY OF EQUATIONSEXERCISE - JEE Advanced Previous Year
9 Videos
Similar Questions
Find the points on the line x + y = 1 that lie at a distance 3 units from the line 5x+12y = 3.
View SolutionFind the points on y−aξs whose perpendicular distance from the line 4x−3y−12=0 is 3.
View Solutionabout to only mathematics
View SolutionThe point P(α,α+1) will lie inside the triangle whose vertices are A(0,3),B(−2,0) and C(6,1) if
View SolutionQ. Let p and q real number such that p≠0,p2≠q and p2≠−q. if α and β are non-zero complex number satisfying α+β=−p and α3+β3=q, then a quadratic equation having αβ and βα as its roots is
View SolutionThe diagonals AC and BD of a rhombus intersect at (5,6). If A≡(3,2), then find the equation of diagonal BD.
View SolutionThe diagonals AC and BD of a rhombus intersect at (5,6). If A(3,2), then find the equation of diagonal BD.
View SolutionDiagonals AC and BD of a quadrilateral ABCD intersect at O in such a way that ar (AOD)=ar ( BOC). Prove that ABCD is a trapezium.
View SolutionABCD is a rhombus whose diagonals intersect at O. If AB=10cm, diagonals BD=16 cm , find the length of diagonal AC
View SolutionABCD is a rhombus. Show that diagonal AC bisects A as well as C and diagonal BD bisects B as well as D.
View SolutionThe diagonals AC and BD of a rhombus intersect at (5,6). If A=(3,2), then find the equation of diagonal BD.
View SolutionThe diagonals AC and BD of a rhombus intersect at (5,6). If A≡(3,2), then find the equation of diagonal BD.
View SolutionThe diagonals of a rectangle ABCD meet at O. If ∠BOC=440, find ∠OAD.
View SolutionShow that the area of a rhombus is half the product of the lengths of its diagonals. GIVEN : A rhombus ABCD whose diagonals AC and BD intersect at O. TO PROVE : ar(rhombusABCD)=12(AC⋅BD)
View SolutionDiagonals AC and BD of a quadrilateral ABCD intersect at O in such a way that ar (AOD)=ar ( BOC). Prove that ABCD is a trapezium.
View SolutionShow that the area of a rhombus is half the product of the lengths of its diagonals. GIVEN : A rhombus ABCD whose diagonals AC and BD intersect at O. TO PROVE : ar(rhombusABCD)=12(ACxBD)
View Solution
