The distribution given below show the marks of 100 students of a class.
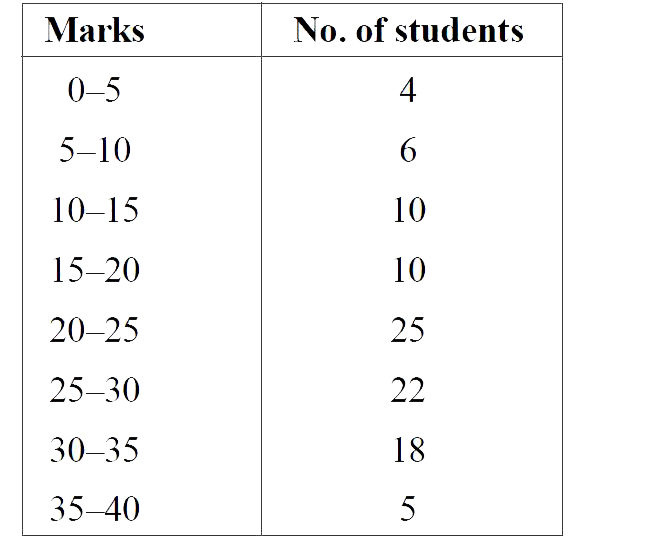
Draw a less than type and a more than type ogive from the given data. Hence obtain the median marks from the graph.
The correct Answer is:Median = 24
Topper's Solved these Questions
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - STATISTICSEXERCISE - Practice -Test5videos
STATISTICS
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - STATISTICSEXERCISE - Short Answer Type Question (II)8videos
STATISTICS
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - SOME APPLICATIONS OF TRIGONOMETRYEXERCISE - PRACTICE-TEST (SECTION-D)1videos
SOME APPLICATIONS OF TRIGONOMETRY
- BOOK - CBSE COMPLEMENTARY MATERIALCHAPTER - SURFACE AREA AND VOLUME EXERCISE - PRACTICE TEST ( SECTION-D)1videos
SURFACE AREA AND VOLUME
Similar Questions
During the medical checkup of 35 students of a class their weight were recorded as follows :
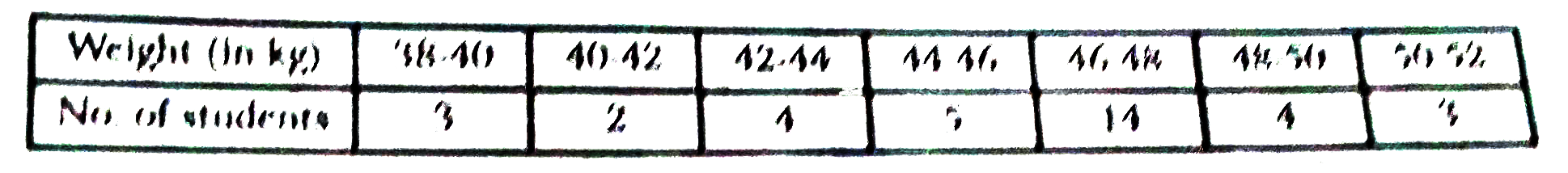
Draw a less than type a more than type ogive from the given data. Hene obtain the median weigth from the graph.View SolutionDraw 'less than' and 'more than' ogive curves from the following data :
 View Solution
View SolutionConstruct a less than ogive and a more than ogive from the following data :
 View Solution
View SolutionConstruct a less than ogive and a more than ogive from the following data :
 View Solution
View SolutionDuring the medical check up of 35 students of a class, their weights were recorded as follows :
Draw a less then type ogive for the given data. Hence obtain the median weight from the graph and verify the result by using the formula.
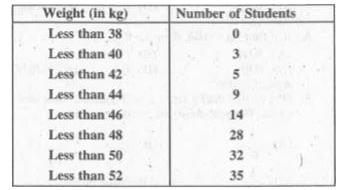 View Solution
View SolutionDraw a more than ogive from the given data and estimate median with the help of ogive. :
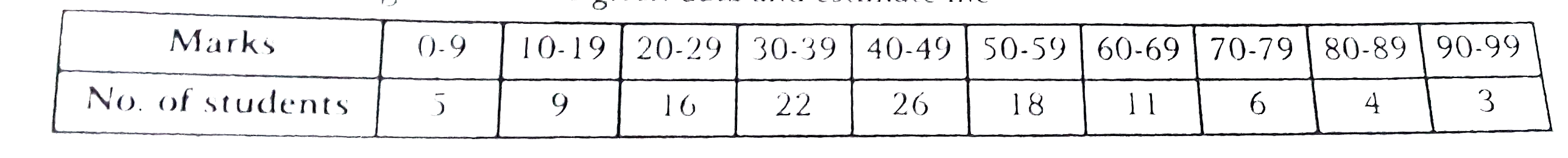 View Solution
View SolutionDuring the medical check-up of 35 students of a class, their weights were recorded as follows:Draw a less than type ogive for the given data. Hence obtain the median weight from the graph and verify the result by using the formula.
View SolutionDraw less than and more than ogive on the same graph and estimate median :
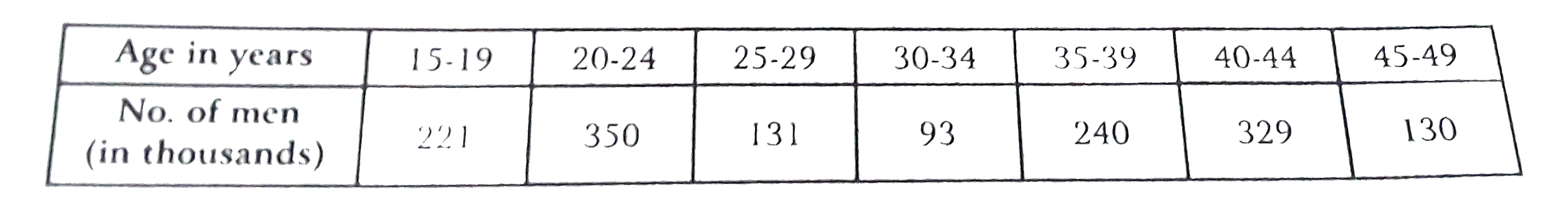 View Solution
View SolutionThe distribution given below, shows the marks obtained by 25 students in an aptitude test. Find the median and mode of the distribution.
View SolutionDraw a less than and more than median weight from the graph and type ogive for the given data. Hence find the verify the result by using the formula.
View Solution

