The eccentricity of the ellipse 9x2+25y2−18x−100y−116=0 is 25/16 b. 4/5 c. 16/25 d. 5/4
Video Solution
Text Solution
We have 9x2+25y2−18x−100y−116=0.
We can rewrite this as (x−)225+(y−2)29=1.
Which is an ellipse having centre at (1, 2), foci at (±4,2).
Major axis y=2 and minor axis as x=1.
The graph of the relation is as follows.
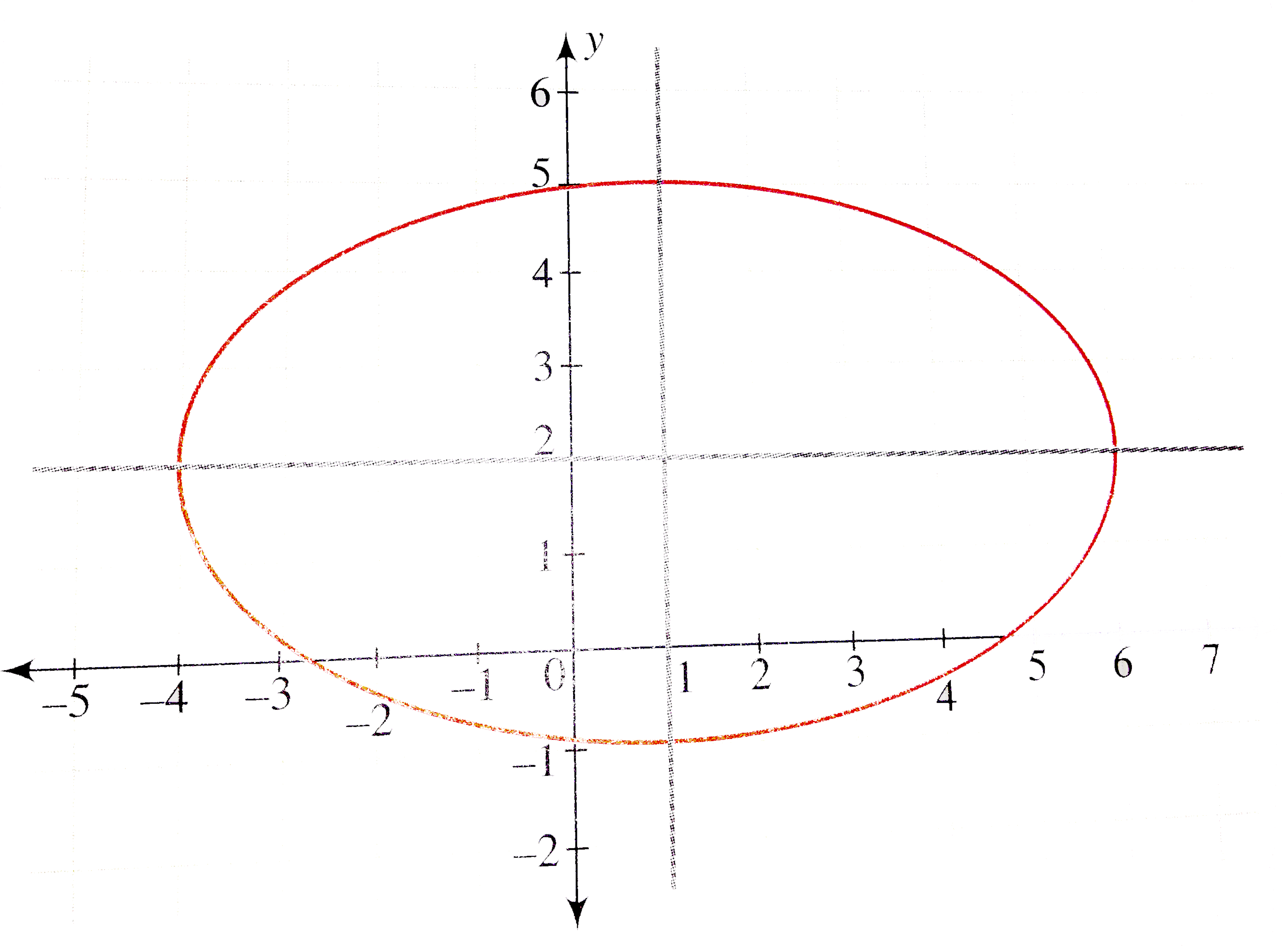
|
Topper's Solved these Questions
- GRAPHS OF ELEMENTARY FUNCTIONS BOOK - CENGAGECHAPTER - GRAPHS OF ELEMENTARY FUNCTIONS EXERCISE - Exercise
34 Videos
- GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSBOOK - CENGAGECHAPTER - GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSEXERCISE - Exercise
13 Videos
- GRAPHS OF TRIGONOMETRIC FUNCTIONSBOOK - CENGAGECHAPTER - GRAPHS OF TRIGONOMETRIC FUNCTIONSEXERCISE - Exercise
22 Videos
Similar Questions
Knowledge Check
Eccentricity of the ellipse x225+y29=1 is
A45B54C35D49The eccentricity of the ellipse 25x2+16y2−150x−175=0 is
A25B23C45D35The eccentricity of the hyperbola 9x2−16y2−18x−64y−199=0 is:
A169B54C2516Dzero
The centre of the ellipse 9x2+25y2−18x−100y−160=0 is
View SolutionFoci of the ellipse 16x2+25y2=400 are
View SolutionThe eccentricity of the hyperbola 9x2−16y2−18x−64y−199=0 is:
View SolutionThe centre of the ellipse 4x2+9y2+16x−18y−11=0 is
View SolutionThe centre of the ellipse 4x2+9y2+16−18y−11=0 is
View Solution
