The following data gives the distribution of total household expenditure (in rupees) of manual workers in a city: Expenditure (Rs.) Frequency Expenditure ( Rs.) Frequency 1000-1500 24 3000-3500 30 1500-2000 40 3500-4000 22 2000-2500 33 4000-4500 16 2500-3000 28 4500-5000 7 Find the average expenditure which is being done by the maximum number of manual workers.
To find the average expenditure of manual workers based on the given frequency distribution, we will follow these steps:
Step 1: Create a Frequency Distribution Table
We will first organize the data into a table format.
| Expenditure (Rs.) | Frequency (f) |
|-------------------|---------------|
| 1000 - 1500 | 24 |
| 1500 - 2000 | 40 |
| 2000 - 2500 | 33 |
| 2500 - 3000 | 28 |
| 3000 - 3500 | 30 |
| 3500 - 4000 | 22 |
| 4000 - 4500 | 16 |
| 4500 - 5000 | 7 |
Step 2: Calculate Midpoints (x_i)
Next, we calculate the midpoints for each expenditure class. The midpoint is calculated as:
xi=Lower Limit+Upper Limit2
| Expenditure (Rs.) | Frequency (f) | Midpoint (x_i) |
|-------------------|---------------|-----------------|
| 1000 - 1500 | 24 | 1250 |
| 1500 - 2000 | 40 | 1750 |
| 2000 - 2500 | 33 | 2250 |
| 2500 - 3000 | 28 | 2750 |
| 3000 - 3500 | 30 | 3250 |
| 3500 - 4000 | 22 | 3750 |
| 4000 - 4500 | 16 | 4250 |
| 4500 - 5000 | 7 | 4750 |
Step 3: Calculate the Total Frequency
Now, we sum up the frequencies:
Σf=24+40+33+28+30+22+16+7=200
Step 4: Calculate the Assumed Mean (a)
We will choose the assumed mean (a) as the midpoint of the class where the highest frequency occurs. The highest frequency is 40 (for the class 1500 - 2000), so:
a=1750
Step 5: Calculate the Deviation (u_i)
We calculate the deviation of each midpoint from the assumed mean divided by the class width (h = 500):
ui=xi−ah
| Midpoint (x_i) | Deviation (u_i) |
|-----------------|------------------|
| 1250 | -1 |
| 1750 | 0 |
| 2250 | 1 |
| 2750 | 2 |
| 3250 | 3 |
| 3750 | 4 |
| 4250 | 5 |
| 4750 | 6 |
Step 6: Calculate f_i * u_i
Now, we multiply each frequency by its corresponding deviation:
| Frequency (f) | Deviation (u_i) | f_i * u_i |
|---------------|------------------|-----------|
| 24 | -1 | -24 |
| 40 | 0 | 0 |
| 33 | 1 | 33 |
| 28 | 2 | 56 |
| 30 | 3 | 90 |
| 22 | 4 | 88 |
| 16 | 5 | 80 |
| 7 | 6 | 42 |
Step 7: Sum of f_i * u_i
Now, we sum up the values of f_i * u_i:
Σfiui=−24+0+33+56+90+88+80+42=265
Step 8: Calculate the Mean
Finally, we can calculate the mean using the formula:
¯x=a+ΣfiuiΣf
Substituting the values:
¯x=1750+265200×500
Calculating:
¯x=1750+6.625×500=1750+33.125=1783.125
Final Answer
The average expenditure of manual workers is approximately 1783.13 Rs.
---
We will first organize the data into a table format.
|-------------------|---------------|
| 1000 - 1500 | 24 |
| 1500 - 2000 | 40 |
| 2000 - 2500 | 33 |
| 2500 - 3000 | 28 |
| 3000 - 3500 | 30 |
| 3500 - 4000 | 22 |
| 4000 - 4500 | 16 |
| 4500 - 5000 | 7 |
Next, we calculate the midpoints for each expenditure class. The midpoint is calculated as:
|-------------------|---------------|-----------------|
| 1000 - 1500 | 24 | 1250 |
| 1500 - 2000 | 40 | 1750 |
| 2000 - 2500 | 33 | 2250 |
| 2500 - 3000 | 28 | 2750 |
| 3000 - 3500 | 30 | 3250 |
| 3500 - 4000 | 22 | 3750 |
| 4000 - 4500 | 16 | 4250 |
| 4500 - 5000 | 7 | 4750 |
Now, we sum up the frequencies:
We will choose the assumed mean (a) as the midpoint of the class where the highest frequency occurs. The highest frequency is 40 (for the class 1500 - 2000), so:
We calculate the deviation of each midpoint from the assumed mean divided by the class width (h = 500):
|-----------------|------------------|
| 1250 | -1 |
| 1750 | 0 |
| 2250 | 1 |
| 2750 | 2 |
| 3250 | 3 |
| 3750 | 4 |
| 4250 | 5 |
| 4750 | 6 |
Now, we multiply each frequency by its corresponding deviation:
|---------------|------------------|-----------|
| 24 | -1 | -24 |
| 40 | 0 | 0 |
| 33 | 1 | 33 |
| 28 | 2 | 56 |
| 30 | 3 | 90 |
| 22 | 4 | 88 |
| 16 | 5 | 80 |
| 7 | 6 | 42 |
Now, we sum up the values of f_i * u_i:
Finally, we can calculate the mean using the formula:
The average expenditure of manual workers is approximately 1783.13 Rs.
Topper's Solved these Questions
Similar Questions
The following data gives the distribution of total household expendilture ( in Rs.) of workers is a city :
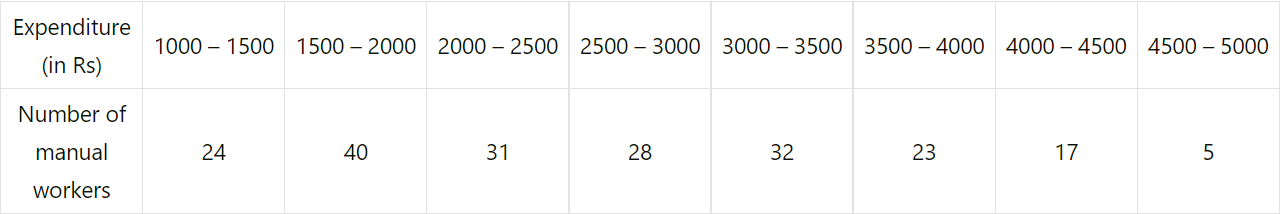
Find the average expenditure which is being done by maximum number of manual workers.View SolutionThe following data gives the distribution of total monthly household expenditure of 200 families of a village. Find the modal monthly expenditure of the families. Also, find the mean monthly expenditure: Expenditure (Rs.) Frequency Expenditure (Rs.) Frequency 1000-1500 24 3000-3500 30 1500-2000 40 3500-4000 22 2000-2500 33 4000-4500 16 2500-3000 28 4500-5000 7
View SolutionThe following table gives the distribution of total household expenditure (in rupees) of manual workers in a city. Expenditure (in rupees) (xi) Frequency (fi) 100-150 24 150-200 40 200-250 33 250-300 28 300-350 30 350-450 22 400-450 16 450-500 7 Find the average expenditure (in rupees) per household.
View SolutionThe following table gives the distribution of total household expenditure (in rupees) of manual workers in a city. Expenditure: (in Rs.) 100-150 150-200 200-250 250-300 300-350 350-400 400-450 450-500 Frequency: 24 40 33 28 30 22 16 7 Find the average expenditure (in Rs.) per household.
View SolutionDraw a pie-diagram for the following data of expenditure pattern in a family: Items, Food, Clothing, Rent, Edu., Misc. Expenditure (in Rs.), 4000, 2000, 1500, 1500, 1000
View SolutionThe following table gives the distribution of the life time of 400 neon lamps: Lite time: (in hours) Number of lamps 1500−2000 14 2000-2500 56 2500-3000 60 3000-3500 86 3500-4000 74 4000-4500 62 4500-5000 48 Find the median life.
View Solution

