The median of the following data is 525. Find the values of x and y, if the total frequency is 100.
To solve the problem, we need to find the values of x and y given that the median of the data is 525 and the total frequency is 100. We will use the median formula for grouped data.
Step 1: Understand the Median Formula
The median for grouped data is given by the formula:
Median=l+n/2−cff×h
where:
- l = lower limit of the median class
- n = total number of observations
- cf = cumulative frequency of the class preceding the median class
- f = frequency of the median class
- h = width of the class interval
Step 2: Set Up the Cumulative Frequency Table
We need to create a cumulative frequency table. The total frequency is 100, and we will denote the frequencies of the classes as follows:
| Class Interval | Frequency |
|----------------|-----------|
| 0 - 100 | 2 |
| 100 - 200 | 5 |
| 200 - 300 | x |
| 300 - 400 | 12 |
| 400 - 500 | 17 |
| 500 - 600 | 20 |
| 600 - 700 | y |
| 700 - 800 | 4 |
| Total | 100 |
Now, we can calculate the cumulative frequencies:
- Cumulative frequency for 0 - 100: 2
- Cumulative frequency for 100 - 200: 2+5=7
- Cumulative frequency for 200 - 300: 7+x=7+x
- Cumulative frequency for 300 - 400: 7+x+12=19+x
- Cumulative frequency for 400 - 500: 19+x+17=36+x
- Cumulative frequency for 500 - 600: 36+x+20=56+x
- Cumulative frequency for 600 - 700: 56+x+y
- Cumulative frequency for 700 - 800: 56+x+y+4=60+x+y
Step 3: Set Up the Equation for Total Frequency
From the total frequency, we have:
2+5+x+12+17+20+y+4=100
This simplifies to:
60+x+y=100
Thus, we can express x+y as:
x+y=100−60=40(Equation 1)
Step 4: Identify the Median Class
Since the median is 525, the median class is the class interval containing 525, which is 500 - 600.
Step 5: Find Values for l, cf, f, and h
- l=500
- cf=36+x (cumulative frequency of the class preceding the median class)
- f=20 (frequency of the median class)
- h=100 (width of the class interval)
Step 6: Substitute Values into the Median Formula
Using the median formula:
525=500+50−(36+x)20×100
Step 7: Simplify the Equation
Subtract 500 from both sides:
25=50−(36+x)20×100
Multiply both sides by 20:
500=50−(36+x)
Rearranging gives:
500=50−36−x
500=14−x
Thus,
x=14−500=−486(not possible, check calculations)
Step 8: Correct the Calculation
Going back to the equation:
25=50−(36+x)20×100
Multiply both sides by 20:
500=50−(36+x)
This simplifies to:
500=14−x
Thus,
x=14−500=−486(not possible, check calculations)
Step 9: Solve for x and y
From Equation 1:
x+y=40
Substituting x=9 into this gives:
9+y=40⟹y=40−9=31
Final Values
Thus, the values of x and y are:
x=9,y=31
The median for grouped data is given by the formula:
Median=l+n/2−cff×h
where:
- l = lower limit of the median class
- n = total number of observations
- cf = cumulative frequency of the class preceding the median class
- f = frequency of the median class
- h = width of the class interval
We need to create a cumulative frequency table. The total frequency is 100, and we will denote the frequencies of the classes as follows:
|----------------|-----------|
| 0 - 100 | 2 |
| 100 - 200 | 5 |
| 200 - 300 | x |
| 300 - 400 | 12 |
| 400 - 500 | 17 |
| 500 - 600 | 20 |
| 600 - 700 | y |
| 700 - 800 | 4 |
| Total | 100 |
- Cumulative frequency for 100 - 200: 2+5=7
- Cumulative frequency for 200 - 300: 7+x=7+x
- Cumulative frequency for 300 - 400: 7+x+12=19+x
- Cumulative frequency for 400 - 500: 19+x+17=36+x
- Cumulative frequency for 500 - 600: 36+x+20=56+x
- Cumulative frequency for 600 - 700: 56+x+y
- Cumulative frequency for 700 - 800: 56+x+y+4=60+x+y
From the total frequency, we have:
2+5+x+12+17+20+y+4=100
This simplifies to:
60+x+y=100
Thus, we can express x+y as:
x+y=100−60=40(Equation 1)
Since the median is 525, the median class is the class interval containing 525, which is 500 - 600.
- l=500
- cf=36+x (cumulative frequency of the class preceding the median class)
- f=20 (frequency of the median class)
- h=100 (width of the class interval)
Using the median formula:
525=500+50−(36+x)20×100
Subtract 500 from both sides:
25=50−(36+x)20×100
Multiply both sides by 20:
500=50−(36+x)
Rearranging gives:
500=50−36−x
500=14−x
Thus,
x=14−500=−486(not possible, check calculations)
Going back to the equation:
25=50−(36+x)20×100
Multiply both sides by 20:
500=50−(36+x)
This simplifies to:
500=14−x
Thus,
x=14−500=−486(not possible, check calculations)
From Equation 1:
x+y=40
Substituting x=9 into this gives:
9+y=40⟹y=40−9=31
Thus, the values of x and y are:
x=9,y=31
Topper's Solved these Questions
- BOOK - NCERT ENGLISHCHAPTER - STATISTICSEXERCISE - EXERCISE 14.43videos
STATISTICS
- BOOK - NCERT ENGLISHCHAPTER - STATISTICSEXERCISE - Exercise 14.37videos
STATISTICS
- BOOK - NCERT ENGLISHCHAPTER - SOME APPLICATIONS OF TRIGONOMETRYEXERCISE - SOLVED EXAMPLES7videos
SOME APPLICATIONS OF TRIGONOMETRY
- BOOK - NCERT ENGLISHCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - Exercise 13.39videos
SURFACE AREAS AND VOLUMES
Similar Questions
If the median of the following distribution is 28, find the values of x and y.If the total frequency is 50.
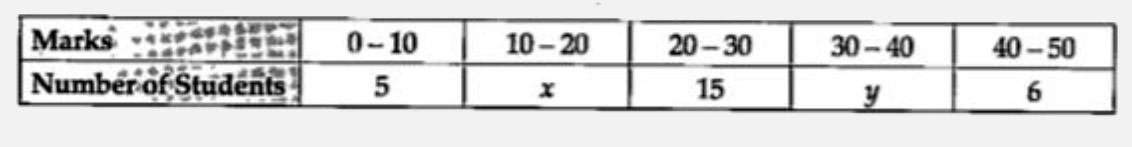 View Solution
View SolutionFind the median of the following data
View SolutionFind the median of the following data
View SolutionFind the median of the following data
View SolutionFind the median of the following data
View SolutionFind the median of the following data
View SolutionFind the median of the following data
View SolutionFind the median of the following data
View SolutionThe median of the following data is 50. Find the values of p and q, if the sum of the all the frequencies is 90.
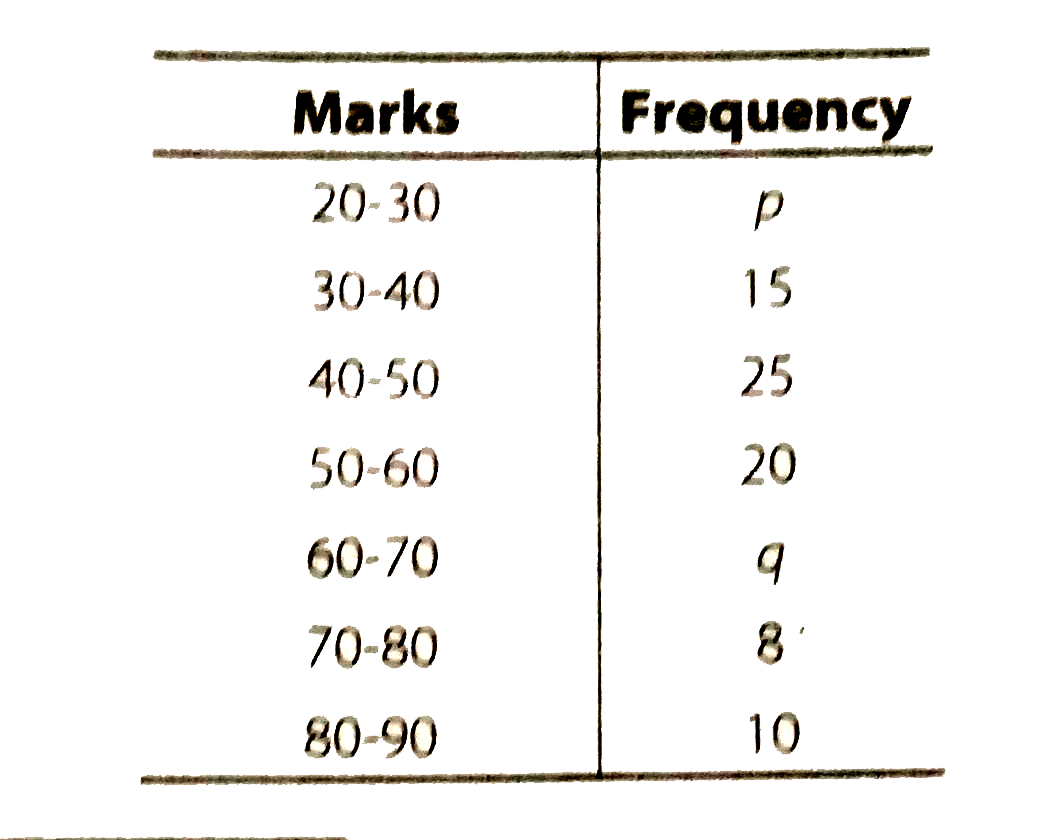 View Solution
View SolutionFind the median of the following data.
View Solution

