Question

The objective function $Z=x_1+x_2$ subject to the constraints: $x_1+x_2\le 10,-2x_1+3x_2\le 15,x_1\le 6,x_1,x_2\ge 0$ has maximum value ________________ of the feasible region .

Options

A. at only two points
B. at only two points
C. at every point of the segment joining two points
D. at every point of the line joining two points

Answer

C

Explanation

Give ,objective function ` Z=x_1 +x_2 ` and constraints are ` x_1 +x_2 le 10, -2x_1 +3x_2 le 15 , x le 6,x_1x_2 ge 0`
The point of intersection lo lines ` x_1+x_2= 10` na `-2x_1+3x_2= 15 ` is B(3,7) and point of intersection of lines ` x_1 = 6 " and " x_1 +x_2= 10` is C (6,4).

The feasible region is OABCD. The corner points of the feasible region are 0(0, 0), A(0, 6), 8(3, 7), C(6, 4) and 0(6, O).

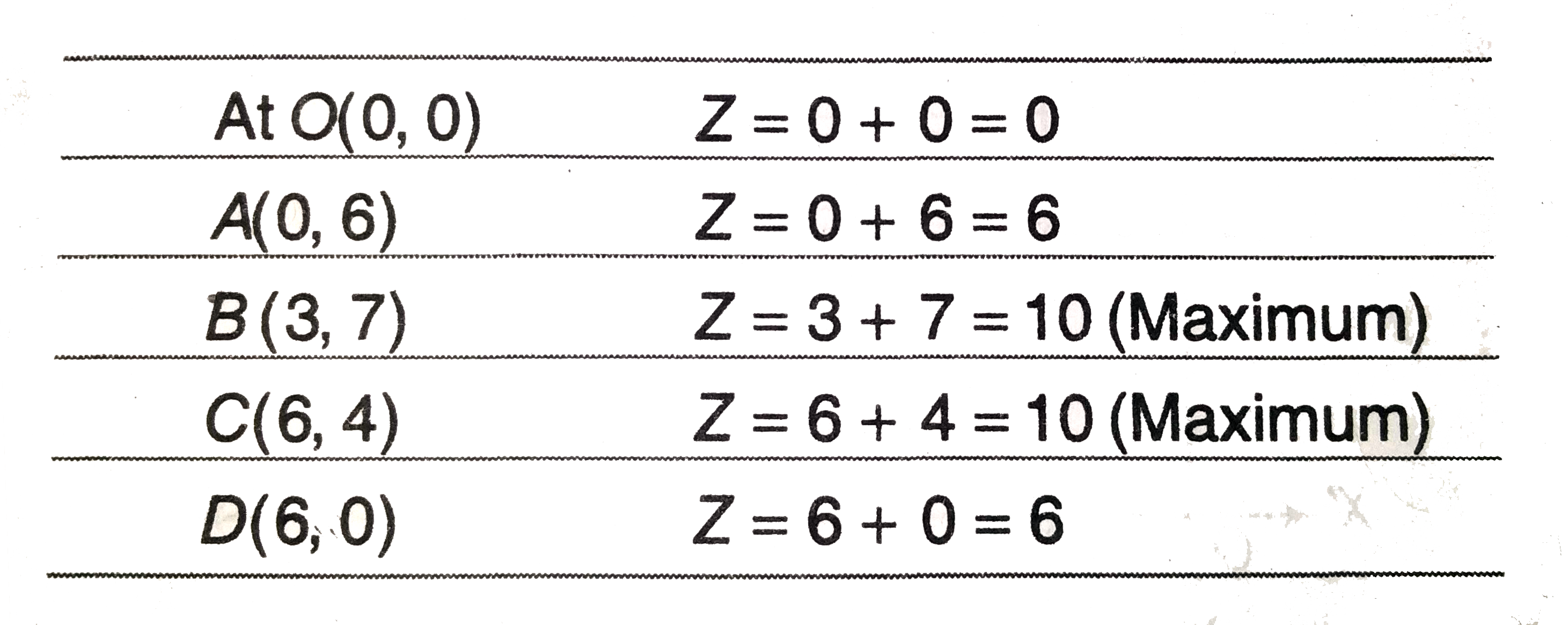
Hence,Z is maximum at every point at every point the segment which combined tow points.