The perpendicular from A on side BC of a ΔABC meets BC at D such that DB = 3CD .Prove that 2AB2=2AC2+BC2.
To prove that 2AB2=2AC2+BC2 given that the perpendicular from A on side BC meets BC at D such that DB=3CD, we can follow these steps:
Step 1: Set Up the Triangle
Let D be the point where the perpendicular from A meets BC. According to the problem, we have:
DB=3CD
Let CD=x. Then, we can express DB as:
DB=3x
Step 2: Express BC in Terms of x
Now, we can find BC:
BC=BD+CD=3x+x=4x
Step 3: Apply the Pythagorean Theorem
We can apply the Pythagorean theorem in triangles ABD and ACD.
For triangle ABD:
AB2=AD2+BD2
For triangle ACD:
AC2=AD2+CD2
Step 4: Substitute BD and CD
From the previous steps, we know:
- BD=3x
- CD=x
Substituting these into the equations gives us:
1. AB2=AD2+(3x)2
2. AC2=AD2+x2
Step 5: Rearranging the Equations
From the equations above, we can express AD2:
1. AB2=AD2+9x2 (1)
2. AC2=AD2+x2 (2)
From equation (2), we can express AD2 as:
AD2=AC2−x2
Step 6: Substitute AD2 in Equation (1)
Substituting AD2 from equation (2) into equation (1):
AB2=(AC2−x2)+9x2
This simplifies to:
AB2=AC2+8x2
Step 7: Express x2 in Terms of BC
Since we have BC=4x, we can express x as:
x=BC4
Thus,
x2=(BC4)2=BC216
Step 8: Substitute x2 Back
Substituting x2 back into the equation:
AB2=AC2+8(BC216)
This simplifies to:
AB2=AC2+8BC216=AC2+BC22
Step 9: Multiply by 2
Now, multiplying the entire equation by 2 gives:
2AB2=2AC2+BC2
Conclusion
Thus, we have proved that:
2AB2=2AC2+BC2
Let D be the point where the perpendicular from A meets BC. According to the problem, we have:
DB=3CD
Let CD=x. Then, we can express DB as:
DB=3x
Now, we can find BC:
BC=BD+CD=3x+x=4x
We can apply the Pythagorean theorem in triangles ABD and ACD.
AB2=AD2+BD2
For triangle ACD:
AC2=AD2+CD2
From the previous steps, we know:
- BD=3x
- CD=x
1. AB2=AD2+(3x)2
2. AC2=AD2+x2
From the equations above, we can express AD2:
1. AB2=AD2+9x2 (1)
2. AC2=AD2+x2 (2)
AD2=AC2−x2
Substituting AD2 from equation (2) into equation (1):
AB2=(AC2−x2)+9x2
This simplifies to:
AB2=AC2+8x2
Since we have BC=4x, we can express x as:
x=BC4
Thus,
x2=(BC4)2=BC216
Substituting x2 back into the equation:
AB2=AC2+8(BC216)
This simplifies to:
AB2=AC2+8BC216=AC2+BC22
Now, multiplying the entire equation by 2 gives:
2AB2=2AC2+BC2
Thus, we have proved that:
2AB2=2AC2+BC2
Topper's Solved these Questions
- BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 2019EXERCISE - Section - D12videos
SAMPLE PAPER 2019
- BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 2019EXERCISE - Section - B8videos
SAMPLE PAPER 2019
- BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 2 EXERCISE - SECTION - D9videos
SAMPLE PAPER 2
- BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 3 [CBSE 2019 (TOPPER SOLVED)]EXERCISE - SECTION - D12videos
SAMPLE PAPER 3 [CBSE 2019 (TOPPER SOLVED)]
Similar Questions
The perpendicular from A on side BC of a ΔABC meets BC at D such that DB = 3CD. Prove that 2AB2=2AC2+BC2
View SolutionThe perpendicular drawn from A on sides BC of a ΔABC intersects BC at D such that DB = 3CD .Prove that 2AB2=2AC2+BC2.
View SolutionThe perpendicular from A on side BC of a ABC intersects BC at D such that DB = 3 CD. Prove that 2AB2=2AC2+BC2.
View SolutionThe perpendicular AD on the base BC of a ABC intersects BC at D so that DB=3CD . Prove that 2 AB2=2 AC2+BC2 .
View SolutionThe perpendicular AD on the base BC of a △ABC intersects BC at D, so that DB=3CD. Prove that 2AB2=2AC2+BC2
View SolutionIn ΔABC,
seg AD⊥ seg BC,
DB=3CD.
Prove that 2AB2=2AC2+BC2.
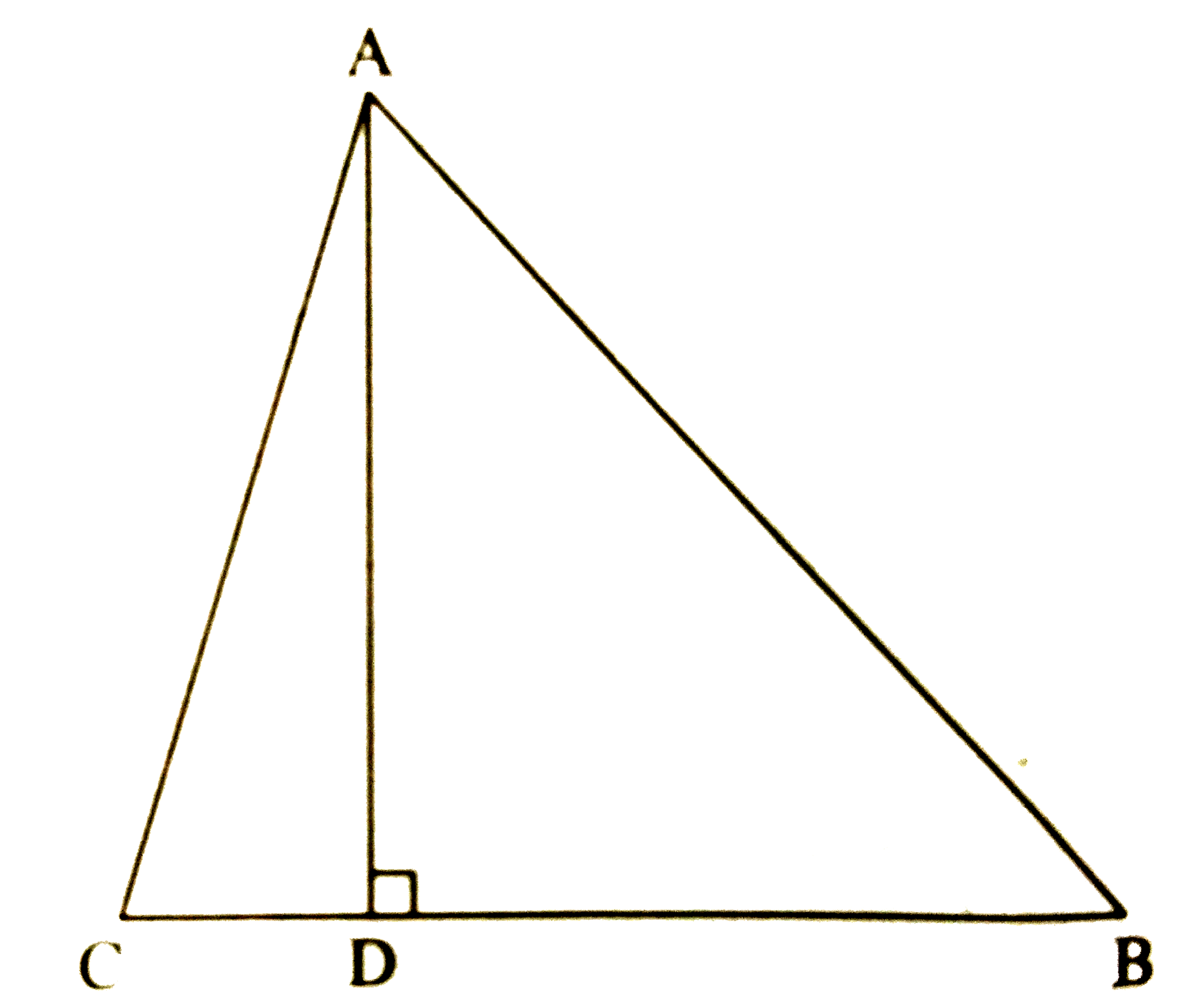 View Solution
View SolutionIn the given figure, the of a ΔABC, interesects BC at D such DB=3CD. Prove that 2AB2=2AC2+BC2
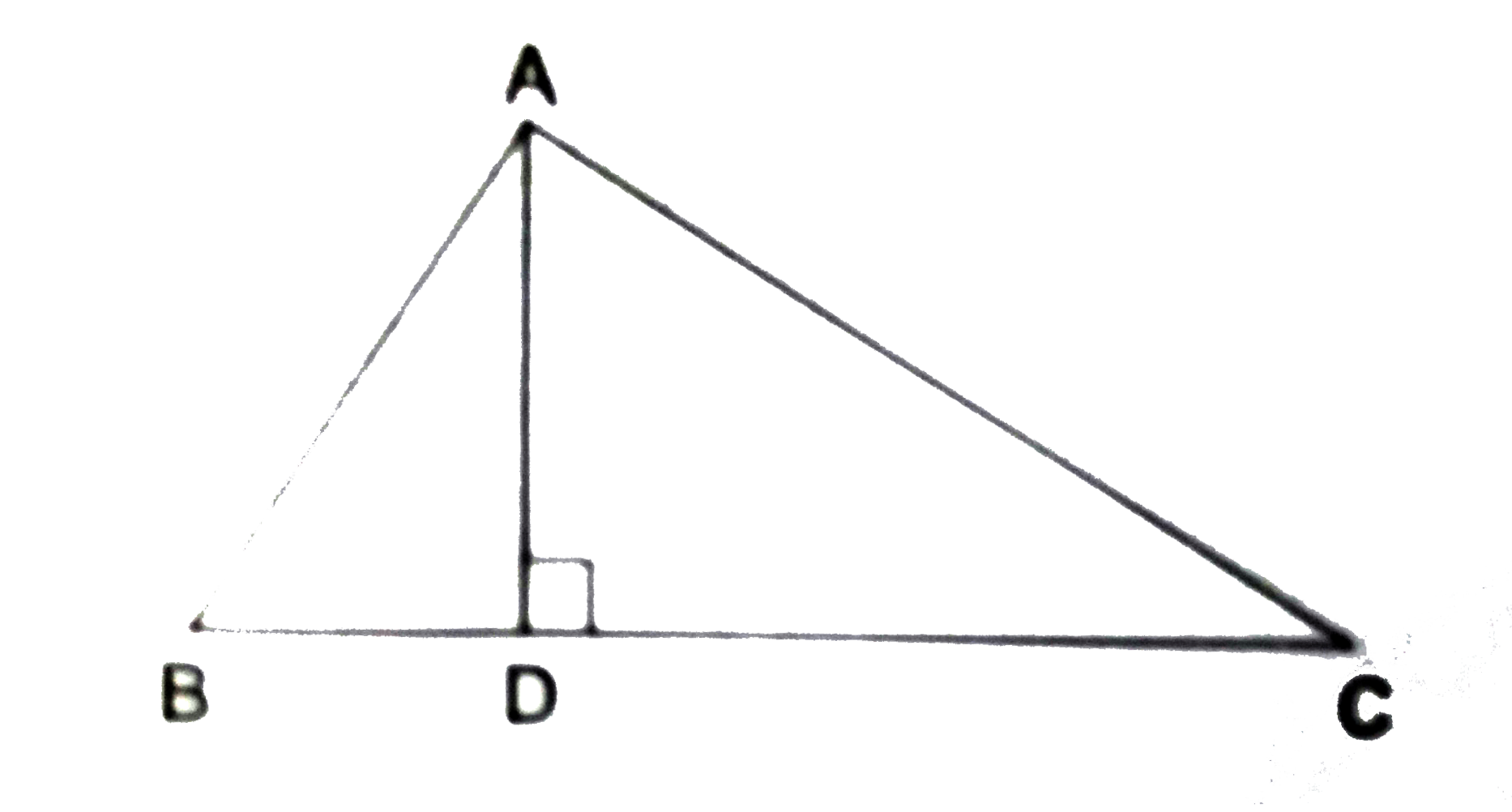 View Solution
View SolutionIn a ΔABC, altitude drawn from vertex A to side BC meets BC at D such that DB=3CD. Prove that 2AB2=2AC2+BC2
View SolutionIn ΔABC seg AD⊥segBC,DB=3CD Prove that 2AB2=2AC2+BC2
View SolutionIn ΔABC, seg AD⊥ seg BCDB=3CD.
Prove that : 2AB2=2AC2+BC2
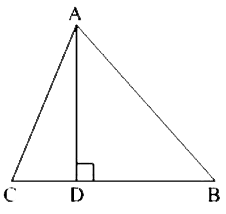 View Solution
View Solution

