The relationship between mean, median and mode for a moderately skewed distribution is (a) Mode = 2 Median – 3 Mean (b) Mode = Median – 2 Mean (c) Mode = 2 Median – Mean (d) Mode = 3 Median – 2 mean
To solve the problem regarding the relationship between mean, median, and mode for a moderately skewed distribution, we can follow these steps:
Step 1: Understand the relationship
For a moderately skewed distribution, it is generally accepted that:
- Mode < Median < Mean
Step 2: Use Karl Pearson's formula
According to Karl Pearson's formula for skewness, we can express the relationship as follows:
Mean=13(Mean−Mode)+Median
Step 3: Rearranging the formula
We can rearrange the formula to isolate the terms:
1. Multiply both sides by 3:
3×Mean=Mean−Mode+3×Median
2. Rearranging gives:
3×Mean−Mean+Mode=3×Median
2×Mean=−Mode+3×Median
Step 4: Isolate Mode
Now, we can isolate the mode:
Mode=3×Median−2×Mean
Step 5: Conclusion
Thus, we find that the relationship between mean, median, and mode for a moderately skewed distribution is:
Mode=3×Median−2×Mean
Final Answer
The correct option is (d) Mode = 3 Median – 2 Mean.
---
For a moderately skewed distribution, it is generally accepted that:
- Mode < Median < Mean
According to Karl Pearson's formula for skewness, we can express the relationship as follows:
Mean=13(Mean−Mode)+Median
We can rearrange the formula to isolate the terms:
1. Multiply both sides by 3:
3×Mean=Mean−Mode+3×Median
3×Mean−Mean+Mode=3×Median
2×Mean=−Mode+3×Median
Now, we can isolate the mode:
Mode=3×Median−2×Mean
Thus, we find that the relationship between mean, median, and mode for a moderately skewed distribution is:
Mode=3×Median−2×Mean
The correct option is (d) Mode = 3 Median – 2 Mean.
Topper's Solved these Questions
Similar Questions
One of the methods of determining mode is (a) Mode = 2 Median – 3 Mean (b) Mode = 2 Median + 3 Mean (c) Mode = 3 Median – 2 Mean (d) Mode = 3 Median + 2 Mean
View SolutionFor a frequency distribution, mean, median and mode are connected by the relation (a) Mode = 3 Mean – 2 median (b) Mode = 2 Median – 3 Mean (c) Mode = 3 Median – 2 Mean (d) Mode = 3 Median + 2 Mean
View Solution
Knowledge Check
- Question 1 - Select One
If the mean and the mode of a moderately skewed frequency distribution are 90 and 96 respectively, then the median is
A93B92C98D88
Find the median and mode of the data:
View SolutionIf for a moderately skewed distribution, mode = 60 and mean = 66, then median =
View SolutionFind the mean, median and mode of the following data
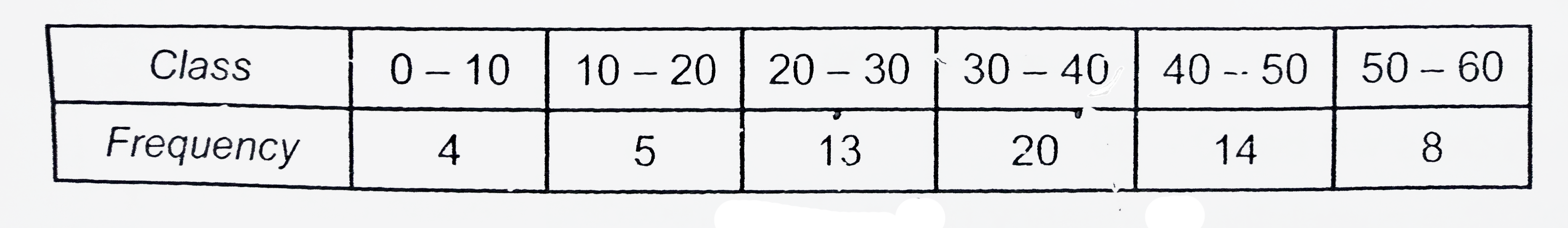 View Solution
View SolutionFind the mean, median and mode of the following data :
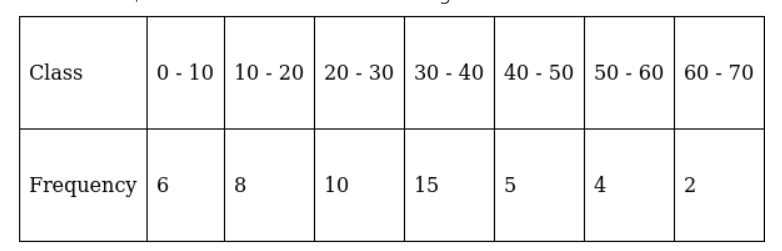 View Solution
View SolutionFind mean, median and mode of the following data :
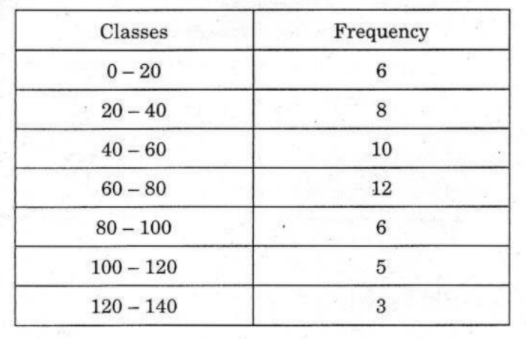 View Solution
View SolutionCalculate the mean, the median and the mode of the following distribution:
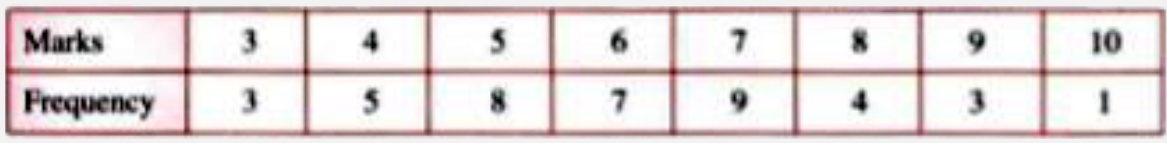 View Solution
View SolutionFind mean and mode of 2,2,3,1.
View Solution

