The width of each of five continuous classes in a frequency distribution is 5 and the lower class limit of the lowest class is 10. The upper class limit of the highest class is
15
25
35
40
The correct Answer is:C
To solve the problem step by step, let's break it down clearly:
Step 1: Understand the Given Information
We are given:
- The width of each class = 5
- The lower class limit of the lowest class = 10
- The number of classes = 5
Step 2: Determine the Lower and Upper Class Limits of the First Class
The first class can be represented as:
- Lower class limit (x) = 10
- Upper class limit (y) = x + width = 10 + 5 = 15
So, the first class is 10 to 15.
Step 3: Determine the Subsequent Classes
Since the classes are continuous and each has a width of 5, we can find the remaining classes:
1. First class: 10 to 15
2. Second class: 15 to 20
3. Third class: 20 to 25
4. Fourth class: 25 to 30
5. Fifth class: 30 to 35
Step 4: Identify the Upper Class Limit of the Highest Class
The highest class is the fifth class, which is:
- Lower class limit = 30
- Upper class limit = 35
Conclusion
Therefore, the upper class limit of the highest class is 35.
---
We are given:
- The width of each class = 5
- The lower class limit of the lowest class = 10
- The number of classes = 5
The first class can be represented as:
- Lower class limit (x) = 10
- Upper class limit (y) = x + width = 10 + 5 = 15
Since the classes are continuous and each has a width of 5, we can find the remaining classes:
1. First class: 10 to 15
2. Second class: 15 to 20
3. Third class: 20 to 25
4. Fourth class: 25 to 30
5. Fifth class: 30 to 35
The highest class is the fifth class, which is:
- Lower class limit = 30
- Upper class limit = 35
Therefore, the upper class limit of the highest class is 35.
Topper's Solved these Questions
- STATISTICS AND PROBABILITYBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - STATISTICS AND PROBABILITYEXERCISE - VERY SHORT ANSWER TYPES QUESTIONS
10 Videos
- STATISTICS AND PROBABILITYBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - STATISTICS AND PROBABILITYEXERCISE - SHORT ANSWER TYPE QUESTIONS
20 Videos
- QUADRILATERALS BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - QUADRILATERALS EXERCISE - LONG ANSWER TYPE QUESTIONS
18 Videos
- TRIANGLESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - TRIANGLESEXERCISE - LONG ANSWER TYPE QUESTIONS
21 Videos
Similar Questions
The width of each of nine classes in a frequency distribution is 2.5 and the lower class boundary of the lowest class 10.6. Then the upper class boundary of the highest class is (a) 35.6 (b) 33.1 (c) 30.6 (d) 28.1
View SolutionIn a frequency distribution, the mid values of a class is 10 and width of the class is 6. The lower limit of the class is
View SolutionIf m is the mid-point and l is the upper limit of a class in a continuous frequency distribution, then lower class limit of the class is
View SolutionLet l be the lower class limit of a class-interval in a frequency distribution and m be the mid-point of the class. Then, the upper class limit of the class is (a) m+l+m2 (b) l+m+12 (c) 2m−l (d) (m−2l)
View SolutionConsider the following frequency distribution

The upper limit of the median class in
View SolutionFor the following distribution
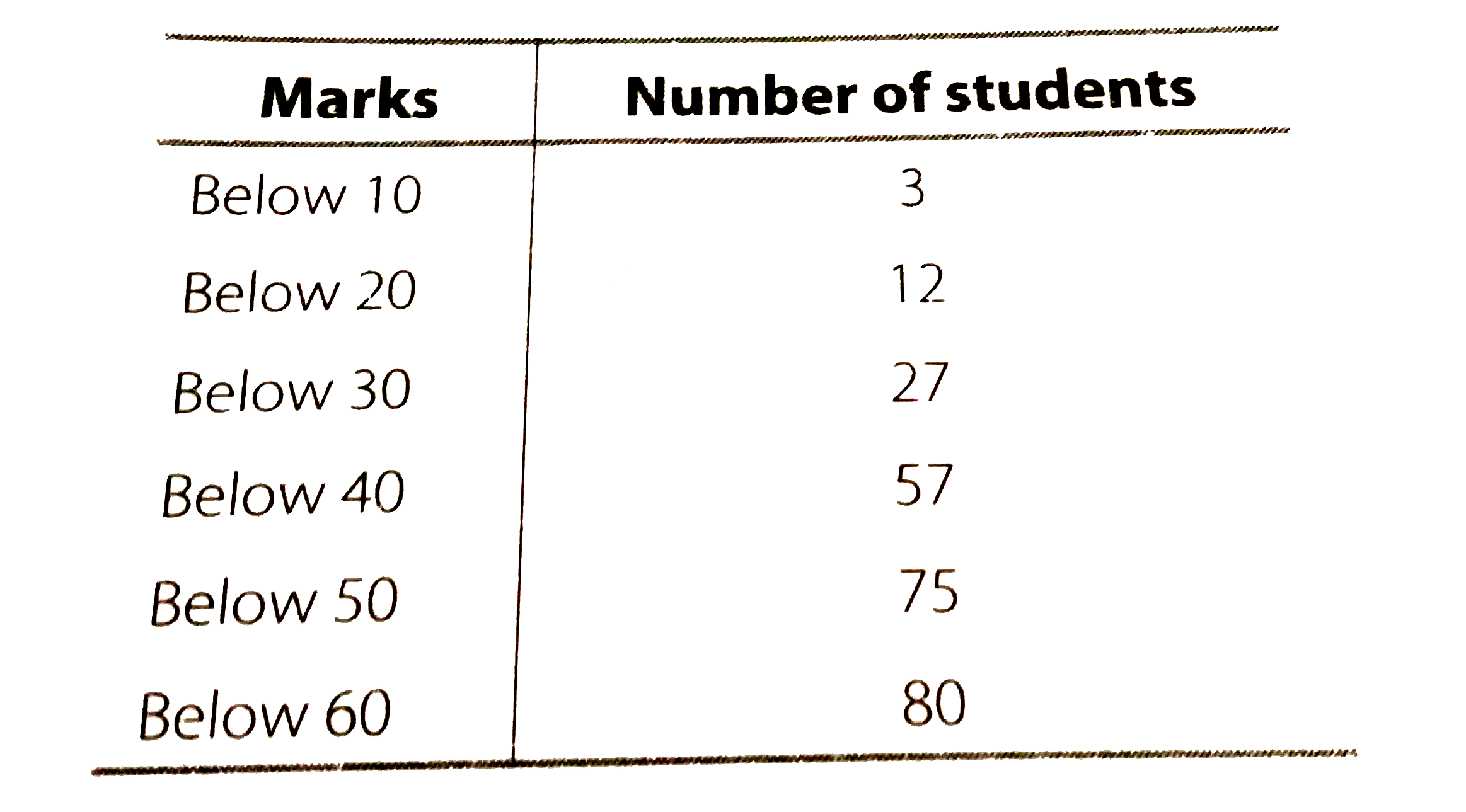
the modal class is
View SolutionFor the following frequency distribution

The upper limit of median class is
View SolutionIn a frequency distribution, the mid-value of a class is 15 and the class intervals is 4. The lower limit of the class is 10 (b) 12 (c) 13 (d) 14
View SolutionThe following are weights (in kg) of 50 children taken at the time of birth:
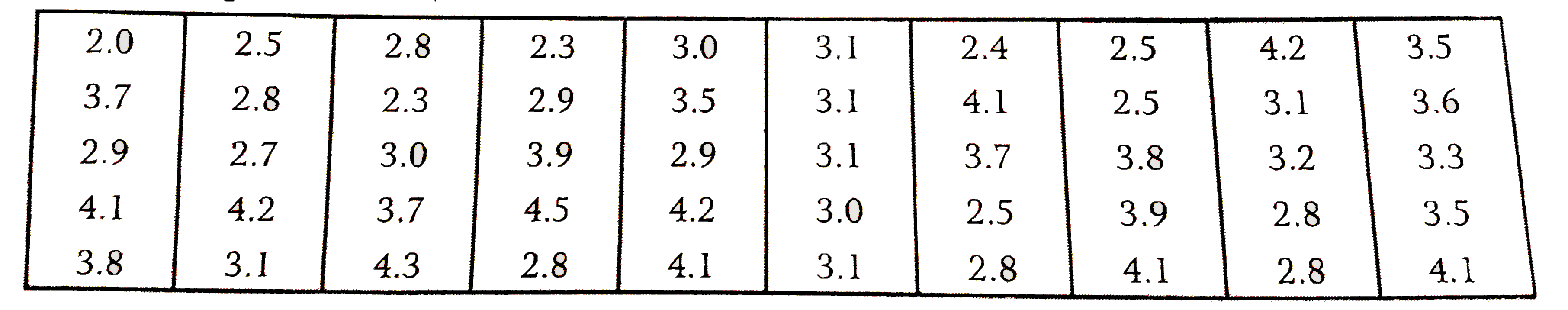
Make an inclusive form grouped frequency distribution table with 0.3 kg as the width of ' each class.
Also, find the true class limits of each class.
View SolutionPrepare a continuous grouped frequency distribution from the following data

Also, find the size of class intervals.
View Solution
