Triangle ABC is right angled at A. The circle with centre A and radius AB cuts BC and AC internally at D and E respectively. If BD=20 and DC=16 then the length AC equals (A) 6sqrt21 (B) 6sqrt26 (C) 30 (D)32

A

$6 \sqrt{21}$

B

$6 \sqrt{26}$

C

30

D

32

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

$b^{2} + r^{2} = {(36)}^{2}$ (1)
Also, $C D . C B = C E . C X$
$∴ 16.36 = (b - r) (b + r)$
$∴ b^{2} - r^{2} = 16 \times 36$ (2)
From (1) and (2)
$2 b^{2} + 36 (36 + 16) = 36 \times 52$
$\Rightarrow b = 6 \sqrt{26}$

|

Updated on:21/07/2023

Class 12 MATHS CIRCLES

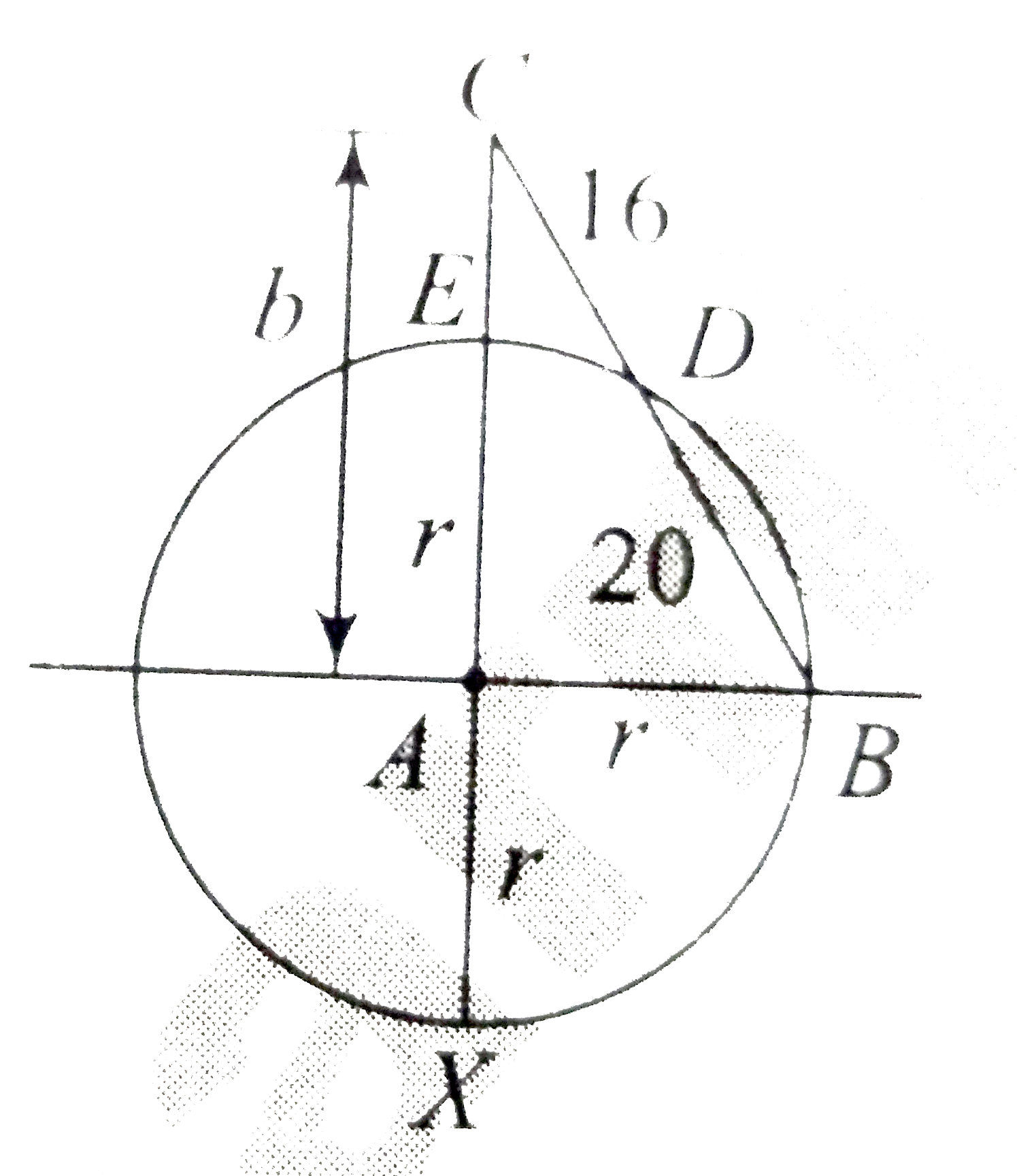
Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Triangle ABC inscribes a circle with centre O and inradius $\sqrt{6} c m$ . The inradius OD divides side BC at D, such that BD=2 cm and DC=8 cm. Find the ratio AB:AC.
A $4 : 7$
B $5 : 7$
C $4 : 5$
Dcannot be determined
Question 2 - Select One
The in circle of $Δ A B C$ touches the sides BC, CA and AB at D, E and F respectively. Then AF + BD + CE is equal to :
A $\frac{1}{2}$ (Perimeter of $Δ$ ABC)
B $\frac{1}{3}$ (Perimeter of $Δ$ ABC)
C $\frac{1}{4}$ (Perimeter of $Δ$ ABC)
DPerimeter of $Δ$ ABC
Question 3 - Select One
In triangle ABC , sides AB and AC are extended to D and E, respectively, such that $A B = B D$ and AC =CE $, F \in d D E, if$ BC=6 cm.
A3 cm
B6cm
C9 cm
D12 cm

CENGAGE-CIRCLES-Single Correct Answer Type

Home
Ask Doubt
Profile