Two circles touch each other extermally at point P. APC and BPD are straight lines. Show that :
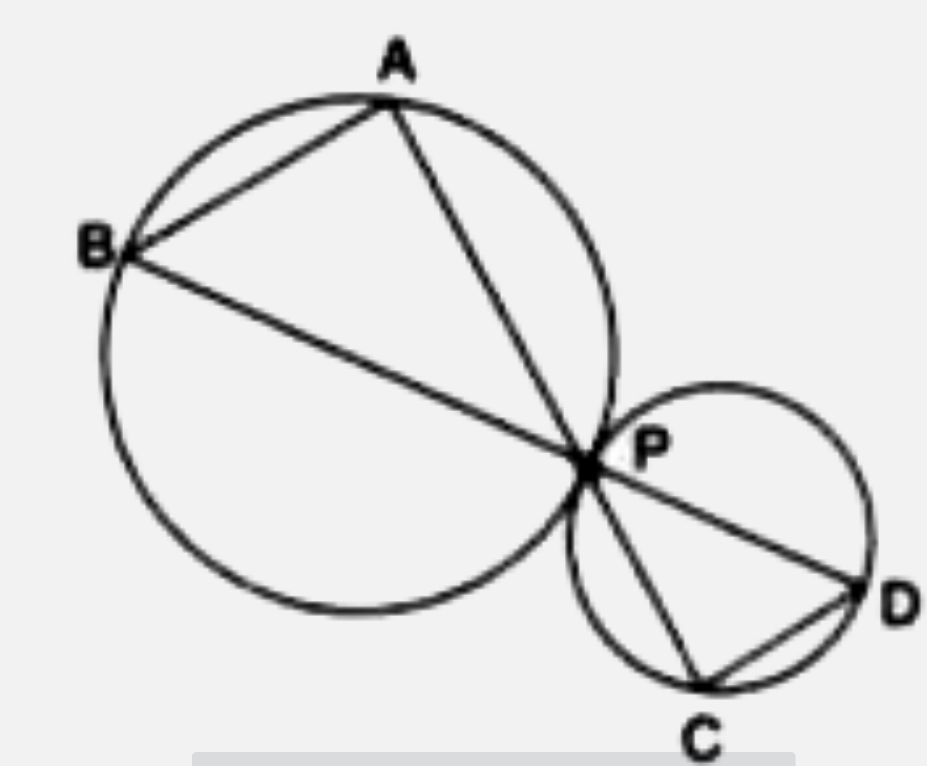
ΔPABandΔPCD are similar
The correct Answer is:4876cm3
Step by step video & image solution for Two circles touch each other extermally at point P. APC and BPD are straight lines. Show that : Delta PAB and Delta PCD are similar by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
Similar Questions
Two circles touch each other extermally at point P. APC and BPD are straight lines. Show that :

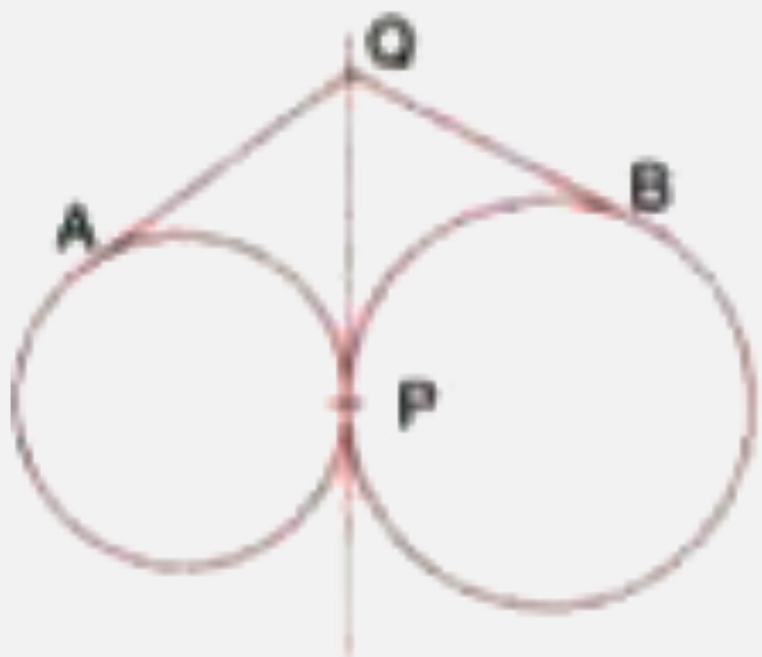
AP is parallel to CD.View SolutionTwo circles touch each other externally at point P. Q is a point on the common tangent through P. Prove that the tangents QA and QB are equal.
 View Solution
View SolutionIn the given figure, two circles touch each other externally at point P. AB is the direct common tangent of these circles. Prove that:
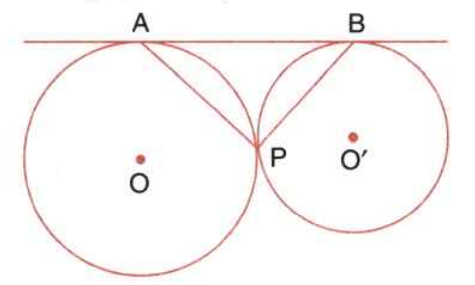
(i) tangent at point P bisects AB
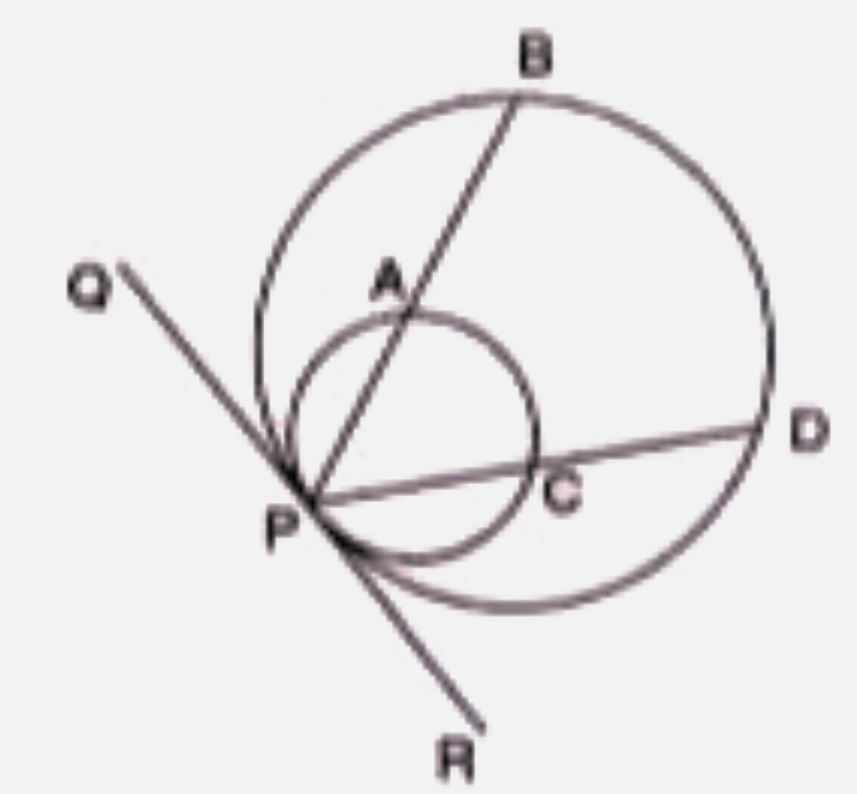
(ii) angle APB=90∘View SolutionTwo circles touch each other internally at point P. QPR is the tangent at P, segments PAB and PCD meet circles at points, A,B,C and D as shown in the figure.
Show that chord AC is parallel to chord BD.
 View Solution
View SolutionTwo circles intersect each other at points A and B. A straight line PAQ cuts the circles at P and Q. If the tangents at P and Q intersect at point T, show that the points P, B, Q and T are concylic.
View SolutionIn the figure, given below, straight lines AB and CD intersect at P, and AC//BD. Prove that :
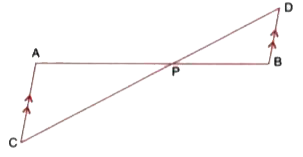
ΔAPCandΔBPD are similar.View SolutionIn the figure, two circles intersect each other at points A and B. AP and AQ are the diameters of these circels. Prove that PBQ is a straight line.
 View Solution
View SolutionIn the figure, two circles intersect each other at points A and B. AP and AQ are the diameters of these circels. Prove that PBQ is a straight line.
 View Solution
View SolutionTwo circles intersect each other at point A and B. Their common tangent touches the circles at points P and Q as shown in the figure. Show that the angles PAQ and PBQ are supplementary.
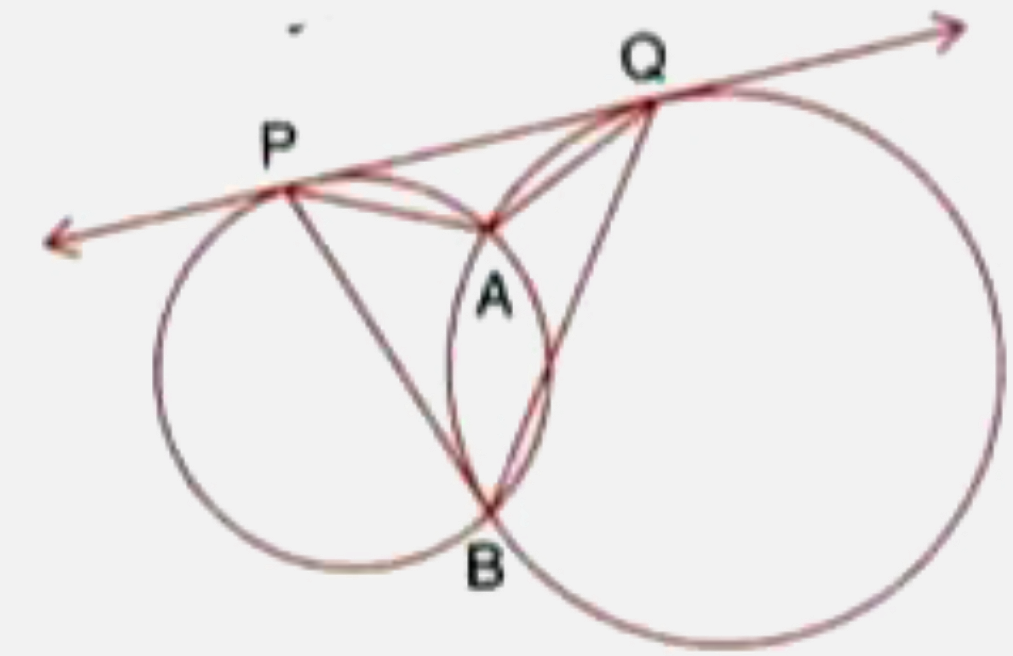 View Solution
View SolutionIn Figure, two circles touch each other at the point C . Prove that the common tangent to the circles at C , bisects the common tangent at P and Q .
View Solution

