Complete Solutions to EXERCISE of chapter SAMPLE PAPER 11 of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | SAMPLE PAPER 11 |
| AGRAWAL PUBLICATION | EXERCISE |
| Number Of Questions | 78 |
| Academic Year | 2023-24 |
Without performing actual division, check if `17/30` is a terminating decimal.
View SolutionFind the value of x so that the distance between the points (-3,4) and (x,-4) is 10 units.
View SolutionThe veticles of an equilateral trianlge ABC are (0,0)(0,y) and (3, `sqrt3`), then find the value of y.
View SolutionDefine the mode of a frequency distribution and give the formula used in computing the mode of a grouped frequency distribution.
View SolutionA right triangle has hypotenuse of length p cm and one side of length q cm . If p-q = 1, find the length of the third side of the triangle.
View SolutionIf a hexgon ABCDEF circumscribes a circle then show that AB+CD+EF =BC+DE+FA,
View SolutionThree identical cubes each of volume 27 cu cm are joined together end to end. What are the dimesions of the resulting cuboid?
View SolutionIf a chord of a circle of radius 'r' subtends a right angle at the centre of the circle, then determine the area of the correspondin segment?
View SolutionWhat is the volume of the material in a spherical shell with inner radius 'r' and outer radius 'R'?
View SolutionIf `tan theta`=1, then calculate the value of `sec theta+ cosec theta`.
View SolutionIf `3tan^2 x = 1(0^@
View SolutionWhat is the positive real root of `64x^2-1= 0`?
View SolutionThe base radii of two cylinders are in the ratio 2 : 3 and their heights are in the ratio 5:3. The ratio of their volumes is :
View SolutionIf `alpha` and `beta` be the zeros of the quadratic polynomial `2x^2+5x+1` then calculate the value of `alpha+beta+alpha beta`?
View SolutionWhat is middle value of a class interval which lies between true upper limit and true lower limit called?
View SolutionAn integer is chosen at random between 1 and 100 . Find the probability that chosen number is divisible by 10.
View SolutionIn the figure.If `("OA")/("OD")=("OC")/("OB")`, then

which pair of angle are equal?
View SolutionCheck if 0.2 is a root of the equatin `x^2-0.4 = 0`.
View SolutionIf 6 times the `6^(th)` term of the A.P is equal to 9 times the `9^(th)` term, then find its `15^(th)` term.
View SolutionFind the soluton of the following pair of equation: x-3y = 2, 3x-y = 14
View SolutionThe chord of a circle of radius 8 cm subtends a right angle at its centre. Find the length of the chord.
View SolutionFormula one Portugese Grand Prix technical team at the Algarve International Circuit are analysing last year data of drives's performance to provide valuable inference to commentators on how the drives can improve this year.
The length of time taken by 80 drives to complete a journey is given in the table below:

In which interval does the median of the distribution lie?
View SolutionGoogle maps cartography team is working on improving the scalability quality of maps when you use the app on your phones to zoom in using 4 fingers. They are using a proprietary tool called "MapMaker' to figure out scalability factors. A mathematical model is created for a type of object (below cross-section) to test its scalability on maps app.

In the diagram, `AC = 8 cm, CE = 4 cm` and the area of the triangle BEC is 4.2 sq cm. Another enlargement with centre E, maps `DeltaEBC " onto " DeltaEFA, BC = 3.6 cm`
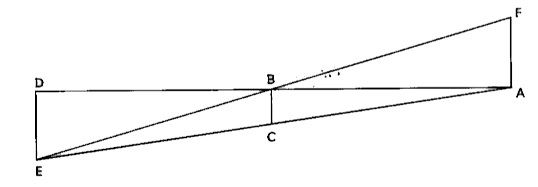
The area of `DeltaABC` is:
View SolutionFind the HCF and the LCM of 72 and 120, using prime factorisation method.
View SolutionWrite a pair of equations in variables x and y which is consistent with
(A) unique solution
(B) infinitely many solution
View SolutionWrite a pair of equations in variables x and y which is consistent with
(A) unique solution
(B) infinitely many solution
View SolutionIn an AP, if a = 1, `a_(n)`= 20 and `S_(n)` = 399, then n is equal to
View SolutionThe verticles of an equilateral triangle ABC are (0, 0), (0, y) and `(3,sqrt(3))`, then find the value of y
View SolutionIf `costheta +sin theta= sqrt(2)cos theta`, then prove that` costheta-sintheta =sqrt(2)sin theta`
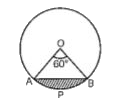
View SolutionIn the figure, chord AB subtends an angle of `60°^(@)` at the centre of the circle of radius 3.5 cm. Find the (a) length of the arc APB (b) the area of the sector AOB (C) area of the minor segment (Shaded region) (use`sqrt(3)=1.73`)
View SolutionIf `sin theta + cos theta = p` and `sec theta + "cosec"theta = q`, then prove that `q(p^(2)-1) = 2p`.
View Solution7. Let A `(4, 2)` B`(6.,5)` and C`(1.,4)`. be the vertices of `DeltaABC`. (1) The median from A meets BC at D. Find the coordinates of the point D. (2) the coordinates of the point P on AD such that `AP: PD= 2:1`. (3) Find the coordinates of points Q and R on medians BE and CF respectively such that `BQ: QE = 2 :1` and `CR :RF =2: 1`. (4) what do yo observe?
View SolutionOne card is drawn from a pack of 52 cards , each of the 52 cards being equally likely to be drawn. Find the probability that
the card drawn is black .
View SolutionOne card is drawn from a pack of 52 cards , each of the 52 cards being equally likely to be drawn. Find the probability that
the card drawn is either black or a queen .
View SolutionOne card is drawn from a pack of 52 cards , each of the 52 cards being equally likely to be drawn. Find the probability that
the card drawn is black and a queen .
View SolutionIn figure ABC and DBC are two triangles on the same base BC. If AD intersects BC at O, show that `(a r(A B C))/(a r(D B C))=(A O)/(D O)`.
View SolutionProve that the line segments joining the mid-points of the sides of a triangle from four triangles, each of which is similar to the original triangle.
View SolutionVerify that 2,1,1 are the zeros of the polynomial `x^(3)-4x^(2)+5x-2`. Also, verify the relationship between the zeroes and the coefficients
View SolutionIf `x=2^(2)xx3^(3)xx7^(2),y=2^(3)xx3^(2)xx5xx7`, then find HCF (x,y)
View SolutionWhat is the HCF of the smallest prime number and the smallest composite number?
View SolutionIF `alpha,beta` are the zeroes of the polynomial `5x^2-7x+2` then the sum of their reciprocal is:
View SolutionIf the lines represened by `3x+2py=2` and `2x+5y+1=0` are parallel, then find the value of p.
View SolutionFind the `10^(th)` term form the end of the A.P . 4 , 9, 14,`…..`,254 .
View SolutionSolve for x and y `y,x+y=3` and `7x+6y=2`.
View SolutionFind a quadratic polynomial whose zeroes are `-3 and 5.`
View SolutionFor what values of 'a' does the quadratic equation `x^(2)-ax+1=0` not have real roots?
View SolutionIf p and q are the roots of the quadratic equation `x^(2)+px-q=0`, then find the values of p and q.
View SolutionWhich term of the AP 21, 42, 63, 84,.. Is 210?
View SolutionFind distance between the points (0, 5) and (-5, 0)
View SolutionWhat is the distance between two parallel tangents to a circle of radius 5 cm?
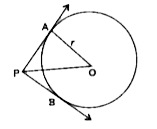
View SolutionIn the figure, `angleAPB=90^(@)`. Find the length of OP.
View Solution`DeltaABC ~ Delta DEF` such that DE = 3 cm , EF = 2 cm , DF = `2.5` and BC = 4 cm . Find the perimeter of `DeltaABC.`
View SolutionIf `"cosec"theta - cot theta = 1/3`, then the value of `"cosec"theta + cot theta` is:
View SolutionIf `DeltaABC` is right angled at C, then the value of cos(A+B) is
View SolutionA wire is in the shape of a circle of radius 21 cm. It is bent to form a square. The side of the square is : `(pi = (22)/(7))`
View SolutionIf the area of three adjacent faces of a cuboid are X, Y and Z respectively, then find the volume of cuboid.
View SolutionA crane stands on a level ground. It is represented by a tower ABCD, of height 11 m and a jib BR. The ib is of length 20 m and can rotate in a vertical plane about B.A vertical cable, RS, carries a load S. the diagram shows current position of the jib, cable and load.
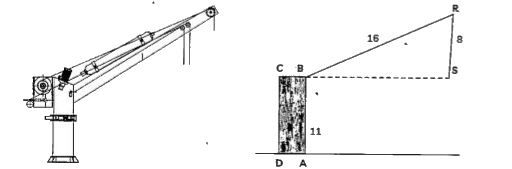
The length BS is
View SolutionA crane stands on a level ground. It is represented by a tower ABCD of height 11 m and BR. The ib is of length 20 m and can rotate in a vertical plane about B.A vertical cable, RS, carries a load S. the diagram shows current position of the jib, cable and load.
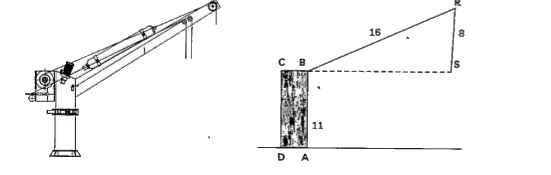
The angle that the jib, BR, makes with the horizontal, is
View SolutionA crane stands on a level ground. It is represented by a tower ABCD, of height 11 m and a jib BR. The Jib is of length 20 m and can rotate in a vertical plane about B.A vertical cable, RS, carries a load S. the diagram shows current position of the jib, cable and load.
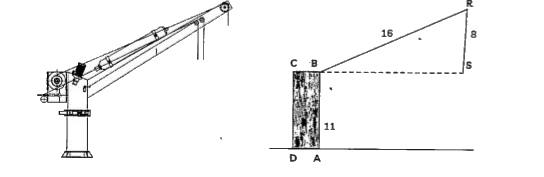
The measure of the angles BRS, is
View SolutionNITI aayog has tasked their statistical officer to create a model for farmers to be able to predict their produce output based on various factors.
To test the model out, the officer picked a local farmer who sells apples to check various factors like weight, bad apples, half-cooked, green vs red etc.
A box containing 250 apples was opened and each apple was weighed.
The distribution of the masses of the apples is given in the following table:

The value of p is
View SolutionNITI aayog has tasked their statistical officer to create a model for farmers to be able to predict their produce output based on various factors.
To test the model out, the officer picked a local farmer who sells apples to check various factors like weight, bad apples, half-cooked, green vs red etc.
A box containing 250 apples was opened and each apple was weighed.
The distribution of the masses of the apples is given in the following table:

The lower limit of the modal class is
View SolutionShow that `3+sqrt(5)` is an irrational number, assuming that `sqrt(5)` is an irrational number.
View SolutionWithout actually performing the long divison, find if `(987)/(10500)` will have terminating or non-terminating (repeating) decimal expansion. Give reasons for your answer
View SolutionProve that the points `(a, b + c), (b, c + a) and (c, a + b)` are collinear.
View SolutionTwo opposite vertices of a square are `(-1,\ 2)` and `(3,\ 2)` . Find the coordinates of other two vertices.
View SolutionIn the figure, OABC is a rhombus, where O is the origin.
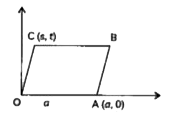
Write down the coordinates of B in terms of a, s and t.
View SolutionABC is an isosceles triangle in which AB = AC. Prove that the tangent to the circum-circle at A is parallel to BC.
View SolutionIn an acute angled `DeltaABC,sec(B+C-A)=2` and `tan(C+A-B)=(1)/(sqrt3)`. Find the three angles of `DeltaABC.`
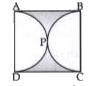
View SolutionFind the area of the shaded region in the given figure, if ABCD is a square of side 14 cm and APD and BPC are semicircles.
View SolutionShow that `12^n` cannot end with the digits `0` or `5` for any natural number `n`
View SolutionWhich term of the AP `-2,-7,-12, …` will be `-77` ? Find the sum of this AP upto the term `-77`.
View Solution5 books and 7 pens together cost Rs 434, whereas 7 books and 5 pens together cost Rs 550, find the total cost of 1 book and 2 pens.
View Solution`(tanA)(1+secA) - (tanA)/(1-secA) = 2cosecA`
View SolutionIf `sintheta=(12)/(13)`, find the value of `(sin^(2)theta-cos^(2)theta)/(2sinthetacostheta)-(1)/(tan^(2)theta)`.
View SolutionIf the zeros of the polynomial `f(x)=ax^3+3bx^2+3cx+d` are in A.P. then show that `2b^3-3abc+a^2d=0`
View SolutionFrom the top of a tower h m high, angles of depression of two objects, which are in line with the foot of the tower are `alpha` and `beta(betagtalpha)`. Find the distance between the two objects.
View SolutionTwo tangents `T P` and `T Q` are drawn to a circle with centre `O` from an external point `T` . Prove that `/_P T Q=2/_O P Q` .
View SolutionProve that the area of the semicircle drawn on the hypotenuse of a right angled triangle is equal to the sum of the areas of the semicircles drawn on the other two sides of the triangle
View Solution
EXERCISE questions and complete solutions for chapter SAMPLE PAPER 11 of AGRAWAL PUBLICATION of Class 10