Complete Solutions to EXERCISE 6.6 of chapter TRIANGLES of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | TRIANGLES |
| KUMAR PRAKASHAN | EXERCISE 6.6 |
| Number Of Questions | 15 |
| Academic Year | 2023-24 |
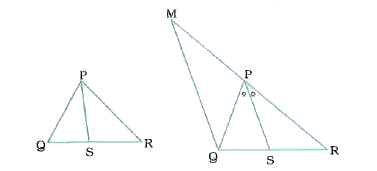
In the given figure, PS in the bisector of `/_QPR " of "DeltaPQR`. Prove that `(QS)/(SR)= (PQ)/(PR)`.
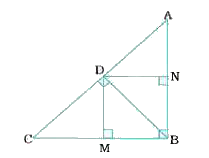
View SolutionIn the given figure, D is a point on hypotenuse AC of `triangleABC`, such that `BD bot AC, DM bot BC" and "DN bot AB`. Prove that :
`DM^(2)= DN*MC`
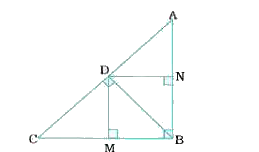
View SolutionIn the given figure, D is a point on hypotenuse AC of `triangleABC`, such that `BD bot AC, DM bot BC" and "DN bot AB`. Prove that :
`DN^(2)= DM*AN`
View SolutionIn the given figure, ABC is a Deltain which `/_ABC gt 90^(@)" and "AD bot CB` produced. Prove that `AC^(2)= AB^(2)+BC^(2)+2BC*BD`
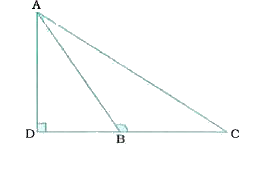
View SolutionIn the given figure, ABC is a Deltain which `/_ABC lt 90^(@)" and "AD bot BC`. Prove that `AC^(2)= AB^(2)+BC^(2)-2BC*BD`
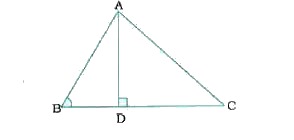
View SolutionIn the given figure, AD is a median of a DeltaABC and `AM bot BC`. Prove that :
`AC^(2)= AD^(2)+BC*DM+((BC)/(2))^(2)`
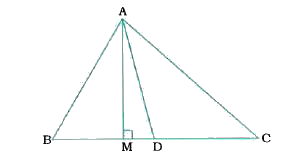
View SolutionIn the given figure, AD is a median of a DeltaABC and `AM bot BC`. Prove that :
`AB^(2)= AD^(2)-BC*DM+((BC)/(2))^(2)`
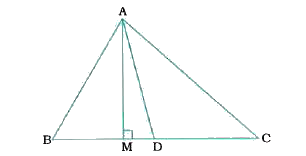
View SolutionIn the given figure, AD is a median of a DeltaABC and `AM bot BC`. Prove that :
`AC^(2)+AB^(2)= 2AD^(2)+(1)/(2) BC^(2)`
View SolutionProve that the sum of the squares of the diagonals of a parallelogram is equal to the sum of the squares of its sides.
View SolutionIn the given figure, two chords AB and CD intersect each other at the point P. Prove that
`DeltaAPC ~ DeltaDPB`

View SolutionIn the given figure, two chords AB and CD intersect each other at the point P. Prove that
`AP*PB= CP*DP`

View SolutionIn the given figure, two chords AB and CD of a circle intersect each other at the point P (when produced) outside the circle. Prove that
`DeltaPAC ~ DeltaPDB`
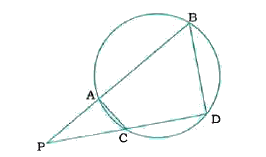
View SolutionIn the given figure, two chords AB and CD of a circle intersect each other at the point P (when produced) outside the circle. Prove that
`PA*PB= PC*PD`

View SolutionIn the given figure, D is a point on side BC of `DeltaABC` such that `(BD)/(CD)=(AB)/(AC)`. Prove that AD is the bisector of `/_BAC`.
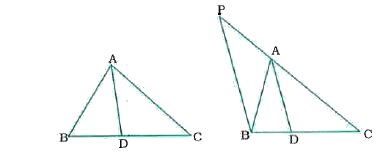
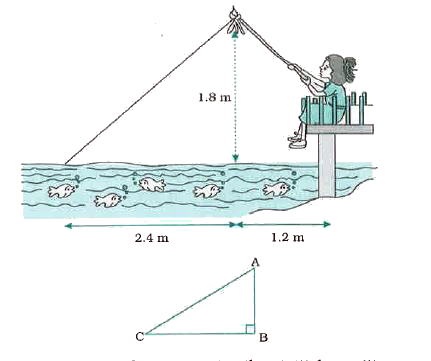
View SolutionNazima is fly fishing in a stream. The tip of her fishing rod is `1.8 m` above the surface of the water and the fly at the end of the string rests on the water `3.6 m` away from her and `2.4 m` from a point directly under the tip of the rod. Assuming that her string (from the tip of her rod to the fly) is taut, how much string does she have out (see the given figure)? If she pulls in the string at the rate of 5 cm per second, what will be the horizontal distance of the fly from her after 12 seconds?
View Solution
EXERCISE 6.6 questions and complete solutions for chapter TRIANGLES of KUMAR PRAKASHAN of Class 10