Complete Solutions to Solved Example of chapter LINEAR EQUATIONS IN TWO VARIABLES of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | LINEAR EQUATIONS IN TWO VARIABLES |
| NAGEEN PRAKASHAN | Solved Example |
| Number Of Questions | 77 |
| Academic Year | 2023-24 |
Solve graphically the system of linear equations
x + y = 10 and x - y = 4.
View SolutionShow graphically the system of linear equations
x + 2y = 3 and 4x + 3y = 2.
View SolutionShow graphically that the system of equations
2x + 4y = 10 and 3x + 6y = 12 has no solution.
View SolutionShow graphically that the system of equations `3x-y=2` `9x-3y=6` has infinitely many solutions.
View SolutionDraw the graphs of the equations `x y+1=0` and `3x+2y 12=0`. Determine the coordinates of the vertices of the triangle formed by these lines and the xaxis, and shade the triangular region.
View SolutionSolve x + y = 7 and 3x - 2y = 11.
View SolutionSolve 15x - 8y = 29 and 17x + 12y = 75.
View SolutionSolve 3x - 4y = 20 and x + 2y = 5.
View SolutionSolve the following system of equations by using the method of elimination by equating the coefficients: `8x+5y=9,\ \ \ 3x+2y=4`
View SolutionSolve 3x - y = 23 and `(x)/(3) + (y)/(4)` = 4.
View SolutionSolve: `4x+6/y=15`
`6x-8/y=14`
and hence find p if `y=p x-2`
View SolutionSolve `(7)/(x) + (8)/(y) = 2` and `(2)/(x) + (12)/(y) = 20`.
View Solution`1/(2x)-1/y=-1 , 1/x+1/(2y)=8`
View SolutionSolve `(20)/(x + y) + (3)/(x - y) = 7` and `(8)/(x - y)- (15)/(x + y) = 5`.
View Solution(i) Solve 8x - 3y = 5xy and 6x - 5y = - 2xy. How many solutions, this system of equations has?
(ii) Solve for x and y, by reducing the following equations in a pair of linear equations :
2x + 3y = 5xy and 3x - 2y = xy.
View Solution`Sol v e :1/(2(2x+3y))+(12)/(7(3x-2y))=1/2` `7/(2x+3y)+4/(3x-2y)=2` where `2x+3y!=0a n d3x-2y!=0.`
View SolutionSolve 41x + 53y = 135 and 53x + 41y = 147.
View SolutionSolve for 'x' and 'y': `(a -b) x + (a + b) y =a^2 - b^2 - 2ab (a + b) (x + y) = a ^2+ b^2`
View SolutionSolve the equations :
`(x - 4) (y - 4) = 16`
` (y - 6) (z - 6) = 36`
`(z - 8) (x - 8) = 64`.
View SolutionSolve the following equations for `(x - 3)^(2)` and `(y + 2)^(2)` :
`2x^(2) + y^(2) - 12x + 4y + 16 = 0` and `3x^(2) - 2y^(2) - 18x - 8y + 3 = 0`.
View SolutionSolve for x and y :
`2^(y-x) (x + y) = 1` and `(x + y)^(x - y) = 2`.
View SolutionSolve :
`(3x + 2y - 7)^(2) + (5x + 7y - 13)^(2) = 0`, for real values of x and y.
View SolutionSolve the following system of equations by using the method of cross multiplication
2x + 3y = 7 and 6x + 5y = 11.
View SolutionSolve the following system of equations by cross multiplication method
3x - 5y = 20 and 7x + 2y = 17.
View SolutionSolve the following system of equations
bx + ay = 2ab and `ax - by = a^(2) - b^(2)`.
View SolutionSolve : `a(x+y)+b(x-y)=a^2-a b+b^2` `a(x+y)-b(x-y)=a^2+a b+b^2`
View SolutionShow that the following system of equations have unique solution :
`{:((i),7x-2y=3,(ii),3x+y=17,(iii),2x+5y=17,),(,22x-3y=16,,8x+11y = 37,,5x+3y=14,):}`
and also solve the system of equations in each case.
View SolutionFind the value of k for which the system of equations 2x + ky = 1 and 3x - 5y = 7 has a unique solution.
View SolutionFor what value of `k` , will the system of equations `x+2y=5,\ \ \ \ 3x+k y-15=0` has (i) a unique solution? (ii) no solution
View SolutionFor what value(s) of `alpha` will the system of linear equations `alphax + 3y = alpha - 3` and `12x + alpha y = alpha` has a unique solution?
View SolutionFor the following system of equations determine the value of `k` for which the given system has infinitely many solutions: `5x+2y=k ,\ \ \ \ 10 x+4y=3`
View SolutionFind the values of `p` and `q` for which the following system of equations has infinite number of solutions: `2x+3y=7,\ \ \ \ (p+q)x+(2p-q)y=21`
View Solutionfor what values of `k` will the following pairs of linear equations have infinitely many solutions `kx+3y-(k-3)=0` and `12x+ky-k=0`
View SolutionWhen a system of linear equations said to be consistent. For what value of k will the equations 3x + 4y + 2 = 0 and 9x + 12y + k = 0 are dependent ?
View SolutionFor what value(s) of k, the pair of linear equations -2x + 5y = 0 and kx + 3y = 0 has a non-zero solutions?
View SolutionThe sum of two numbers is 80. If the larger number exceeds four times the smaller one by 5. Find the numbers.
View SolutionThe sum of two numbers is 18. The sum of their reciprocals is . Find the numbers.
View SolutionThe ages of two friends Ani and Biju differ by 3 years. Anis father Dharam is twice as old as Ani and Biju as twice as old as his sister Cathy. The ages of Cathy and Dharam differ by 30 years. Find the ages of Ani and Biju.
View SolutionFive years age a man was seven times as old as his son. Five years hence, the father will be three times as old as his son. Find their present ages.
View SolutionThe total of the ages of father and son is 55 years. If the father was to live till his son's age equals his present age, the total of their ages would be 93 years. Find their present ages.
View SolutionThe sum of the two-digit number is 9 The number formed by interchanging the order of the digits 27 more than the given number.Find the number.
View SolutionIf 2 is added to the numerator of a fraction, it reduces to `1//2` and if 1 is subtracted from the denominator, it reduces to `1//3` . Find the fraction.
View Solution5 books and 7 pens together cost Rs 79 whereas 7 books and 5 pens together cost Rs 77. Find the total cost of 1 book and 2 pens.
View SolutionA fruit seller has certain number of oranges. He divides them into two lots A and B. He sells the lot at 3 oranges for Rs. 2 and the lot B at 1 oranges for Rs. 1. Thus he gets Rs. 400, if he had sold the first lot at 1 oranges for Rs. 1 and the second lot B at 5 oranges for Rs. 4, he would have got Rs. 460. Find the total number of oranges.
View SolutionOn selling a tea-set 5% loss and a lemon-set at 15% gain, a crockery seller gains Rs. 7. If he sells the tea-set at 5% gain and the lemon-set at 10% gain, he gains Rs. 13. Find the actual price of the tea-set and the lemon-set.
View SolutionA man sold a chair and a table together for 760 thereby making a profit of 25% on the chair and 10% on the table. By selling them together for 767.50 he would have made a profit of 10% on the chair and 25%, on the table. Find the cost price of each.
View SolutionIn a `DeltaABC, angleC = 3 angleB = 2(angleA + angleB)` find the three angles.
View SolutionThe area of a rectangle gets reduced by 9 square units if its length is reduced by 5 units and the breadth is increased by 3 units. If we increase the length by 3 units and breadth by 2 units, the area is increased by 67 square units. Find the length and breadth of the rectangle.
View SolutionA lady has 25 paise and 50 paise coins in her purse. If in all she has 40 coins and value of her money is Rs. 12.75, how many coins of each type does she have?
View SolutionA man when asked ''how many hens and buffaloes he has ?''. He replied that they have 78 eyes and 110 legs in all. Find the number of hens and buffaloes separately.
View Solution2 men and 7 boys can do a piece of work in 4 days. The same work is done in 3 days by 4 men and 4 boys. How long would it take one man and one boy to do it?
View SolutionWater is pouring into a tank at a constant rate. when the tank is full, 10 pumps of equal capacity empty the tank in 12 hrs, while 15 pumps of the same capacity empty the tank in 6 hrs. The time which 25 pumps of the same capacity take to empty the tank, if the tank in initially full, will be :
View SolutionSome students planned a picnic. The budget for food was Rs. 500. But 5 of them failed to go and thus the cost of food for each member increased by Rs. 5. How many students attended the picnic?
View SolutionA plane left 30 minutes late than its scheduled time and in order to reach the destination1500 km away in time, it had to increase the speed by 250 km/h from the usual speed.Find its usual speed.
View SolutionPlaces A and B are 100 km apart on a highway. One car starts from A and another from b at the same time. If the cars travel in the same direction at different speeds, they meet in 5 hours. If they travel towards each other, they meet in 1 hour. What are the speeds of the two cars
View Solution5. After covering a distance of 30 km with a uniform speed there is some defect in train engine and therefore, its speed is reduced to 4/5 of its original speed. Consequently, train reaches its destination late by 45 minutes. Had it happened after covering 18 km more, reached 9 minutes earlier. Find the speed of the train and the distance of covered.
View SolutionA boat goes 30 km upstream and 44 km downstream in 10 hours. In 13 hours, it can go 40 km upstream and 55 km downstream. Determine the speed of the stream and that of the boat in still water.
View SolutionA boat which travels at the rate of 10.5 km/hr downstream takes 3 times as long time to go to a certain distance up a river as to go the same distance down. Find the rate at which stream flows.
View SolutionHalf the perimeter of a rectangular garden, whose length is 4 m more than its width, is 36 m. Find the dimensions of the garden by graphical method.
View SolutionSolve `2x+3y=11` and `2x-4y=-24` and hence find the value of 'm' for which `y=mx+3`.
View Solution(i) For which values of a and b does the following pair of linear equations have an infinite number of solutions?
2x + 3y = 7
(a - b)x + (a + b)y = 3a + b - 2
(ii) For which value of k will the following pair of linear equations have no solution?
3x + y = 1
(2k - 1)x + (k - 1)y = 2k + 1
View SolutionFormulate the following problems as a pair of equations, and hence find their solutions: (i) Ritu can row downstream 20 km in 2 hours, and upstream 4 km in 2 hours. Find her speed of rowing in still water and the speed of the current. (ii) 2 wome
View SolutionOne says, "Give me a hundred, friend ! I shall then become twice as rich as you". The other replies. 'If you give me ten, I shall be six times as rich as you". Tell me what is the amount of their (respective) capital? [From the Bijaganita of B
View SolutionThe students of a class are made to stand in rows. If 3 students are extra in a row, there would be 1 row less. If 3 students are less in a row there would be 2 rows more. Find the number of students in the class.
View SolutionTwo straight paths are represented by the equations ` x - 3y = 2` and `-2x + 6y = 5`. Check whether the paths cross each other or not.
View SolutionFind the values of x and y in the following rectangle :
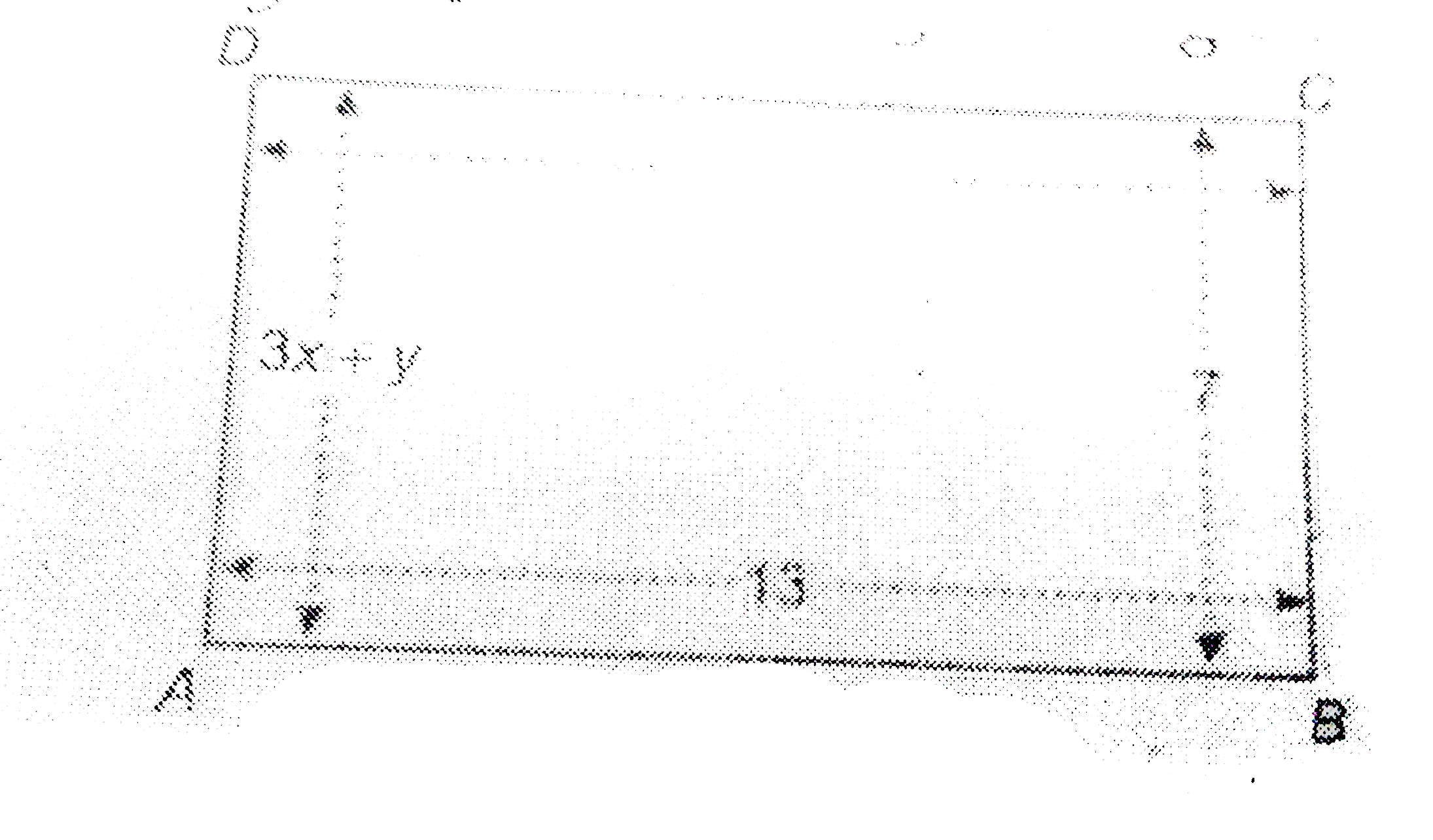
We know that the opposite sides of a rectangle are equal
`therefore` 3x + y = 7 …(1)
x + 3y = 13 …(2)
9x + 3y = 21 [from (1)]
`{:(" x + 3y = 13"),( " "ul"- - -"),("On subtracting, 8x =8"):}`
x = 1
Now, from (1), 3 (1) + y = 7
implies y = 7 - 3
implies y = 4
Hence, the required values of x and y are 1 and 4 respectively.
View SolutionThe age of the father is twice the sum of the ages of his two children. After 20 yr, his age will be equal to the sum of the ages of his children. Find the age of the father.
View SolutionTwo numbers are in the ratio 5 : 6. If 8 is subtracted from each of the numbers, the ratio becomes 4 : 5, then find the numbers.
View SolutionA shopkeeper gives books on rent for reading. She takes a fixed charge for the first two days and an additional charge for each day thereafter. Latika paid Rs. 22 for a book kept for six days, while Anand paid Rs. 16 for the book kept for four days. Find the fixed charges and the charge for each extra day.
View SolutionIn a competitive examination, 1 mark is awarded for each correct answer while 1/2 mark is deducted for every wrong answer. Jayanti answered 120 questions and got 90 marks. How many questions did she answer correctly?
View SolutionA shopkeeper sells a saree at 8% profit and a sweater at 10% discount, thereby, getting a sum Rs 1008. If she had sold the saree at 10% profit and the sweater at 8% discount, she would have got Rs 1028 then find the cost of the saree and the list price (price before discount) of the sweater.
View SolutionThe pair of equations y = 0 and y = 7 has :
(a) one solution (b) two solutions
(c ) infinitely many solutions (d) no solution
View SolutionIf x = a and y = b is the solution of the equations x - y =2 and x + y = 4 , then the values of a and b are, respectively
View SolutionFor the pair of equations `lambdax + 3y + 7 = 0` and ` 2x + 6y - 14 = 0`. To have infinitely many solutions, the value of `lambda` should be 1 . Is the statement true ? Give reasons.
View SolutionFor which values of a and b will the following pair of linear equations has infinitely many solutions ?
`x + 2y = 1`
` (a-b) x + (a + b ) y = a + b = -2`
View SolutionIf (x+1) is a factor of `2x^(3)+ax^(2)+2bx+1`, then find the value of a and b given that 2a-3b=4.
View SolutionA person can row a boat at the rate of 5 km/hour in still water. He takes thrice as much time in going 40 km upstream as in going 40 km downstream. Find the speed of the stream.
View Solution
Solved Example questions and complete solutions for chapter LINEAR EQUATIONS IN TWO VARIABLES of NAGEEN MATHS (HINGLISH) of Class 10