Complete Solutions to All Questions of chapter AREAS RELATED TO CIRCLES of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | AREAS RELATED TO CIRCLES |
| Mathematics | All Questions |
| Number Of Questions | 100 |
| Academic Year | 2023-24 |
A circular pond is on diameter 17.5m. It is surrounded by a 2m wide path. Find the cost of constructing the path at the rate of Rs. 25 per square metre...
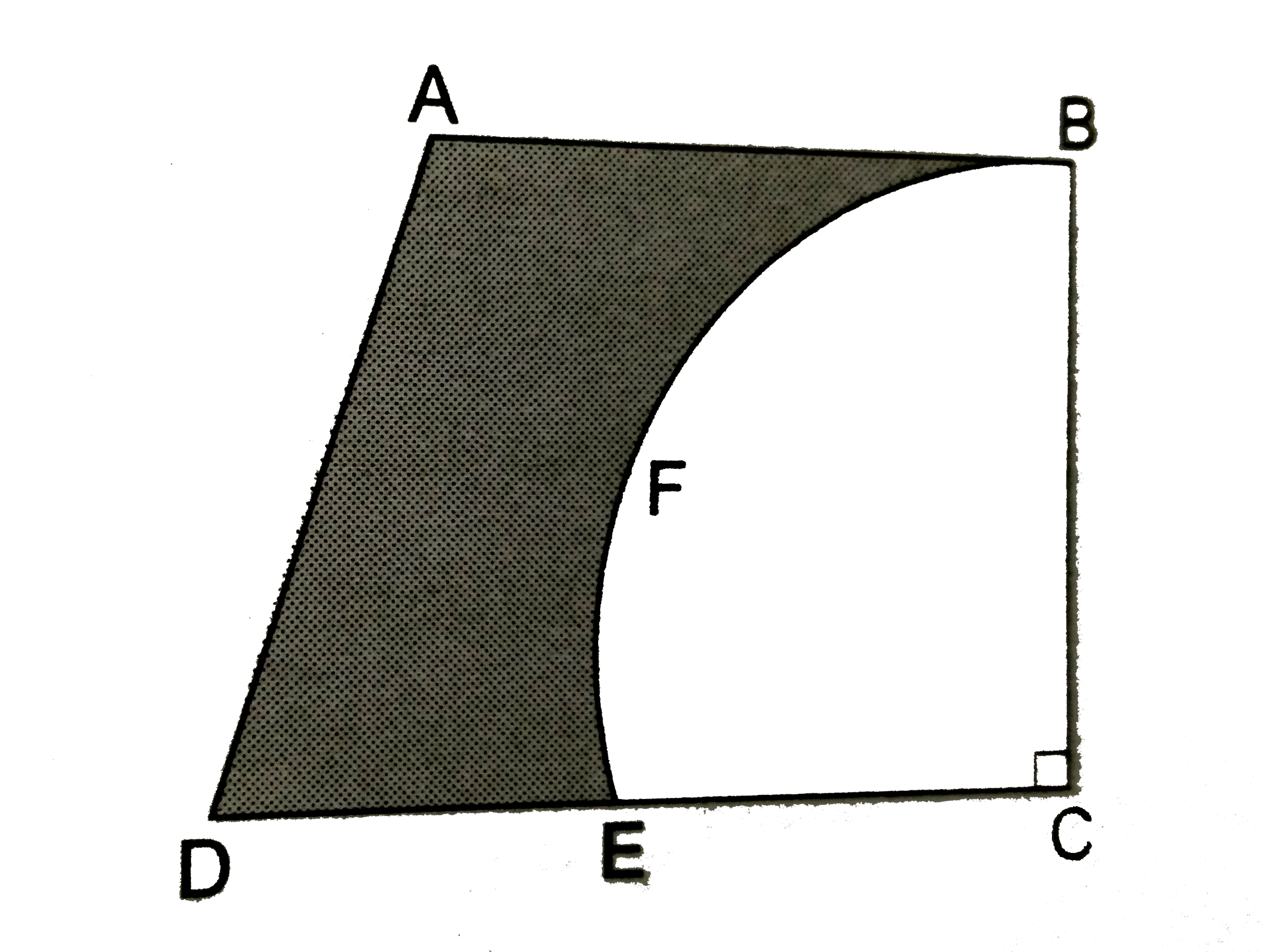
View SolutionFrom a thin metallic piece in the shaped of a trapezium ABCD in which `AB||CD` and `/_BCD=90^(@)`,a quarter circle BFEC is removed. Given, `AB=BC=3.5cm` and `DE=2cm` calculate the area of remaining (shaded) part of metal sheet.
View SolutionCombination of quadrilateral and circle : In Figure; ABCD is a trapezium with AB||DC ; AB = 18cm; DC = 32cm and the distance between AB and DC is 14 cm. Circles of Equal radii 7 cm with centres A;B;C and D have been drawn. Then; find the area of the shaded region of the figure.
View SolutionIn Figure, ABC is an equilateral triangle of side 8cm. A, B and C are the centres of circular arcs of radius 4cm. Find the area of the shaded region correct upto 2 decimal places.
View SolutionIn figure, find the area of the shaded region [Use `pi=3.14`]
View SolutionFind to the three places of decimal the radius of the circle whose area is the sum of the areas of two triangles whose sides are 35, 53, 66 and 33, 56, 65 measured in centimetres.
View SolutionFind the area of a shaded region in the figure, where a circular arc of radius 7cm has been drawn with vertex A of an equilateral triangle ABC of side 14cm as centre.
View SolutionFigure, shows a kite in which BCD is the shape of a quadrant of ac ircle of radius 42cm. ABCD is a square and `` CEF is an isosceles right angled triangle whose equal side are 6cm long. Find the area of the shaded region.
View SolutionA circular grassy plot of land, 42m in diameter, has a path 3.5m wide running round it on the outside. Find the cost of gravelling the path at Rs. 4 per square metre.
View SolutionFrom each of the two opposite corners of a square of side 8cm, a quadrant of a circle of radius 1.4 cm is cut. Another circle of diameter 4.2cm is also cut from the centre as shown in figure. Find the area of the remaining (shaded) portion of the square.
View SolutionThe diagram shown two arcs, A and B. Arc A is part of the circle with centre `O` and radius `O P` . Arc `B` is part of the circle with centre `M` and radius `P M` , where is the mid-point of PQ. Show that the area enclosed by the two arcs is equal to `25(sqrt(3)-pi/6)c m^2`
View SolutionThe copper wire, when bent in the form of a square, encloses an area of 484 `c m^2` . If the same wire is bent in the form of a circle, find the area enclosed by it.
View SolutionThe inner circumference if a circular track is 220m. The track is 7m wide everywhere. Calculate the cost of putting up a fence along the outer circle at the rate of Rs. 2 per metre. `(U s epi(22)/7)`
View SolutionA boy is cycling such that the wheel of the cycle are making 140 revolutions per minute. If the diameter of the wheel is 60cm, calculate the speed per hour with which the boy is cycling.
View SolutionThe diameter of the driving wheel of a bus is 140cm. How many revolutions per minute must the wheel make in order to keep a speed of 66km per hour?
View SolutionFind the area of a quadrant of a circle whose circumference is 22cm.
View SolutionIf the perimeter of a semi-circular protractor is 108cm, find the diameter of the protractor.
View SolutionTwo circles touch externally. The sum of their areas is 130 `pis qdotc mdot` and the distance between their centres is 14cm. Find the radii of the circles.
View SolutionIn a circle of radius 6cm, a chord of length 10cm makes an angle of `110^0` at the centre of the circle. Find: the circumference of the circle the area of the circle the length of the arc AB, the area of the sector OAB
View SolutionFigure shows a sector of a circle of radius r containing an angle `theta^@` The area of the sector is `A cm^2` and the perimeter is 50cm. Prove that `theta = (360)/pi ((25)/r-1)` and `A=25r -r^2`
View SolutionIf the equation `x^3 + ax^2+ bx+216=0` has three roots in GP then b/a is equal to
View SolutionA sector of `56^0` cut out from a circle contains area `4. 4 c m^2dot` Find the radius of the circle
View SolutionA horse is tied to a pole with 28m long string. Find the area where the horse can graze. `(T a k epi(22)/7)`
View SolutionIf a square is inscribed in a circle, find the ratio of the areas of the circle and the square.
View SolutionThe length of the minute hand of a clock is 14cm. Find the area swept by the minute hand in 5 minutes.
View SolutionIn a circle of radius 21cm, an arc subtends an angle of `60^0` at the centre. Find (i) the length of the arc (ii) area of the sector formed by the arc. `(U s epi(22)/7)`
View SolutionIn an equilateral triangle of side 24cm, a circle is inscribed touching its sides. Find the area of the remaining portion of the triangle `(T a k esqrt(3)=1. 732)`
View SolutionAn athletic track 14 m wide consists of two straight sections 120 m long joining and semicircular ends inner diameter 35 cm . find area
View SolutionThe area of an equilateral triangle is `49sqrt(3)c m^2` . Taking each angular point as shown in Figure. Find the area of the triangle not included in the circle.
View SolutionIn Figure, two circular flower beds have been shown on two sides of a square lawn ABCD of side 56m. If the centre of each circular flower bed is the point of intersection of the diagonals of the square lawn, find the sum of the areas of the lawns and the flower beds.
View SolutionABCD is a field in the shape of a trapezium. `AB || DC` and `/_A B C=90^@,` `/_D A B=60^@`. Four sectors are formed with centres `A ,B ,C` and `D`. The radius of each sector is 17.5 m. Find the total area of the four sectors.
View SolutionIn Figure, a crescent is formed by two circles which touch at A. C is the centre of the larger circle. The width of the crescent at BD is 9cm and at EF it is 5cm. Find (i) the radii of two circles (ii) the area of the shaded region.
View SolutionIn the given figure,three circles of radius 2 cm touch one another externally.These circles are circumscribed by a circle of radius R cm.Find the approximate value of R.
View SolutionFour equal circles, each of radius 5cm, touch each other. Find the area included between them.
View SolutionFour cows are tethered at four corners of a square plot of side 50m, so that they just cannot reach on another. What area will be left ungrazed?
View SolutionThe inside perimeter of a running track (shown in figure) is 400m. The length of each of the straight portion is 90m and the ends are semi-circles. If the track is everywhere 14m wide, find the area of the track. Also find the length of the outer running track.
View SolutionIn the figure below, the square ABCD is made up of two parts, X and Y.The part X, is formed by a semicircle and line AB. The perimeter of X is 36 cm and perimeter of the shaded part Y is 64 cm. a. Find the perimeter of square ABCD b. Find the area of shaded region Y
View SolutionFigure, ABCD is a square of side 2a. Find the ratio between the circumferences and the areas of the incircle and the circum-circle of the square.
View SolutionA bucket is raised from a well by means of a rope which is wound round a wheel of diameter 77 cm. Given that the bucket ascends in 1 minute 28 seconds with a uniform speed of 1.1 m/sec, calculate the number of complete revolutions the wheel makes in raising the bucket.
View SolutionA chord 10 cm long is drawn in the circle whose radius is `5 sqrt2` cm. Find the area of both the segments.
View SolutionIn Figure, ABC is a right-angled triangle, `/_B=90^0,A B=28 c m` and `B C=21 c mdot` With AC as diameter a semicircle is drawn and with BC as radius a quarter circle is drawn. Find the area of the shaded region correct to two decimal places.
View SolutionIf Figure, there are three semicircles, A, B and C having diameter 3cm each, and another semicircle E having a circle D with diameter 4.5cm are shown. Calculate: the area of the shaded region. the cost of painting the shaded region at the rate of 25 paise per `c m^2` , to the nearest rupee.
View SolutionThe inner and outer diameters of ring 1 of a dartboard are 32cm and 34cm respectively and those of rings II are 19cm and 21cm respectively. What is the total area of these two rings?
View SolutionIn Figure AB = 36 cm and M is mid-point of AB. Semi-circles are drawn on AB, AM and MB as diameters. A circle with centre C touches all the three circles. Find the area of the shaded region.
View SolutionA drain cover is made from a square metal plate of side 40cm having 441 holes of diameter 1cm each drilled in it. Find the area of the remaining square plate.
View SolutionA chord AB of a circle of radius 10cm makes a right angle at the centre of the circle. Find the area of the major and minor segments (Take `pi=3. 14`).
View SolutionA chord AB of a circle of radius 10cm subtends a right angle at the centre. Find: area of the minor sector area of the minor segment area of the major sector area of the major segment
View SolutionA pendulum swing through an angle of `30^@` and describes an arc 8.8 cm in length. Find the length of the pendulum.
View SolutionIn Figure, there are shown sectors of two concentric circles of radii 7cm and 3.5cm. Find the area of the shaded region. (Use `pi=(22)/7`).
View SolutionA car travels 1 kilometre distance in which each wheel makes 450 complete revolutions. Find the radius of the its wheels.
View SolutionIn `DeltaABC` with fixed length of `BC` , the internal bisector of angle C meets the side `AB` at `D` and meet the circumcircle at `E.` The maximum value of `CD xx DE` is
View SolutionAn elastic belt is placed round the rim of a pulled of radius 5cm. One point on the belt is pulled directly away from the centre `O` of the pulley until it is at P, 10cm from O. Find the length of the belt that is in contact with the rim of the pulley. Also, find the shaded area.
View SolutionThe short and long hands of a clock are 4cm and 6cm long respectively. Find the sum of distances travelled by their tips in 2 days. `( pi=(22)/7)`
View SolutionThe perimeter of a sector of a circle of radius 5.2cm is 16.4cm. Find the area of the sector.
View SolutionThe length of minute hand of a clock is 14cm. Find the area swept by the minute hand in one minute. `(U s epi(22)/7)`
View SolutionA chord AB of a circle of radius 10cm subtends a right angle at the centre. Find: area of the minor sector area of the minor segment area of the major sector area of the major segment
View Solution`A B` is the diameter of a circle, centre `OdotC` is a point on the circumference such that `/_C O B=thetadot` The area of the minor segment cut off by AC is equal to twice the area of the sector BOC. Prove that `sintheta/2costheta/2=pi(1/2-theta/(120))`
View SolutionIn a circle with centre `O` and radius 5cm, AB is a chord of length `5sqrt(3)c m` . Find the area of sector `A O Bdot`
View SolutionAn arc of a circle is a length 5`pic m` and the sector it bounds has an area of `20pic m^2` . Find the radius of the circle.
View SolutionA horse is placed for grazing inside a rectangular field 70m by 52m and is tethered to one corner by a rope 21m long. On how much area can it graze?
View SolutionCircle inside a Circle : PQRS is a diameter of a circle of radius 6 cm. The length PQ;QR and RS are equal. Semi circles are drawn on PQ and QS as diameters as shown in Figure. Find the perimeter and area of shaded Region.
View SolutionIn figure, find the area of the shaded region `[U s epi=3. 14]`
View SolutionA paper is in the form of a rectangle `A B C D` in which `A B=20 c m` and `B C=14 c mdot` A semi-circular portion with BC as diameter is cut off. Find the area of a remaining part.
View SolutionFind the circumference and area of a circle of radius 8.4 cm.
View SolutionFind the area of a circle whose circumference is 22 cm.
View SolutionThe circumference of a circle exceeds the diameter by 16.8 cm. Find the radius of the circle.
View SolutionTwo circles touch internally. The sum of their areas is `116pi\ c m^2` and distance between their centres is 6 cm. Find the radii of the circles.
View SolutionA wire is looped in the form of a circle of radius 28 cm. It is re-bent into a square form. Determine the length of the side of the square.
View SolutionA race track is in the form of a ring whose inner circumference is 352 m, and the outer circumference is 396 m. Find the width of the track.
View SolutionA bicycle wheel makes 5000 revolutions in moving 11 km. Find the diameter of the wheel.
View SolutionThe diameter of a wheel is 84 cm. How many revolution will it make to cover 792 m ?
View SolutionA car has wheels which are 80 cm in diameter. How many complete revolutions does each wheel make in 10 minutes when the car is travelling at a speed of 66 km per hour?
View SolutionThe cost of fencing a circular field at the rate Rs 24 per metre is Rs 5280. The field is to be ploughed at the rate of Rs `0. 50\ p e r\ m^2` . Find the cost of ploughing the field. `(T a k e\ pi=22//7)`
View SolutionFig. 15.6, depicts an archery target marked with its five scoring areas from the centre outwards as Gold, Red, Blue Black and white. The diameter of the region representing Gold score is 21 cm and each of the other bands is 10.5 cm wide. Find the area of each of the five scoring regions.
View SolutionFind the circumference and area of a circle of radius 4.2 cm.
View SolutionFind the circumference of a circle whose area is `301. 84\ c m^2` .
View SolutionFind the area of a circle whose circumference is 44 cm.
View SolutionThe circumference of a circle exceeds the diameter by 16.8 cm. Find the circumference of the circle.
View SolutionA steel wire when bent in the form of a square encloses an area of `121\ c m^2` . If the same wire is bent in the form of a circle, find the area of the circle.
View SolutionA horse is placed for grazing inside a rectangular field 40 m by 36 m and is tethered to one corner by a rope 14 m long. Over how much area can it graze? `(T a k e\ \ pi=22//7)` .
View SolutionA sheet of paper is in the form of a rectangle `A B C D` in which `A B=40 c m` and `A D=28 c m` . A semi-circular portion with `B C` as diameter is cut off. Find the area of the remaining paper.
View SolutionThe circumference of two circles are in the ratio `2\ :3` . Find the ratio of their areas.
View SolutionThe side of a square is 10 cm. Find the area of circumscribed and inscribed circles.
View SolutionThe sum of the radii of two circles is 140 cm and the difference of their circumferences is 88 cm. Find the diameters of the circles.
View SolutionThe area of a circle inscribed in an equilateral triangle is `154\ c m^2` . Find the perimeter of the triangle. `[U s e\ \ pi=22//7a n d\ sqrt(3)=1. 73]`
View SolutionA field is in the form of a circle. A fence is to be erected around the field. The cost of fencing would be Rs. 2640 at the rate of Rs. 12 per metre. Then, the field is to be thoroughly ploughed at the cost of Re. `0. 50\ p e r\ m^2` . What is the amount required to plough the field? `[T a k e\ \ pi=22//7]` .
View SolutionThe radii of two circles are 8 cm and 6 cm respectively. Find the radius of the circle having its area equal to the sum of the areas of the two circles.
View SolutionThe radii of two circles are 19 cm and 9 cm respectively. Find the radius and area of the circle which has its circumference equal to the sum of the circumferences of the two circles.
View SolutionThe area enclosed between the concentric circles is `770\ c m^2` . If the radius of the outer circle is 21 cm, find the radius of the inner circle.
View SolutionA sector is cut from a circle of radius 21 cm. The angle of the sector is 150 degree . Find the length of its arc and area.
View SolutionFind the area of the sector of a circle whose radius is 14 cm and angle of sector is `45o` .
View SolutionThe minute hand of a clock is 10 cm long. Find the area of the face of the clock described by the minute hand between 9 A.M. and 9.35 A.M.
View SolutionFind the area of the sector of a circle with radius 4 cm and of angle `30o` . Also, find the area of the corresponding major sector. `(U s e\ \ pi=3. 14)`
View SolutionA car has two wipers which do not overlap. Each wiper has a blade of length 25 cm sweeping through an angle of `115o` . Find the total area cleaned at each sweep of the blades.
View SolutionTo warm ships for underwater rocks, a light house throws a red coloured light over a sector of `80o` angle to a distance of 16.5 km. Find the area of the sea over which the ships are warmed.
View SolutionAn umbrella has 8 ribs which are equally spaced. Assuming umbrella to be a flat circle of radius 45 cm. Find the area between the two consecutive ribs of the umbrella.
View SolutionA brooch is made with silver wire in the form of a circle with diameter 35 mm. The wire also used in making 5 diameters which divide the circle into 10 equal sectors as shown in Fig. 15.16. Find: (i) the total length of the silver wire required (ii) the area of each sector of the brooch.
View SolutionFind, in terms of `pi` , the length of the arc that subtends an angle of `30o` at the centre of a circle of radius 4 cm.
View SolutionFind the angle subtended at the centre of a circle of radius 5 cm by an arc of length `(5pi//3)c m` .
View SolutionAn arc of length `20pi` cm subtends an angle of `144o` at the centre of a circle. Find the radius of the circle.
View Solution
All Questions questions and complete solutions for chapter AREAS RELATED TO CIRCLES of RD SHARMA MATHS (HINGLISH) of Class 10