Complete Solutions to SHORT ANSWER QUESTIONS-II of chapter TRIANGLES of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | TRIANGLES |
| VK GLOBAL PUBLICATION | SHORT ANSWER QUESTIONS-II |
| Number Of Questions | 24 |
| Academic Year | 2023-24 |
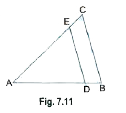
In Fig. 7.11, `Deabs()BC`. If AD= x, DB= x- 2, AE = x + 2 and EC =x -1, find the value of x.
View SolutionE and F are points on the sides PQ and PR respectively of a `DeltaPQR`. Show that `Efabs()QR`. If PQ = 1.28 cm,PR= 2.56 cm,PE = 0.18 cm and PF= 0.36 cm.
View SolutionA vertical pole of length 6 m casts a shadow 4 m long on the ground and at the same time a tower casts a shadow 28 m long. Find the height of the tower.``
View SolutionIn figure, If LM || CB and LN || CD, prove that `(A M)/(A B)=(A N)/(A D)`.
View SolutionIn Fig. 7.15,`DE abs() OQ` and `Dfabs()OR`,Show that `Efabs()QR.`
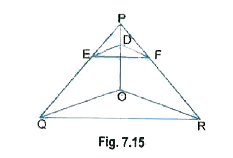
View SolutionThe line joining the mid-points of two sides of a triangle is parallel to the third side.
View SolutionState which pairs of triangles in Figure are similar. Write the similarity criterion used by you for answering the question and also write the pairs of similar triangles in the symbolic form:
View SolutionIn Fig. 4.96, `(A O)/(O C)=(B O)/(O D)=1/2` and `A B=5c m` . Find the value of `D C` . (FIGURE)
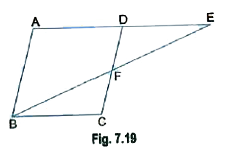
View SolutionE is a point on the side AD produced of a parallelogram ABCD and BE intersects CD at F.Show that `DeltaABE~DeltaCFB`.
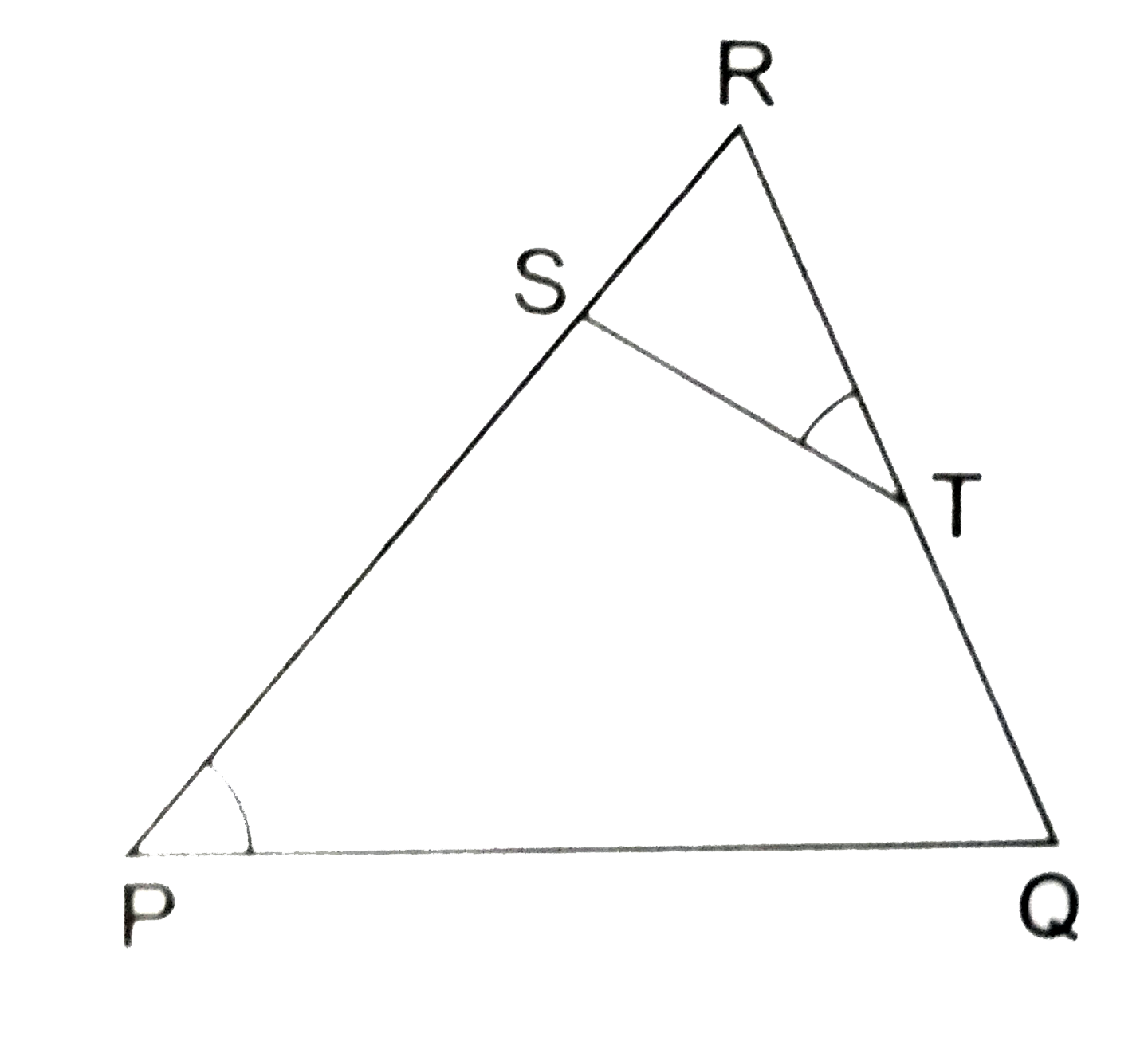
View SolutionIn the given figure, S and T are points on sides PR and QR of `Delta PQR` such that `angle P= angle RTS`. Show that `Delta RPQ~ Delta RTS`.
View SolutionIn figure ABC and AMP are two right triangles, right angles at B and M respectively. Prove that (i) `DeltaA B C DeltaA M P` (ii) `(C A)/(P A)=(B C)/(M P)`
View SolutionD is a point on the side BC of a triangle ABC such that `/_A D C=/_B A C`. Show that `C A^2=C BdotC D`.
View SolutionABC is an equilateral triangle of side 2a. Find each of its altitudes.
View SolutionAn aeroplane leaves an airport and flies due north at a speed of 1000 km per hour. At the same tune, another aeroplane leaves the same airport and flies due west at a speed of 1200 km per hour. |How far apart will be the two planes after 1`1/2
View SolutionIn the given figure, `DeltaABC and DeltaDBC` are on the same base BC. If AD intersects BC at O, prove that `(ar(DeltaABC))/(ar(DeltaDBC))=(AO)/(DO)`
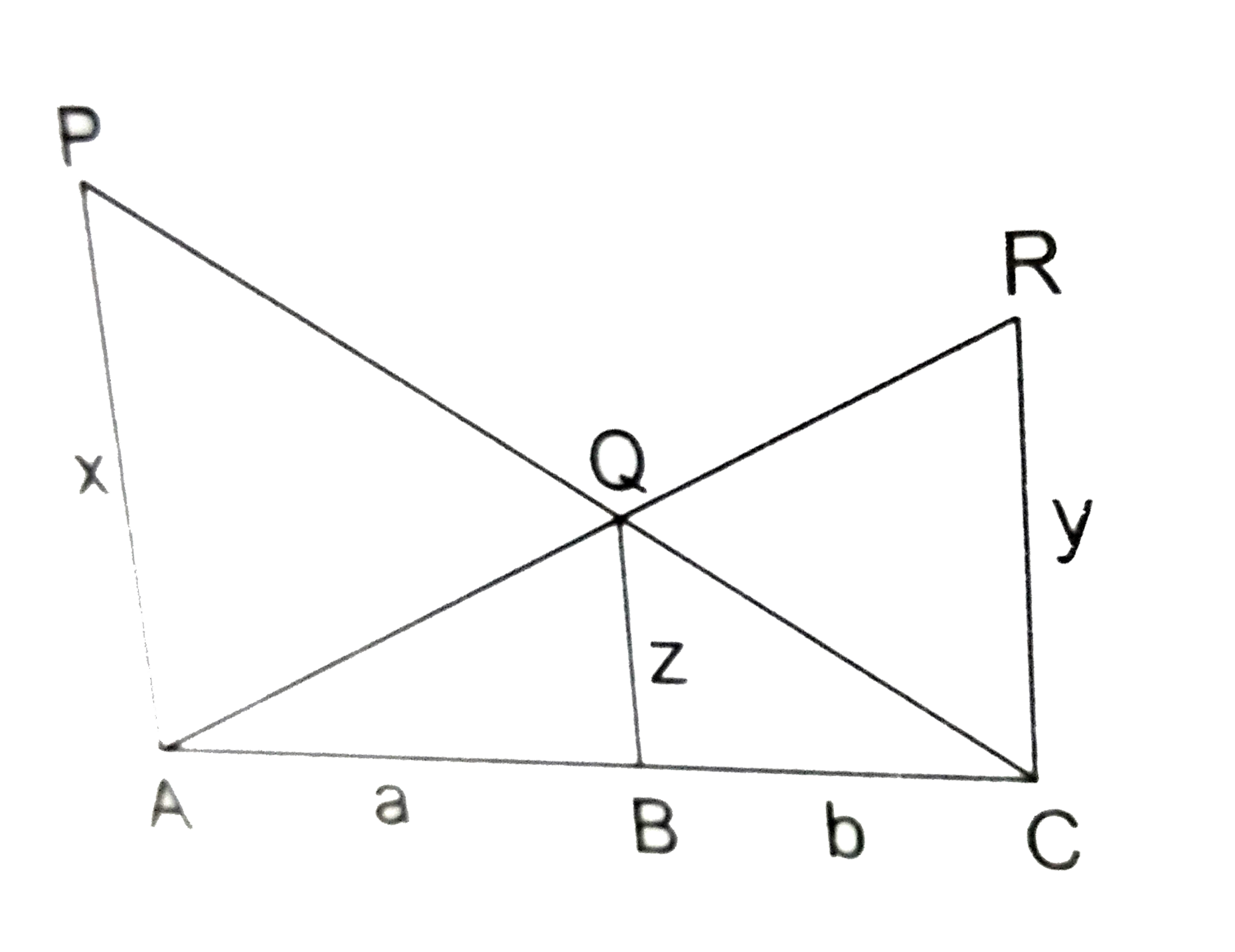
View SolutionIn the given figure, PA, QB and RC each is perpendicular to AC such that `PA=x, RC=y, QB=z, AB=a, and BC=b`
Prove that `(1)/(x)+(1)/(y)=(1)/(z)`
View SolutionIn Fig. 7 .27, if `DeltaABC~ DeltaDEF` and their sides are oflengths (in cm) as marked along them, then find the lengths of the sides of each triangle.
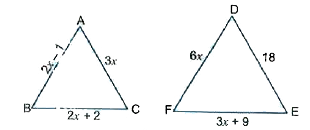
View SolutionIn `Delta ABC` it is given that `(AB)/(AC)=(BD)/(DC)` . If `angle B=70^(@) and angle C=50^(@) "then" angle BAD=?`
View SolutionIf the diagonals of a quadrilateral divide each other proportionally, prove that it is a trapezium
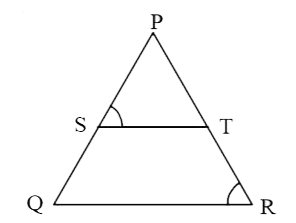
View SolutionIn the given figure `(PS)/(SQ)=(PT)/(TR) and anglePST = anglePRQ.` Prove that PQR is an isoscles triangle.
View SolutionDiagonals of a trapezium ABCD with AB || DC intersect each other at the point O. If AB = 2 CD, find the ratio of the areas of triangles AOB and COD.
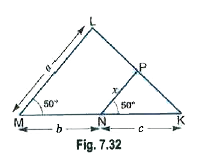
View SolutionIn the given Fig. 7.32, find the value of x in terms of a, b and c.
View SolutionIn the given Fig. 7.33, `Cdabs()LA` and `DEabs()AC`. Find the length of CL if BE= 4 cm and EC= 2 cm.

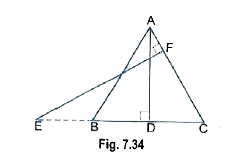
View SolutionIn the given Fig. 7 .34, AB = AC. E is a point on CB produced. If AD is perpendicular to BC and EF perpendicular to AC, prove that `DeltaABD` is similar to `DeltaCEF`.
View Solution
SHORT ANSWER QUESTIONS-II questions and complete solutions for chapter TRIANGLES of VK GLOBAL PUBLICATION MATHS (HINGLISH) of Class 10