Complete Solutions to Level 2 of chapter FIRST LAW OF THERMODYNAMICS of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | FIRST LAW OF THERMODYNAMICS |
| ARIHANT MASTER PROBLEM | Level 2 |
| Number Of Questions | 34 |
| Academic Year | 2023-24 |
A container has a tight fitting movable piston which can slide without friction. The compartment containing spring has vacuum and to the left of the piston there is diatomic gas. If vacuum is created in the right compartment also the piston touches the right wall and the spring is relaxed. Find the heat capacity of the system neglecting the heat capacities of the material of spring, container and the piston. Express your answer in terms of `P_(0), V_(0)` and `T_(0)`.
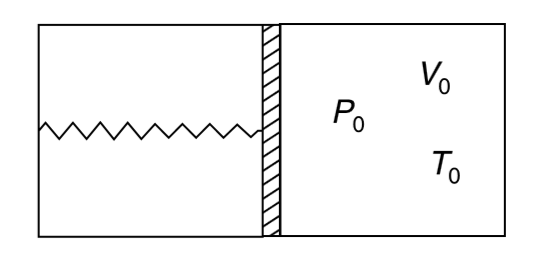 09:20View Solution
09:20View SolutionOne mole of a gas is in state `A[P, V, T_(A)]`. A small adiabatic process causes the state of the gas to change to `B [P + dP, V + dV, T_(B)]`. The changes dV & dP are infinitesimally small and dV is negative. An alternative process takes the gas from state A to B via `A rarr C rarr B`. `A rarr C` is isochoric and `C rarr B` is isobaric path. State at C is `[P + dP, V, T_(C)]`.
(a) Rank the temperatures `T_(A), T_(B)` and `T_(C)` from highest to lowest.
(b) Find g of the gas in terms of `T_(A)`, `T_(B)` and `T_(C)`.
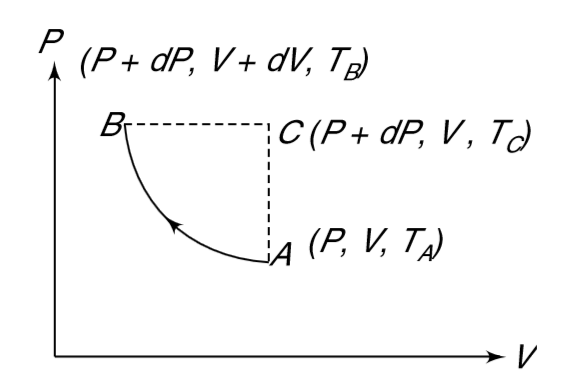 06:39View Solution
06:39View SolutionA metal cylinder of density d, cross sectional area A and height h is standing on a horizontal surface. Coefficient of linear expansion of the metal is `apha .^(@)C^(-1)` and its specific heat capacity is S. Calculate the rise in temperature of the cylinder if a heat `Delta Q` is supplied to it. Assume no atmosphere.
03:08View Solutionn moles of an ideal mono atomic gas is initially at pressure `32 P_(0)` and volume `V_(0)`. Its volume is doubled by an isobaric process. After this the gas is adiabatically expanded so as to make its volume `16V_(0)`. Now the gas is isobarically expanded. Finally, the gas is made to return to its initial state by an isothermal process.
(a) Represent the process on a P–V diagram.
(b) Calculate work done by the gas in one cycle.08:08View SolutionOne end of an insulating U tube is sealed using insulating material. A mono atomic gas at temperature 300 K occupies 20 cm length of the tube as shown. The level of mercury on two sides of the tube differ by 5 cm. The other end of the tube is open to atmosphere. Area of cross section of the tube is uniform and is equal to `0.01 m^(2)`. The gas in the tube is heated by an electric heater so as to raise its temperature to 562.5 K. Assume that no heat is conducted to mercury by the gas.
(a) Find the final length of the gas column.
(b) Find the amount of heat supplied by the heater to the gas.
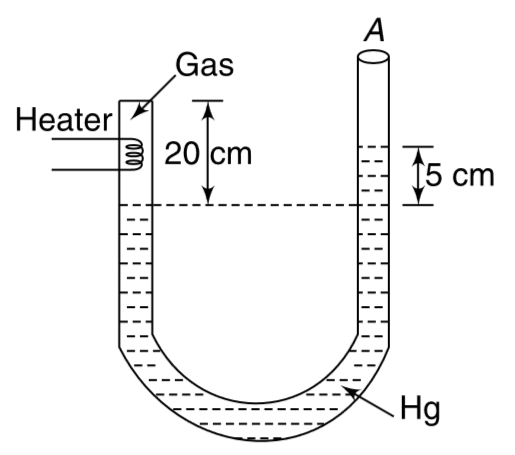 07:22View Solution
07:22View SolutionAir is filled inside a jar which has a pressure gauge connected to it. The temperature of the air inside the jar is same as outside temperature `(= T_(0))` but pressure `(P_(1))` is slightly larger than the atmospheric pressure `(P_(0))`. The stopcock is quickly opened and quickly closed, so that the pressure inside the jar becomes equal to the atmospheric pressure `P_(0)`. The jar is now allowed to slowly warm up to its original temperature `T_(0)`. At this time the pressure of the air inside is `P_(2) (P_(0) lt P_(2) lt P_(1))`. Assume air to be an ideal gas. Calculate the ratio of specific heats `(= gamma)` for the air, in terms of `P_(0), P_(1)` and `P_(2)`.
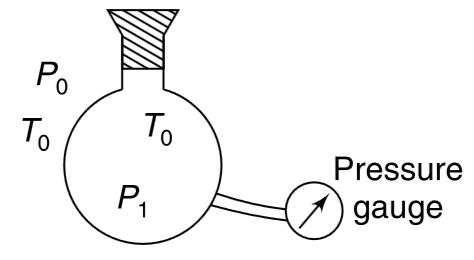 05:18View Solution
05:18View SolutionThe molar specific heat capacity at constant volume `(C_(V))` for an ideal gas changes with temperature as shown in the graph. Find the amount of heat supplied at constant pressure in raising the temperature of one mole of the gas from 200 K to 400 K.
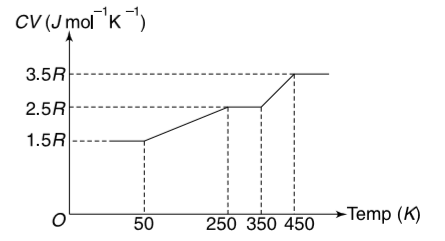 09:14View Solution
09:14View SolutionA sample of oxygen is heated in a process for which the molar specific heat capacity is 2R. During the process the temperature becomes `(32)^(1//3)` times of the original temperature. How does the volume of the gas change?
05:54View SolutionA gas-gun has a cylindrical bore made in an insulating material. Length of the bore is L. A small bullet having mass m just fits inside the bore and can move frictionlessly inside it. Initially n moles of helium gas is filled in the bore to a length `L_(0)`. The bullet does not allow the gas to leak and the bullet itself is kept at rest by a stopper S. The gas is at temperature `T_(0)`. The gun fires if the stopper S is removed suddenly. Neglect atmospheric pressure in your calculations [Think that the gun is in space].
(a) Calculate the speed with which the bullet is ejected from the gun.
(b) Find the maximum possible speed that can be imparted to the bullet by using n moles of helium at temperature `T_(0)`.
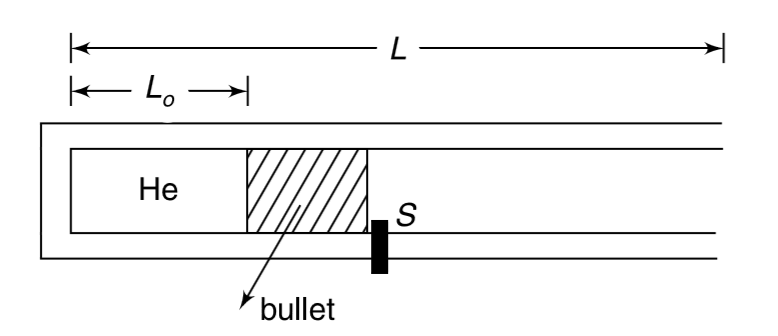 05:22View Solution
05:22View SolutionDuring a process carried on an ideal gas it was found that `eta (lt 1)` times the heat supplied to the gas is equal to increase in internal energy of the gas. Write the process equation in terms of pressure (P) and volume (V) of the gas. It is given that ratio of specific heats for the gas is `(C_(P))/(C_(V)) = gamma`.
09:14View SolutionAn ideal gas is taken through a thermodynamic cycle ABCDA. In state A pressure and volume are `P_(0)` and `V_(0)` respectively. During the process `A rarr B`, work done by the gas is zero and its temperature increases two fold. During the process `B rarr C`, internal energy of the gas remains constant but work done by it is `W_(BC) = – P_(0)V_(0) ln2`. In the process `C rarr D`, the temperature decreases by 50% while the volume does not change. In the process `D rarr A` the temperature of the gas does not change.
(a) Draw pressure – volume (P–V) and pressure – density `(P – rho)` graph for the cyclic process.
(b) Calculate work done on the gas during the cycle.10:15View Solution(i) A conducting piston divides a closed thermally insulated cylinder into two equal parts. The piston can slide inside the cylinder without friction. The two parts of the cylinder contain equal number of moles of an ideal gas at temperature `T_(0)`. An external agent slowly moves the piston so as to increase the volume of one part and decrease that of the other. Write the gas temperature as a function of ratio `(beta)` of the volumes of the larger and the smaller parts. The adiabatic exponent of the gas is `gamma`.
(ii) In a closed container of volume V there is a mixture of oxygen and helium gases. The total mass of gas in the container is m gram. When Q amount of heat is added to the gas mixture its temperature rises by `Delta T`. Calculate change in pressure of the gas.16:32View SolutionA spherical container made of non conducting wall has a small orifice in it. Initially air is filled in it at atmospheric pressure `(P_(0))` and atmospheric temperature `(T_(0))`. Using a small heater, heat is slowly supplied to the air inside the container at a constant rate of H J/s. Assuming air to be an ideal diatomic gas find its temperature as a function of time inside the container.
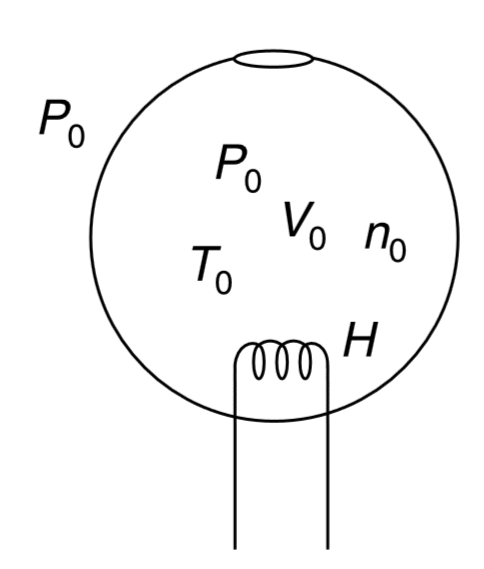 03:46View Solution
03:46View SolutionA glass tube is inverted and dipped in mercury as shown. One mole of an ideal monoatomic gas is trapped in the tube and the tube is held so that length of the tube above the mercury level is always `h_(0)` meter. The atmospheric pressure is equal to `h_(0)` meter of mercury. The mercury vapour pressure, heat capacity of mercury, tube and the container are negligible. How much heat must be supplied to the gas inside the tube so as to increase its temperature by `Delta T`?
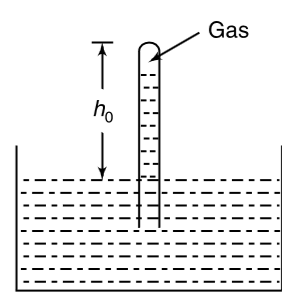 06:05View Solution
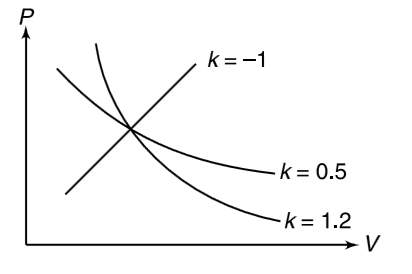
06:05View SolutionFigure shows P versus V graph for various processes performed by an ideal gas. All the processes are polytropic following the process equation `PV^(k) =` constant.
(i) Find the value of k for which the molar specific heat of the gas for the process is `(C_(P)+C_(V))/(2)`. Does any of the graph given in figure represent this process?
(ii) Find the value of k for which the molar specific heat of the gas is `C_(V) + C_(P)`. Assume that gas is mono atomic. Draw approximately the P versus V graph for this process in the graph given above.
 03:37View Solution
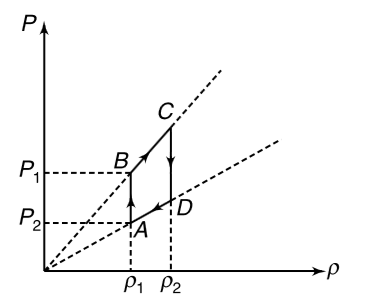
03:37View Solutionn' moles of an ideal gas having molar mass M is made to undergo a cyclic process ABCDA. The cycle has been represented on a pressure (P) density `(rho)`) diagram.
(a) Draw the corresponding P – V diagram
(b) Calculate the work done by the gas in the cycle
 10:30View Solution
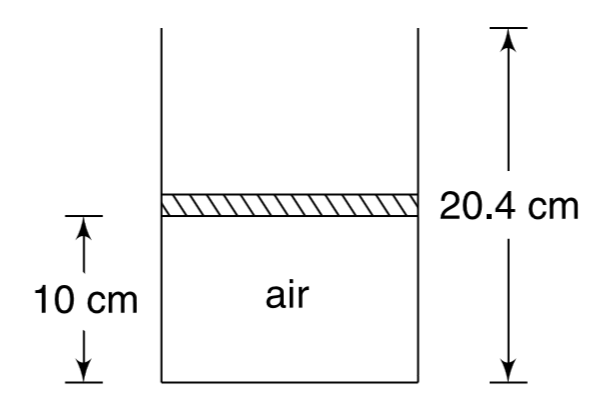
10:30View SolutionIn the arrangement shown in figure, the piston can smoothly move inside the cylinder. The mass of the piston is m = 100 g and its cross sectional area is `A = 10 cm^(2)`. The length of air column at temperature of `T = 27^(@)C` is 10 cm. Overall length of the cylinder is 20.4 cm. The container is turned upside down and the length of the air column in equilibrium was found to be l at `27^(@)C`. Take `R = (25)/(3)J mol^(-1) K^(-1)` and assume air to be diatomic gas. `g = 10 m//s^(2)`, atmospheric pressure is `1.01 xx 10^(5) Nm^(2)`
(a) Find l
(b) If the air in the container is supplied heat in upside down position, the piston slowly begins to move down, and ultimately it gets ejected out of the cylinder. Calculate the amount of heat that the air must absorb for the piston to come out.
 07:23View Solution
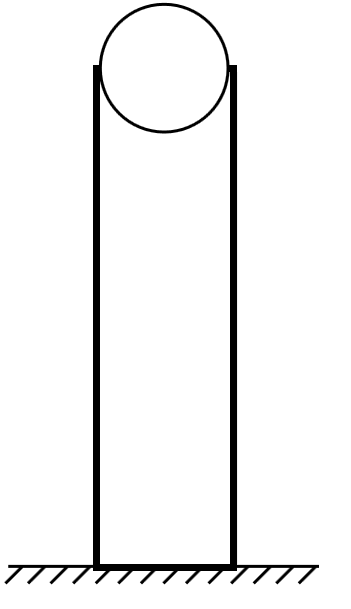
07:23View SolutionThere is a long vertical tube of radius r containing air at atmospheric pressure. A steel ball is held at the mouth of the tube and dropped. The ball has radius r and it just fits inside the tube. The tube wall is perfectly smooth and no air can leak from the tube as the ball falls inside it. The ball falls through half the length of the tube before coming to rest. Assume that wall of the tube is perfectly conducting and temperature of the air inside the tube remains constant. Density of steel is d and atmospheric pressure is `P_(0)` and take `L gt gt r`. Take air to be an ideal gas.
(a) Find the radius (r) of the tube.
(b) At what depth from the top of the tube the ball will be in equilibrium?
 06:36View Solution
06:36View SolutionA ball of radius r fits tightly inside a tube attached to a container. There is no friction between the tube wall and the ball. Volume of air inside the container is `V_(0)` when the ball is in equilibrium. Density of the material of the ball is d and atmospheric pressure is `P_(0)`. If the ball is displaced a little from its equilibrium position and released, find time period of its oscillation. Assume that temperature in the container remains constant and that air is an ideal gas.
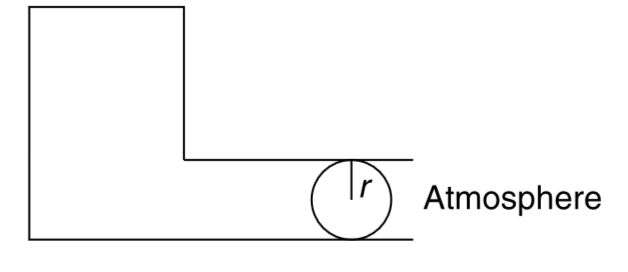 View Solution
View SolutionAn ideal mono atomic gas is at temperature `T_(0)`. The pressure and volume are quasi-statically doubled such that the process traces a straight line on the PV diagram.
(a) Calculate the heat absorbed by the gas in the process if number of moles of the gas in the sample is n.
(b) Calculate the average molar specific heat capacity of the gas for the process.08:57View SolutionA cylinder contains equal volumes of Ar and `H_(2)`, separated by a freely movable piston B. Piston A can also move without friction. Volume of each gas in equilibrium is`V_(0)`. All walls of the container including piston A are non conducting.
(i) Piston A is pushed down slowly till the volume occupied by argon becomes `(V_(0))/(4)` . Find the volume of `H_(2)`. Assume that piston B is also non conducting.
(ii) Now assume that piston B is conducting and assume that each gas has n moles. The external agent performs work `W_(0)` in slowly pushing down the piston A. Find rise in temperature of each gas.
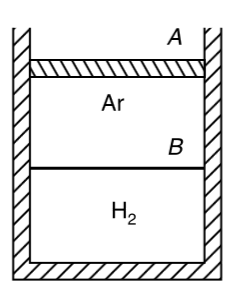 05:10View Solution
05:10View SolutionAn ideal gas is taken through cycle 1231 (see figure) and the efficiency of the cycle was found to be 25%. When the same gas goes through the cycle 1341 the efficiency is 10%. Find the efficiency of the cycle 12341.
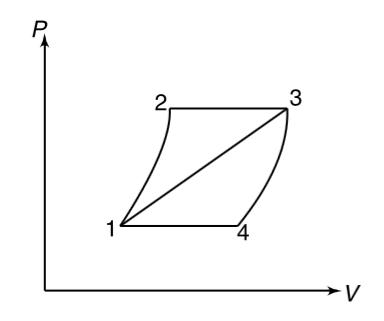 View Solution
View SolutionA heat engine is based on a gaseous cycle comprising of four processes viz. isothermal expansion at temperature `(T_(1))`, isochoric cooling to temperature `T_(2)`, isothermal compression (at `T_(2)`) and isochoric heating back to temperature `T_(1)`. The engine has been designed so as to completely use the heat rejected during isochoric cooling, in the isochoric heating process. Calculate the efficiency of this reversible cycle. Show the process on a PV graph.
07:48View SolutionAn ideal gas with a known `gamma`, completes a cycle consisting of two isotherms and two isobars. The isothermal processes are executed at temperatures T and `T' (lt T)` and isobaric processes are completed at pressures `P_(0)` and `eP_(0)` [e = base of natural logarithm]. Find the efficiency of the cycle.
14:32View SolutionOne mole of a mono atomic gas of molar mass M undergoes a cyclic process as shown in the figure. Here `rho` is density and P is pressure of the gas.
(a) Calculate the heat rejected by the gas in one complete cycle.
(b) Find the efficiency of the cycle.
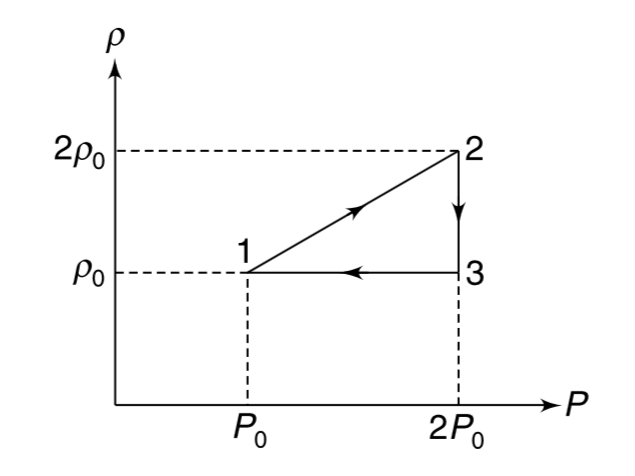 16:10View Solution
16:10View SolutionHelum gas is used as working substance in an engine working on a thermodynamic cycle A – B – C – D – A. Process AB is isobaric, BC is adiabatic compression. During process CD, pressure is increased keeping the volume constant and DA is an isothermal process. The gas has maximum volume at A and the ratio of maximum to minimum volume during the entire cycle is `8sqrt(2)` . Also, the ratio of maximum to minimum absolute temperature is 4.
(a) Represent the cycle on a P – V diagram.
(b) Calculate efficiency of the cycle in percentage if it is used as an engine. [Take ln 2 = 0.693]12:17View SolutionA ring shaped tube has uniform cross sectional area and its entire volume is `2V_(0)`. The tube is well insulated from the surrounding. Inside the tube there is an adiabatic fixed wall `S_(1)` and another movable adiabatic partition `S_(2)`. Initially, the movable partition is diametrically opposite to S1 and the two halves of the tube have equal amount of an ideal gas (g = 1.5) at same pressure `P_(0)`. Now, a heater H is switched on which supplies heat slowly to one of the chambers. Heater is kept on till the partition `S_(2)` moves through the quarter of the circle. At this position the heater is switched off and the partition `S_(2)` remains in equilibrium. Neglect any friction as well as heat loss to the surrounding through the walls of the tube. Find the heat supplied by the heater to the gas.
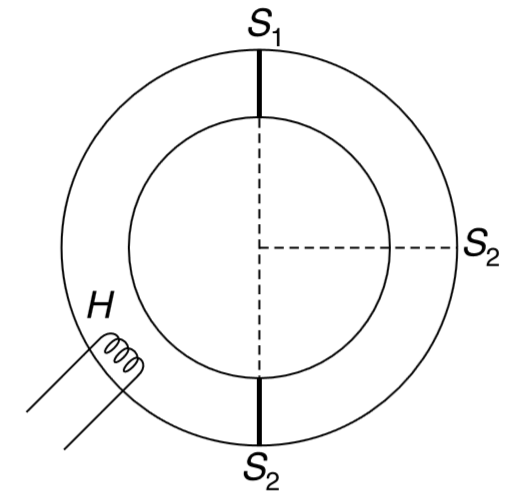 04:51View Solution
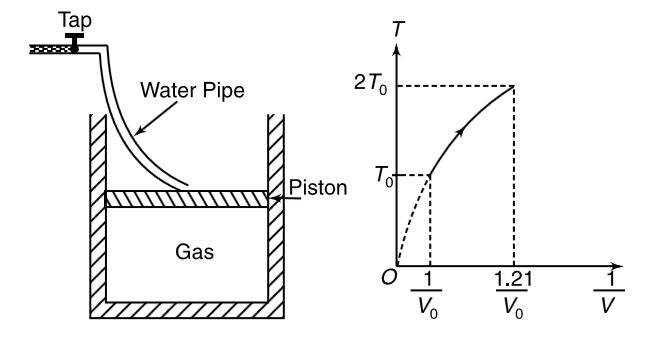
04:51View SolutionA cylindrical container has insulating wall and an insulating piston which can freely move up and down without any friction. It contains a mixture of ideal gases. Originally the gas is at atmospheric pressure `P_(0)` and temperature `(T_(0))`. A tap positioned above the container is opened and it supplies water at a constant rate of `(dm)/(dt)= 0.25 kg//s`. The water collects above the piston in the container and the gas compresses. The tap is kept open till the temperature of the gas is doubled. During the process the T vs `(1)/(V)` graph for the gas was recorded and found to be a parabola with its vertex at origin as shown in the graph. Area of piston `A = 1.515 xx 10^(-3) m^(2)` and atmospheric pressure `= 10^(5) N//m^(2)`
(a) Find the ratio of `V_(rms)` and speed of sound in the gaseous mixture.
(b) For how much time the tap was kept open?
 06:02View Solution
06:02View SolutionOn a hot summer day the temperature inside is house is `T_(0)` and the outside temperature is `T_(0) + Delta T`. How does the energy consumed by the air conditioner depend on `T_(0)` and `Delta T` ? Assume that the air conditioner operates ideally at its maximum coefficient of performance.
06:02View SolutionA room air conditioner is a Carnot cycle based heat engine run is reverse. An amount of heat `Q_(2)` is absorbed from the room at a temperature `T_(2)` into coils having a working gas (these gases are not good for environment!). The gas is compressed adiabatically to the outside temperature `T_(1)`. Then the gas is compressed isothermally in the unit outside the room, giving off an amount of heat `Q_(1)`. The gas expands adiabatically back to the temperature`T_(2)` and the cycle is repeated. The electric motor electric consumes power P.
(i) Find the maximum rate at which heat can be removed from the room.
(i) Heat flows into the room at a constant rate of `k DeltaT` where k is a constant and `Delta T` is temperature difference between the outside and inside of the room. Find the smallest possible room temperature in terms of `T_(1), k` and P.04:56View SolutionA Carnot cycle based ideal heat engine operates between two tanks each having same mass m of water. The source tank has an initial temperature of `T_(1) = 361 K` and the sink tank has an initial temperature of `T_(2) = 289 K`. Assume that the two tanks are isolated from the surrounding and exchange heat with the engine only. Specific heat of water is s.
(a) Find the final common temperature of the two tanks.
(b) Find the total work that the engine will be able to deliver by the time the two tanks reach common temperature.
 10:28View Solution
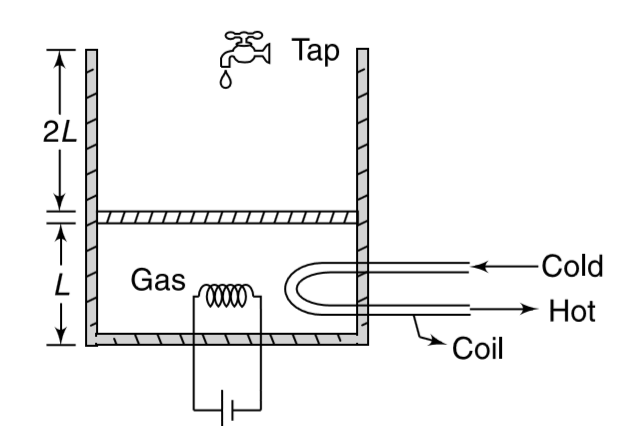
10:28View SolutionA cylindrical container of height 3L and cross sectional area A is fitted with a smooth movable piston of negligible weight. It contains an ideal diatomic gas. Under normal atmospheric pressure `P_(0)` the piston stays in equilibrium at a height L above the base of the container. The gas chamber is provided with a heater and a copper coil through which a cold liquid can be circulated to extract heat from the gas. Volume occupied by the heater and the liquid coil is negligible. Following set of operations are performed to take the gas through a cyclic process.
(1) Heater is switched on. At the same time a tap above the cylinder is opened. Water fills slowly in the container above the piston and it is observed that the piston does not move. Water is allowed to fill the container so that the height of water column becomes L. Now the tap is closed.
(2) The heater is kept on and the piston slowly moves up. Heater is switched off at the time water is at brink of overflowing.
(3) Now the cold liquid is allowed to pass through the coil. The liquid extracts heat from the gas. Water is removed from the container so as to keep the position of piston fixed. Entire water is removed and the gas is brought back to atmospheric pressure.
(4) The circulation of cold liquid is continued and the piston slowly falls down to the original height L above the base of the container. Circulation of liquid is stopped. Assume that the container is made of adiabatic wall and density of water is `rho`. Force on piston due to impact of falling water may be neglected.
(a) Draw the entire cycle on a P–V graph.
(b) Find the amount of heat supplied by the heater and the amount of heat extracted by the cold liquid from the gas during the complete cycle.
 10:26View Solution
10:26View SolutionAn adiabatic cylindrical chamber with a frictionless movable piston has been placed on a smooth horizontal surface as shown. One mole an ideal monotonic gas is enclosed inside the chamber. Mass of the piston is M and mass of the remaining chamber including the gas is 4 M. The gas is at atmospheric pressure and temperature. A particle of mass M moving horizontally with speed v, strikes the piston elastically. Find the change in temperature of the gas when the compression is maximum.
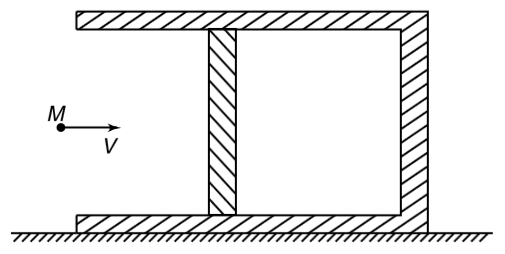 04:23View Solution
04:23View Solution(a) A polytropic process for an ideal gas is represented by `PV^(x) =` constant, where `x != 1`. Show that molar specific heat capacity for such a process is given by `C = C_(v) + (R)/(1-x)`.
(b) An amount Q of heat is added to a mono atomic ideal gas in a process in which the gas performs a work `(Q)/(2)` on its surrounding. Show that the process is polytropic and find the molar heat capacity of the gas in the process.13:54View Solution
Level 2 questions and complete solutions for chapter FIRST LAW OF THERMODYNAMICS of ARIHANT PHYSICS (HINGLISH) of Class 11
