Complete Solutions to All Questions of chapter MOTION IN !,2,2 DIMENSIONS AND POJECTILE MOTION of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | MOTION IN !,2,2 DIMENSIONS AND POJECTILE MOTION |
| BITSAT GUIDE | All Questions |
| Number Of Questions | 86 |
| Academic Year | 2023-24 |
A cat wants to catch a rat. The cat follows the path whose equation is `x+y=0`. But rat follows the path whose equation is `x^(2)+y^(2)=4`. The coordinates of possible points of catching the rat are
View SolutionSpeed is too velocity is
View SolutionA person travelling on a straight line moves with a uniform velocity `v_1` for some time and with uniform velocity `v_2` for the next equal time. The average velocity v is given by
View SolutionA car moves at `80km` in the first half of total time of motion and at `40km` in the later half. Its average speed is
View SolutionA particle moves with constant speed `v` along a regular hexagon `ABCDEF` in the same order. Then the magnitude of the avergae velocity for its motion form `A` to
View SolutionDuring the shooting of a superhit film 'MARD' Amitabh Bachahan was waiting for his beloved Amrita Singh with his dog. When he saw her approaching, thedog was excited and dashed to her than back to master and so on, never stopping. How far would you estimate the dog ran if his speed is `30km//h` and each of them walked at `4km//h`, starting `400m` apart?
View SolutionTwo particles start from the same point with different speeds but one moves along `y=a sin omega x` and other moves along curve `y=cos omegax`
View SolutionA sheet of wood moves over a smooth surface (shown in the figure). The magnitude of velocity of `C` is
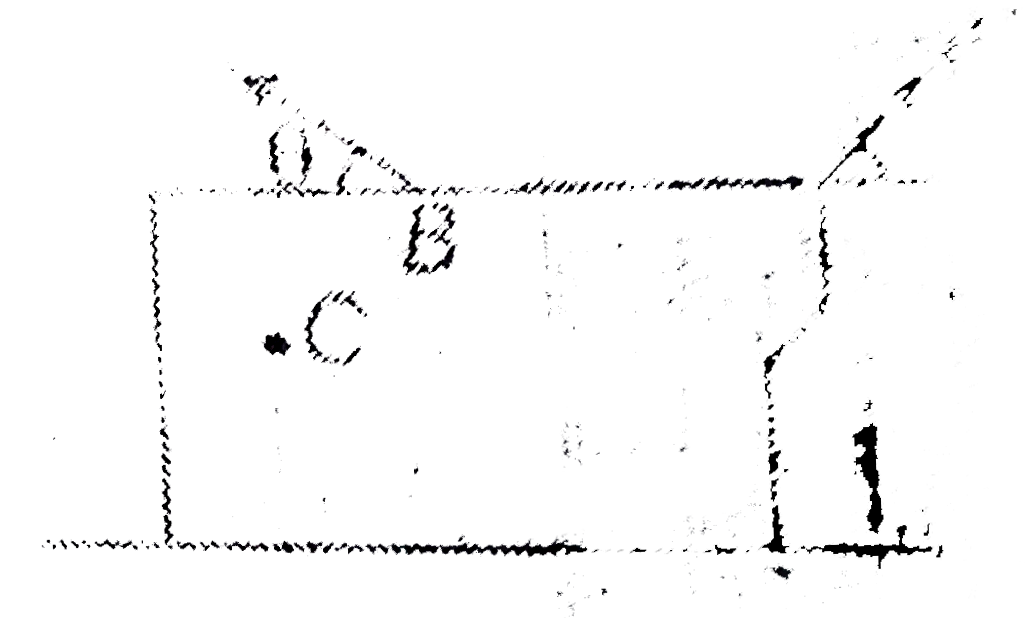
View SolutionIn the arrangement shown in figure, the ends `P` and `Q` of an inextensible string move downwards with uniform speed `u`. Pulleys `A` and `B` are fixed. The mass `m` moves upwards with a speed
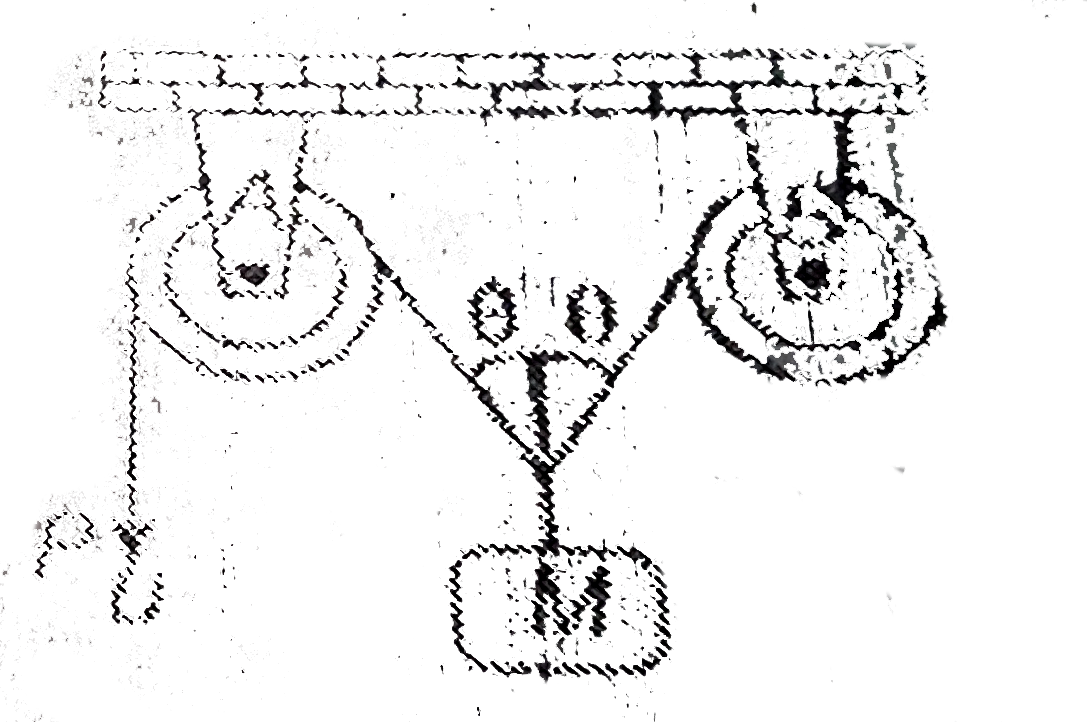
View SolutionIn the figure find the speed of pulley `P`

View SolutionA link `AB` is moving in a vertical plane. At a certain instant when the link is inclined `60^(@)` to the horizontal the point `A` is moving horizontaly at `3m//s`, while `B` is moving in the vertical direction. What is the velocity of `B`?
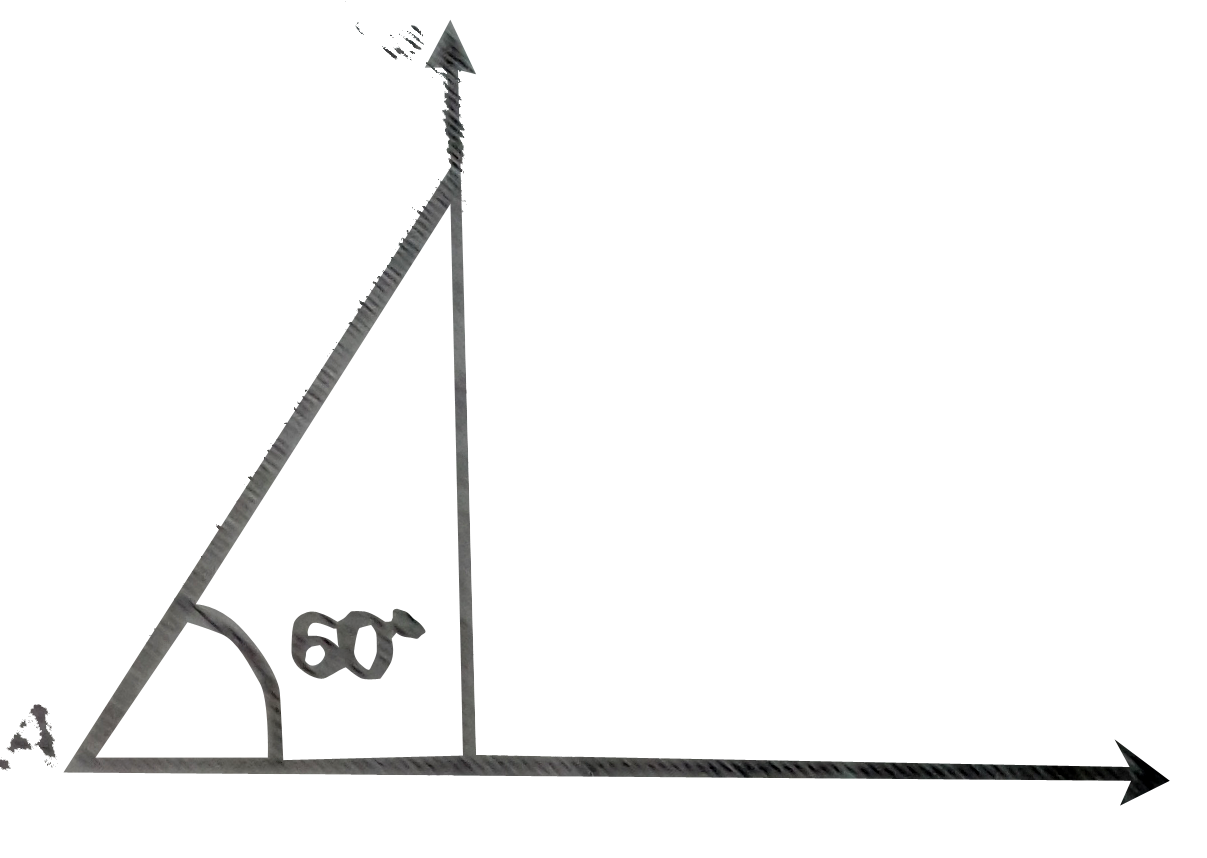
View SolutionTwo intersecting straight lines move parallel to themselves with speeds `3m//s` and `4m//s`, respectively. The speed of the point of intersection of the lines, if the angle between them is `90^(@)` will be
View SolutionThe displacement time graph is shown in figure. The instantaneous velociyt is negative at the point.
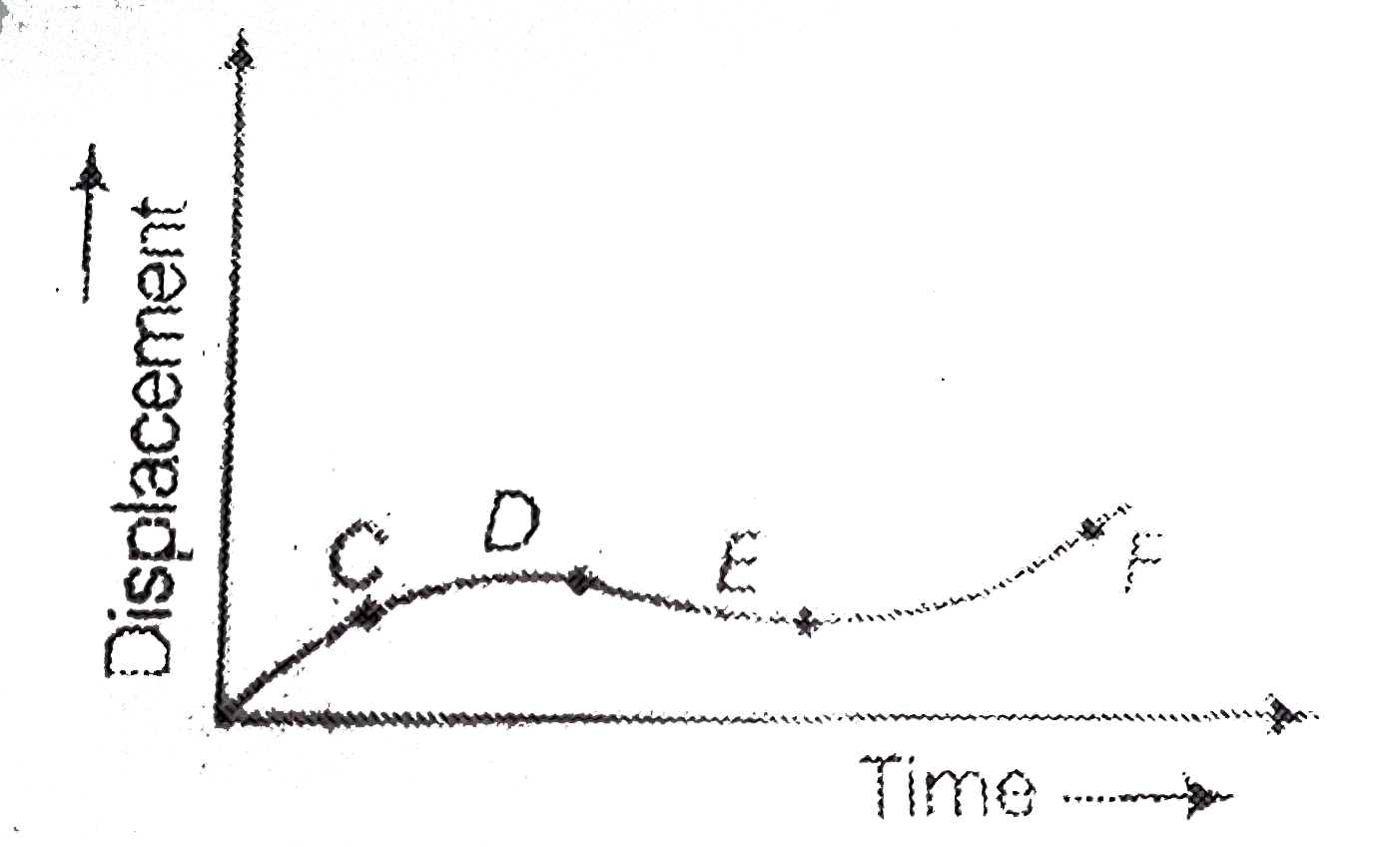
View SolutionIn the given `x-t` curve,

View SolutionWhich of the following speed time graph exists in the nature?
View SolutionTwo particles describes the same circle of radius `a` in the same sense with the same speed `v`. What is their relative angular velocity?
View SolutionA particle starts with a velocity of `2m//s` and moves in a straight line with a retardation of `0.1m//s^(2)`. The time that it takes to describe `15m` is
View SolutionA particle starts from rest with acceleration `2m//s^(2)`. The acceleratioin of the particle of the particle descreases down to zero uniformly during time -interval of `4s`. The velocity of particle after `2s` is
View SolutionAn aeroplane moves `400m` towards north, `300m` towards west and then `1200m` vertically upward. Then its displacement from the initial position is
View SolutionThe displacement of a particle is moving by `x = (t - 2)^2` where `x` is in metres and `t` in second. The distance covered by the particle in first `4` seconds is.
View SolutionA person walks up a stalled escalator in 90 s. When standingon the same escalator, now moving, he is carried in 60 s.The time it would take him to walk up the moving escalator will be:
View SolutionA body starts from rest and moves with constant acceleration. The ratio of distance covered by the body in `nth` second to that covered in `n` second is.
View SolutionA particle moving with a uniform acceleration along a straight line covers distances `a` and `b` in successive intervals of `p` and `q` second. The acceleration of the particle is
View SolutionA body moves along `x`-axis with velocity `V_(x)` at position `x`. If the plot `v_(x)-x` is an ellipse with major axis `2A` and minor axis `2V_(0)` the maximum acceleration has a modulus
View SolutionThe distance time graph of a particle at time `t` makes angle `45^(@)` with respect to time axis. After `1s`, if makes angle `60^(@)` with respect to time axis. What is the acceleration of the particle?
View SolutionThe velocity-time plot for a particle moving on a straight line is shown in the figure, then
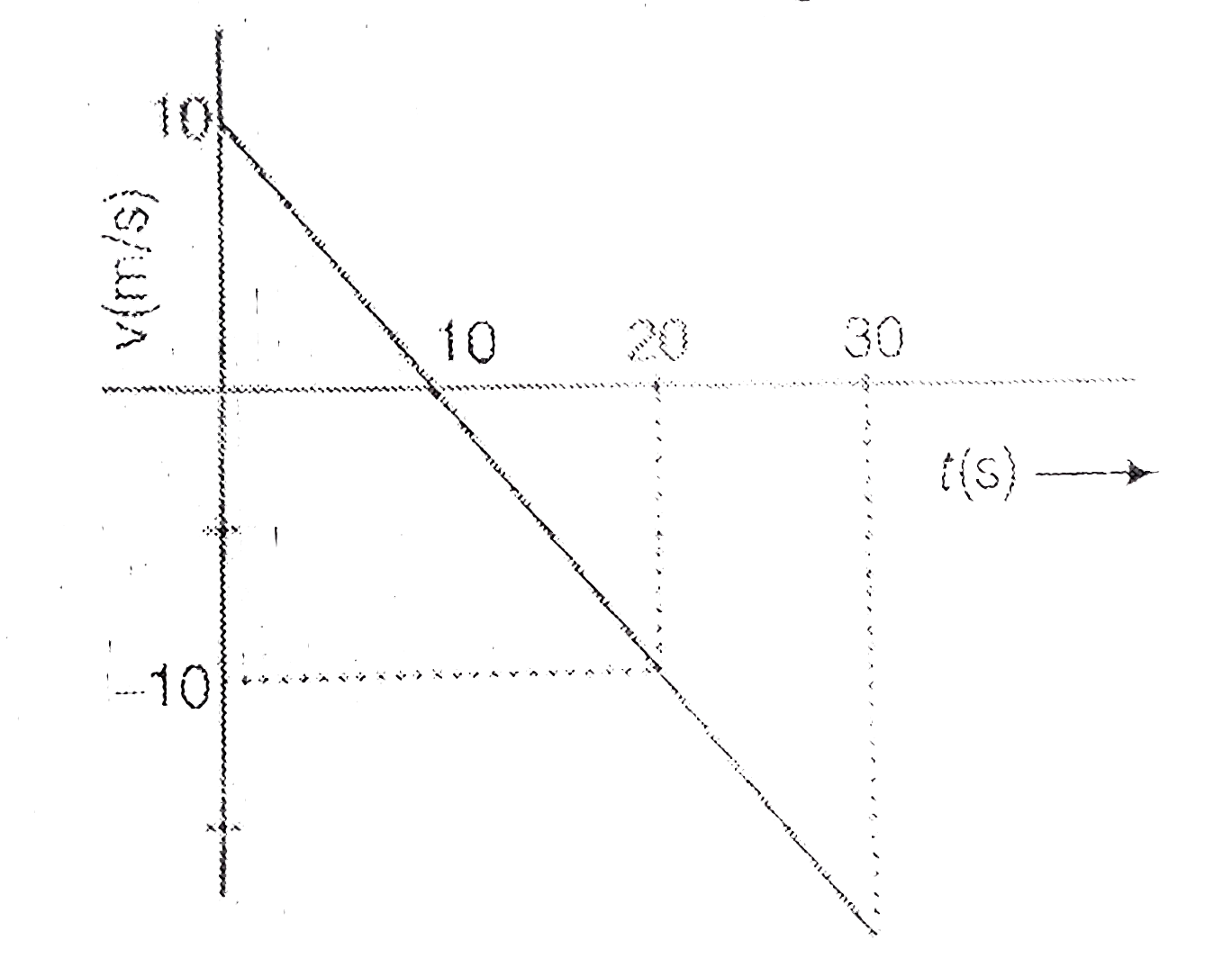
View SolutionThe acceleration of a train between two stations 2 kilometre apart is shown in the figure. If train starts from rest than maximum speed of the train is

View SolutionA body is at rest at ` x =0 ` . At ` t = 0`, it starts moving in the positive `x - direction` with a constant acceleration . At the same instant another body passes through ` x= 0 ` moving in the positive ` x - direction ` with a constant speed . The position of the first body is given by `x_(1)(t)` after time 't', and that of the second body by ` x_(2)(t)` after the same time interval . which of the following graphs correctly describes `(x_(1) - x_(2))` as a function of time 't' ?
View SolutionA particle moves in a straight line, so that after `t` second, the distance `x` from a fixed point `O` on the line is given by `x=(l-2)^(2)(t-5)`. Then
View SolutionA bird flies for 4s with a velocity `V=(t-2) m//s` in a straight line where t= time in second Calculate the displacement and distance covered by the bird
View SolutionThe velocity of particle is `v=v_(0)+"gt"+ft^(2)`. If its position is `x=0` at `t=0` then its displacement after unit time `(t=1)` is
View SolutionA particle located at `x = 0` at time `t = 0`, starts moving along with the positive ` x-direction` with a velocity 'v' that varies as ` v = a sqrt(x)`. The displacement of the particle varies with time as
View SolutionA particle moves as such acceleration is given by a = 3 sin 4t, then :
View SolutionA particle moves along a straight line such that its position` x` at any time `t` is `x=3t^(2)-t^(3)`, where `x` is in metre and `t` in second the
View SolutionThe motion of a body falling from rest in a resting medium is described by the equation `(dv)/(dt)=a-bv`, where `a` and `b` are constant. The velocity at any time `t` is
View SolutionA rectangular box is sliding on a smooth inclined plane of inclination `theta`. At `t=0` the box starts to move on the inclined plane. A bolt starts to fall from point `A`. Find the time after which bolt strikes the bottom surface of the box.
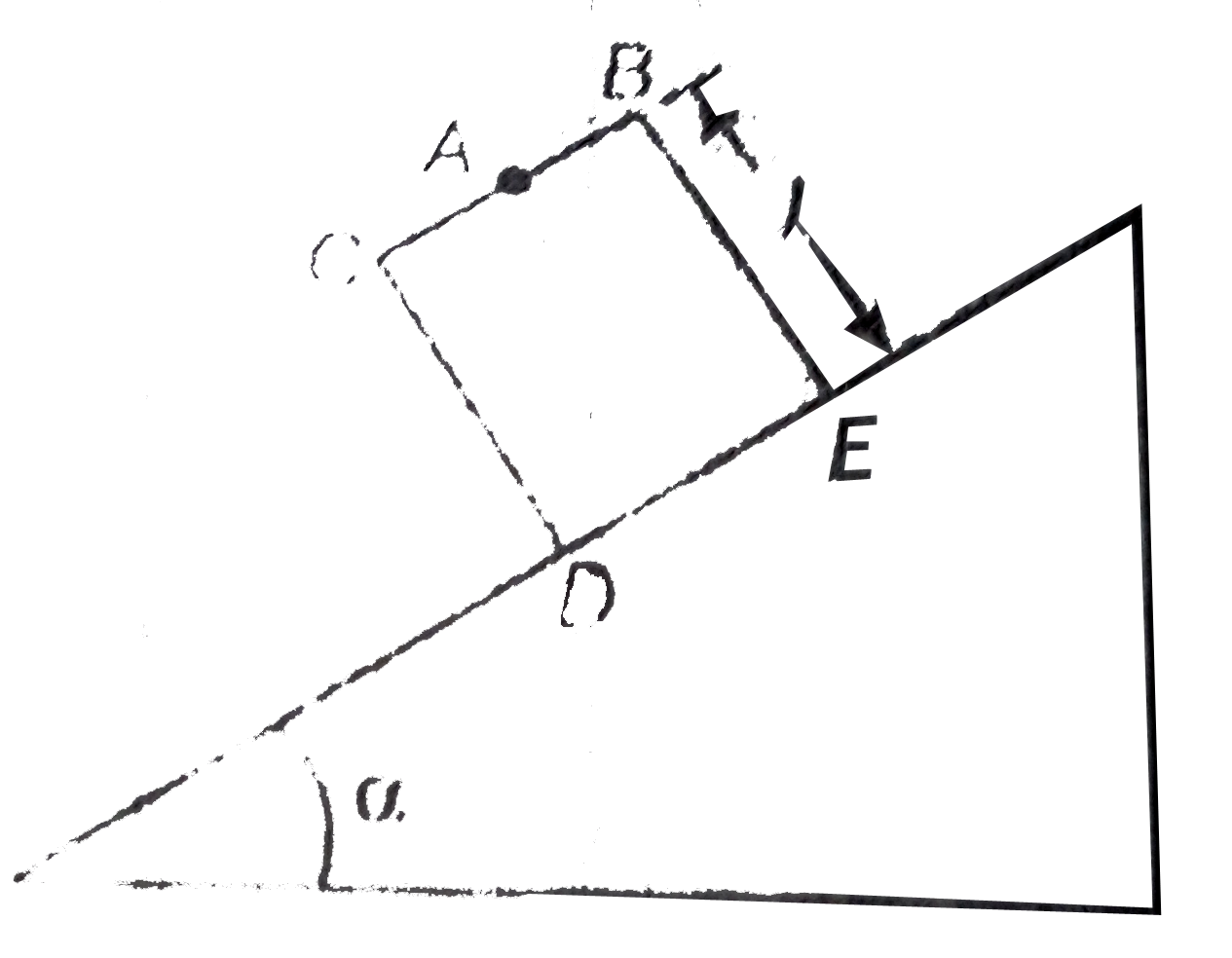
View SolutionA car , starting from rest, accelerates at the rate `f` through a distance `S` then continues at constant speed for time `t` and then decelerates at the rate `(f)/(2)` to come to rest . If the total distance traversed is `15 S` , then
View SolutionAn object moves startig from rest through a resistive medium, such that its acceleration is related to velocity as `a=3-2v`. Then
View SolutionIf the velocity of a moving particle `vpropx^(n)`, where `x` is the displacement then
View SolutionA particle is projected vertically upward in vacum with a speed `40m//s` then velocity of particle when it reaches at maximum height `2s` before, is
(Take `g=10m//s`)
View SolutionA juggler keeps on moving four balls in the air throwing the balls after regular intervals. When one ball leaves his hand `("speed" = 20 ms^-1)` the positions of other balls (height in m) `("Take" g = 10 ms^-2)`.
View SolutionBalls are thrown vertically upwards in such a way that the next ball is thorwn when the previous one is at the maximum height. If the maximum hieght is `5m`, the number of balls thrown per minute will be
View SolutionA ball is dropped vertically from a height `d` above the ground. It hits the ground and bounes up vertically to a height `d//2`. Neglecting subsequent motion and air resistance, its speed `v` varies with the height `h` above the ground as
View SolutionA ball is thrown vertically upwards. Which of the following graph/graphs represent velocity time graph of the ball during its flight ( air resistance is neglected).
View SolutionAn object is thrown upward with a velocity `u`, then displacement -time graph is
View SolutionA balloon going upward with a velocity of `12m//s` is at a height of `65m` from the earthh surface at any instant. Exactly at this instant a packet drops from it. How much time will the packet take in reaching the surface of earth?(Take `g=10m//s^(2)`)
View SolutionIf a stone is relased from a balloon moving upwards with certain velocity `v_(0)` at certain height above earth's surface then velocity time curve of stone's motion can be best represented by : (g = constant)
View SolutionA particle `P` is at the origin starts with velocity `u=(2hati-4hatj)m//s` with constant acceleration `(3hati+5hatj)m//s^(2)`. After travelling for `2s` its distance from the origin is
View SolutionAt an instasnt `t` the coordinates of a particles are `x=at^(2), y=bt^(2)` and `z=-0`. The magnitude of velocity of particle at an instant is
View SolutionIf `x=a(cos theta+theta sin theta)` and `y=a(sin theta-theta cos theta)` and `theta` increases at uniform rate `omega`. The velocity of particle is
View SolutionA particle is moving with velocity ` vecv = k( y hat(i) + x hat(j)) `, where `k` is a constant . The genergal equation for its path is
View SolutionA light rigid rod is placed on a smooth horizontal surface. Initially the end `A` begins to move vertically upward with constant velocity `v_(0)` and centre of the rod upward with a velocity `v_(0)//2` having downward acceleration `a_(0)//2`, the other end moves downward with
View SolutionAt the top of the trajectory of a projectile, the directions of its velocity and acceleration are
View SolutionA projectile is thrown at an angle of `theta=45^(@)` to the horizontal, reaches maximum reaches a maxium height of `16m`, then
View SolutionA heavy stone is thrown from a cliff of height h in a given direction. The speed with which it hits the ground
View SolutionA particle of mass m is projected with a velocity v making an angle of `45^@` with the horizontal. The magnitude of the angular momentum of the projectile abut the point of projection when the particle is at its maximum height h is.
View SolutionTwo particles are projected vertically upward with the same velocity on two diferent planes with accelerations due to gravities `g_(1)` and `g_(2)` respectively. If they fall back to their initial points of projection after lapse of time `t_(1)` and `t_(2)` respectively. Then
View SolutionA point `p` moves in counter - clockwise direction on a circular path as shown in the figure . The movement of 'p' is such that it sweeps out in the figure . The movement of 'p' is such that it sweeps out a length `s = t^(3) + 5 ` , where `s` is in metres and ` t` is in seconds . The radius of the path is `20 m` . The acceleration of 'P' when ` t = 2 s` is nearly .
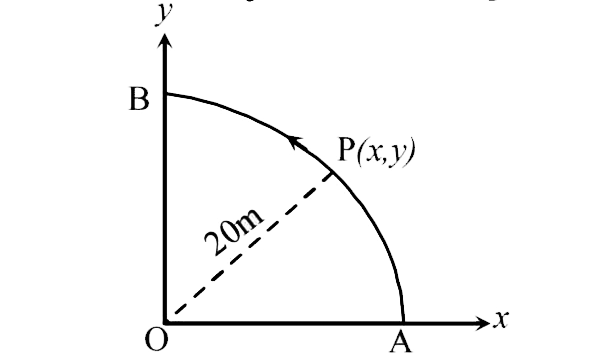
View SolutionA number of particles are projected from a given point with equal velocities in different directions in the same vertical plane. At any instant they will lie on
View SolutionRain water is falling vertically downward with velocity `v`. When velocity of wind is `u` in horizontal direction, water is collected at the rate of `R m^(3)//s`. When velocity of wind becomes `2u` in horizontal direction. The rate of collection of water in vessel is
View SolutionA ball projected from ground at an angle of `45^(@)` just clears a wall infront. If point of projection is `4m` from the foot of wall and ball strikes the ground at a distance of `6m` on the other side of the wall, the height of the wall is
View SolutionThe maximum range of a bullet fired from a toy pistol mounted on a car at rest is `R_(0)=40m`. What will be the acute angle of inclination of the pistol for maximum range when the car is moving in the direction of firing with uniform velocity `V=20m//s`, on a horizontal surface? `(g=10m//s^(2))`
View SolutionFigure shows four paths for a kicked football. Ignoring the effects of air on the flight, rank the paths according to the initial horizontal velocity component, highest first.
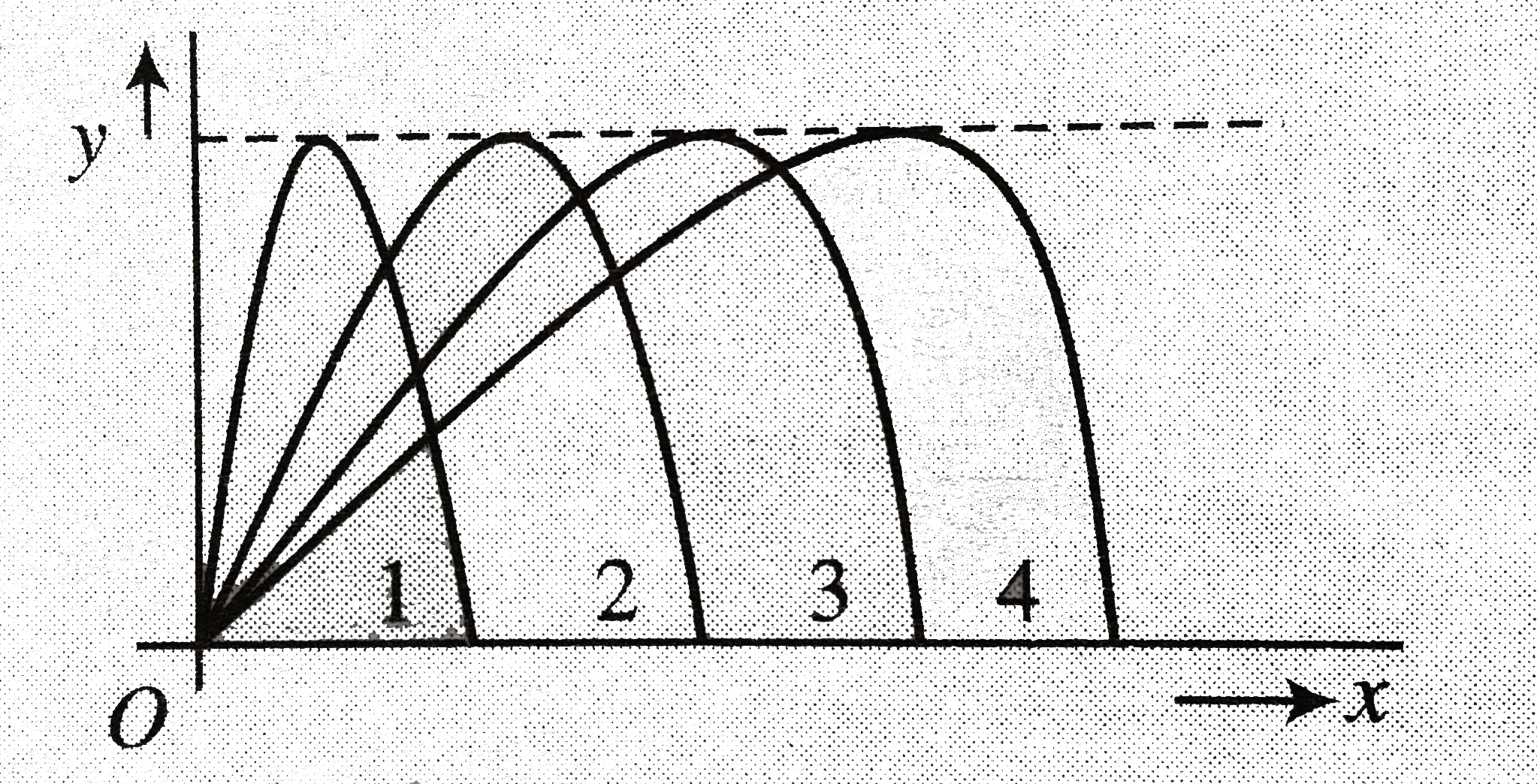 .
.
View SolutionA body is projected at an angle `theta` with the horzontal. When it is at the highest point, the ratio of the potential and kinetic energies of the body is s
View SolutionA particle is projected at an angle `60^(@)` with the horizontal with a speed `10m//s`. Then, latus rectum is (Take `g=10m//s^(2)`)
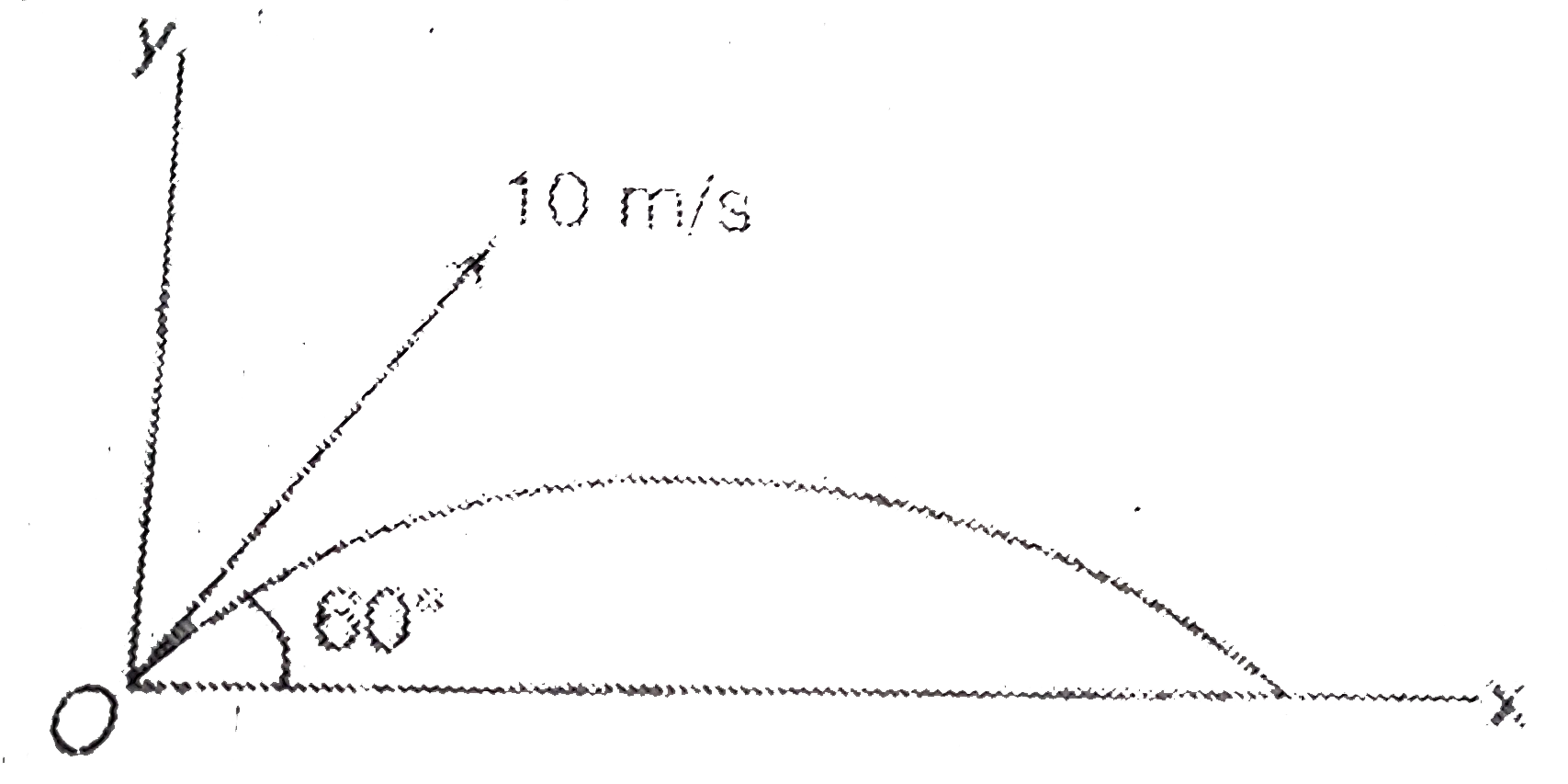
View SolutionA projectile is thrown at an angle `theta` that it is just able to cross a vertical wall at its highest point of journey as shown in the figure. The angle `theta` at which the projectile is thrown is given by

View SolutionTwo cars move in the same direction along parallel roads. One of them is a `200m`, long travelling with a velocity of `20m//s`. The second one is `800m` long travelling with a velocity of `7.5m//s`. How long will it take for the first car to overtake the second car?
View SolutionA motorboat covers the distance between two spots on the river banks `t_(1)=8g` and `t_(2)=12h` in down stream and upstream respectively. The time required for the boat to cover this distance in still water will be
View SolutionTo a person, going eastward in a car with a velociyt of `25km//hr`, a train appears to move towards north with a velocity of `25sqrt(3) km//hr`. The actual velocity of the train will be
View SolutionA man can swim with a speed of `4kmh^(-1)` in still water. He crosses a river 1km wise that flows steadly at `3kmh^(-1)`. If he makes his strokes normal to the river current, how far down the river does he go when he reaches the other bank?
View SolutionRain drops fall vertically at a speed of `20m//s`. At what angle do they fall on the wind screen of a car moving with a velocity of `15m//`, if the wind screen velocity incined at an agle of `23^(@)` to the vertical `("cot"^(-1)4/3~~37^(@))`
View SolutionA bus moves over a straight level road with a constant acceleration a. A boy in the bus drops a ball out side. The acceleration of the ball w.r.t the bus and the earth are respectively
View SolutionA train accelerating uniormly from rest attains a maximum speed of `40ms^(-1)` in `20s`. It travels at this speed for `20s` and is brought to rest with uniform retardation i further `40s`. What is the average velocity during this period?
View SolutionA ball is projected upwards from the top of a tower with a velocity `50ms^-1` making an angle `30^@` with the horizontal. The height of tower is 70m. After how many seconds from the instant of throwing, will the ball reach the ground. `(g=10 ms^-2)`
View SolutionA ball thrown upward from the top of a tower with speed `v` reaches the ground in `t_(1)` sec. If this ball is thrown downward from the top of the same tower with speed `v` it reaches the ground in `t_(2)` sec. In what time, the ball shall reach the grouned, if it is allowed to fall freely under gravity from the top of the tower?
View SolutionA man runs at a speed of `4m//s` to overtake a standing bus. When he is `6m` beind the door at `t=0`, the bus mover forward and continuous with a constant acceleration of `1.2m//s^(2)`. The man reaches the door in time `t`. Then
View SolutionFor a given velocity, a projectile has the same range `R` for two angles of rpojection if `t_(1)` and `t_(2)` are the times of flight in the two cases then
View SolutionA body moves with uniform acceleration, which of the following graph is correct?
View SolutionOne second after the projection, a stone moves at an angle of ` 45^@` with the horizontal. Two seconds from the start,
it is travelling horizontally. Find the angle of projection with the horizontal. `(g=10 ms^(-2))`.
View SolutionA body covers a total distance of `3s`. The first `s` is covered with a velocity `u` the second `s` with `V` and the last `s` with `omega`. Then, the average velocity during the whole journey is
View SolutionDo we observe the time variation of position is nature as shown in the graph?
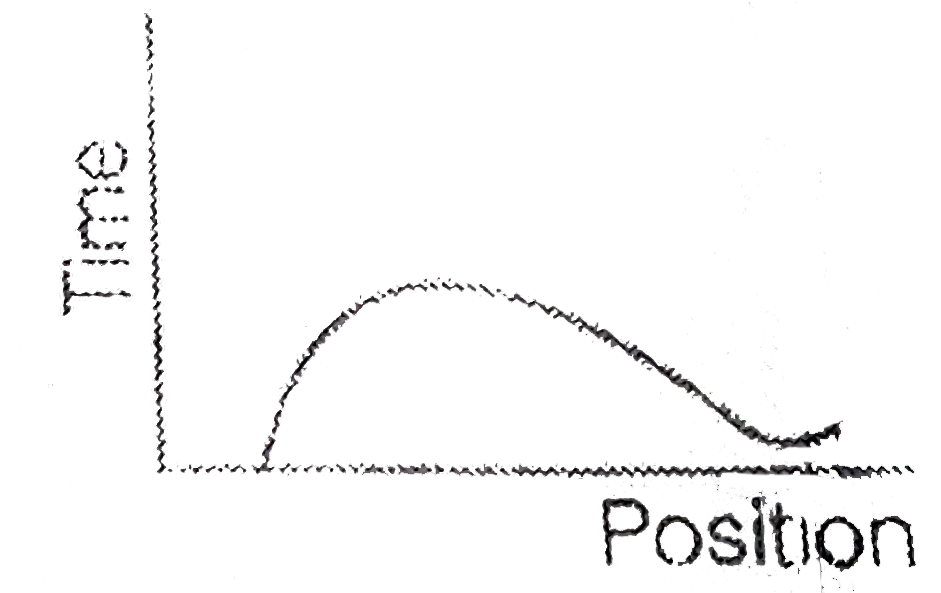
View SolutionIf a body is projected with an angle `theta` to the horizontal, then
View SolutionVelocity-time graph for a moving object is shown in the figure. Total displacement of the object during the time interval when there is non-zero acceleration and retardation is.
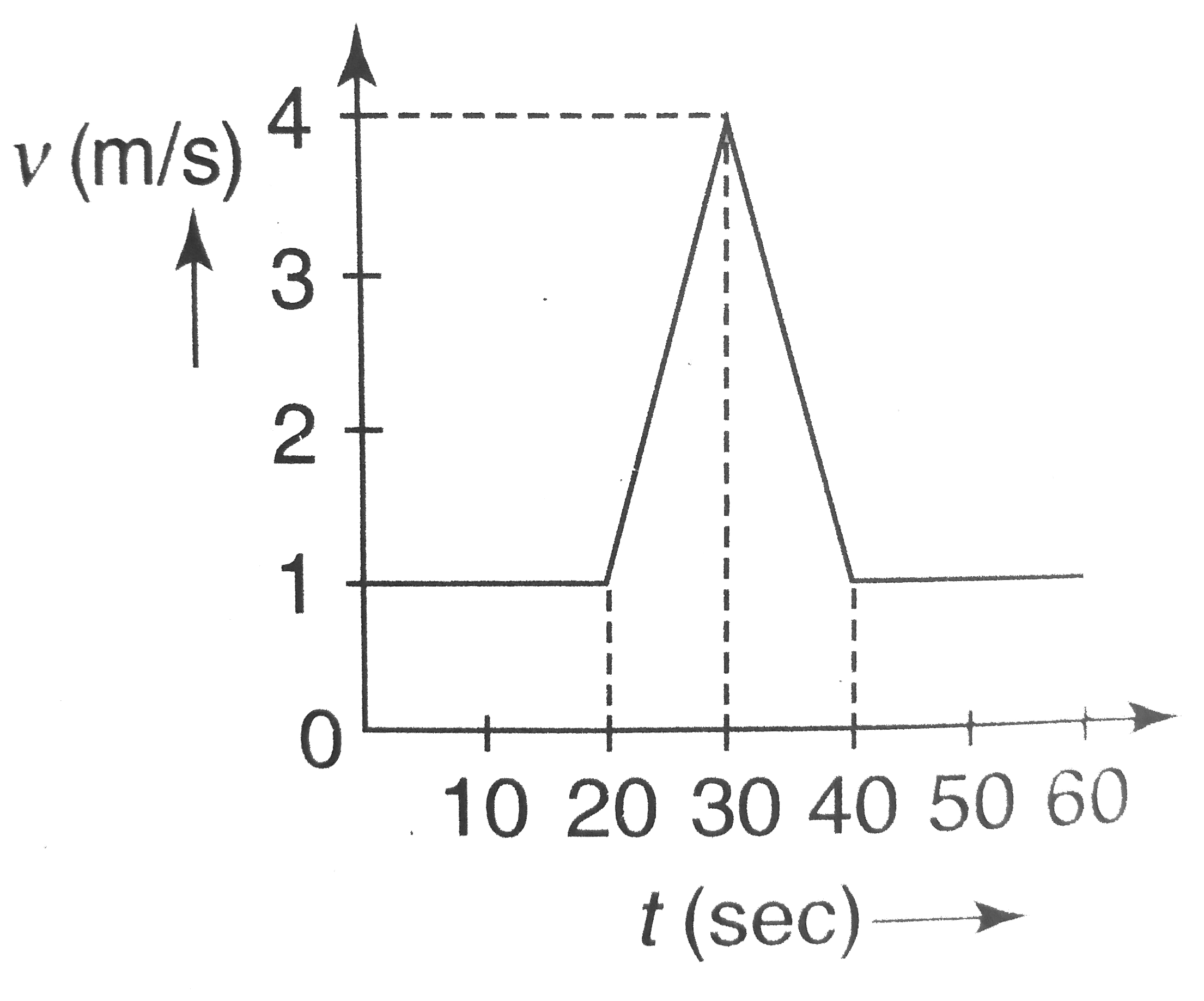
View SolutionAt the top of the trajectory of a projectile, the directions of its velocity and acceleration are
View SolutionAt what point of a projectile motion acceleration and velocity are perpendicular to each other
View SolutionFrom the top of a tower two stones, whose masses are in the ratio 1 : 2 are thrown one straight up with an initial speed u and the second straight down with the same speed u . Then, neglecting air resistance
View Solution
All Questions questions and complete solutions for chapter MOTION IN !,2,2 DIMENSIONS AND POJECTILE MOTION of BITSAT GUIDE PHYSICS (HINGLISH) of Class 11