Complete Solutions to Integer Type of chapter MISCELLANEOUS KINEMATICS of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | MISCELLANEOUS KINEMATICS |
| CENGAGE PHYSICS | Integer Type |
| Number Of Questions | 17 |
| Academic Year | 2023-24 |
In a square cut, the speed of the cricket ball changes from `30 ms^9-1)` to `40 ms^(-1)` during the time of its contact `Delta t = 0 .01 s` with the bat. If the ball is deflected by the bat through an angle of `theta = 90^@`, find the magnitude of the average acceleration `("in" xx 10^(2) ms^(-2))` of the ball during the square cut.
02:02View SolutionA particle moves vertically with an upwards initial speed `v_0 = 10.5 ms^(-1).` If its acceleration varies with time as shown in a-t graph in figure, find the velocity of the particles at t = 4s.
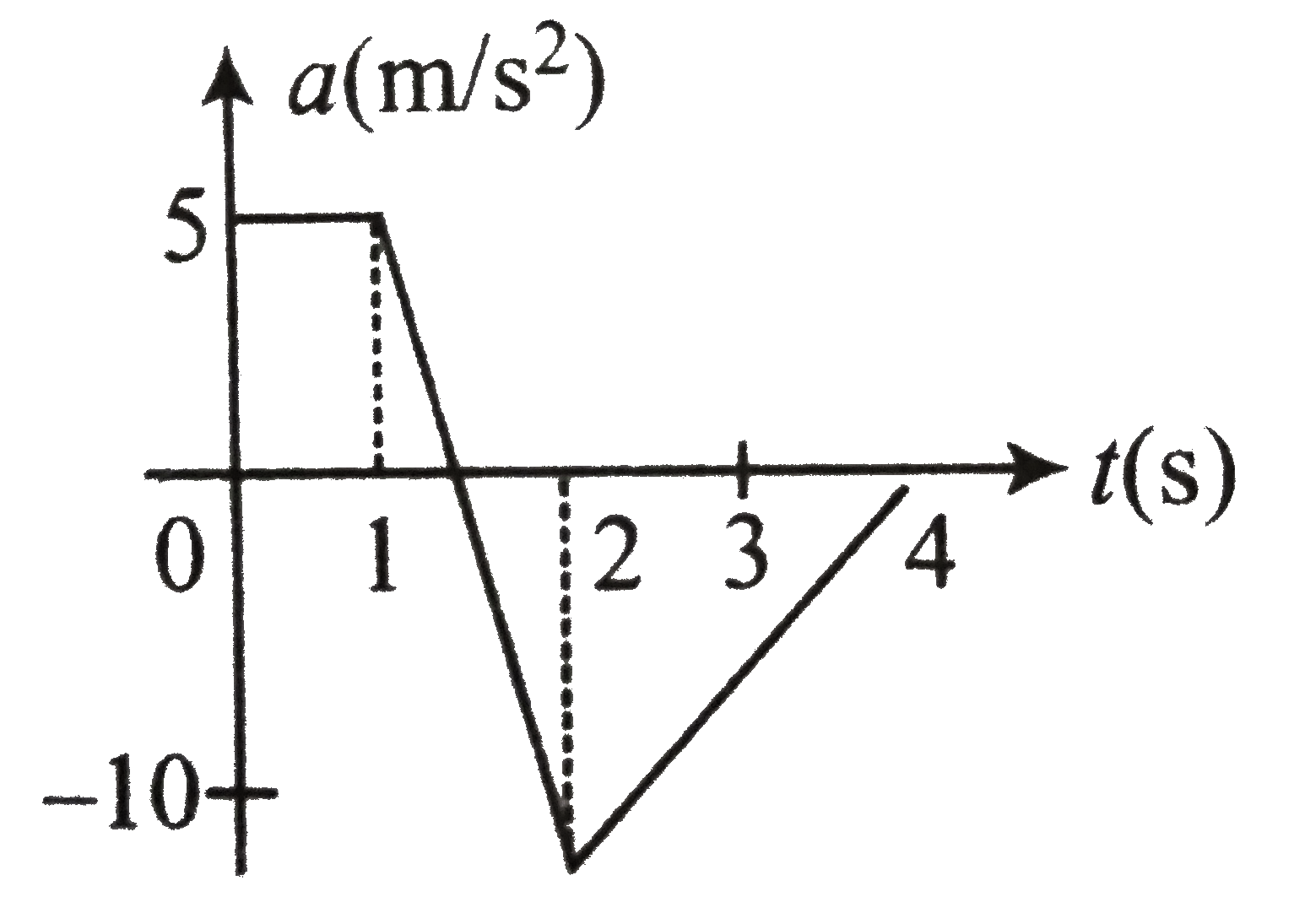 04:22View Solution
04:22View SolutionTwo bodies 1 and 2 are projected simultaneously with velocities `v_1 = 2ms^(-1) and v_2 = 4ms^(-1)` respectively. The body 1 is projected vertically up from the top of a cliff of height h = 10 and the body 2 is projected vertically up from the bottom of the cliff. If the bodies meet, find the time (in s) of meeting of the bodies.
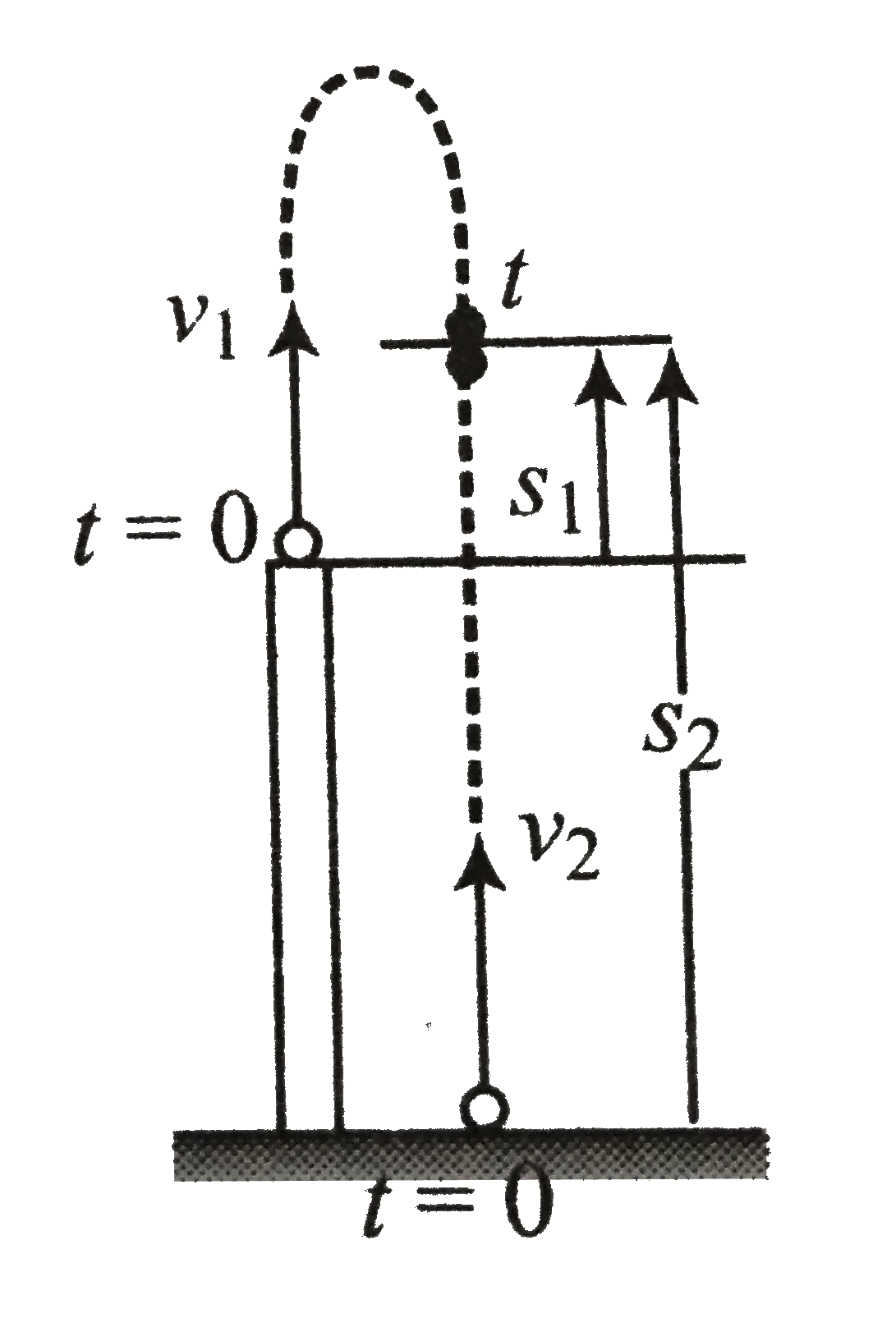 02:08View Solution
02:08View SolutionA particle moves rectilinearly possessing a parabolic s-t graph. Find the average velocity of the particle over a time interval from `t = 1/2 s to t = 1.5 s.`
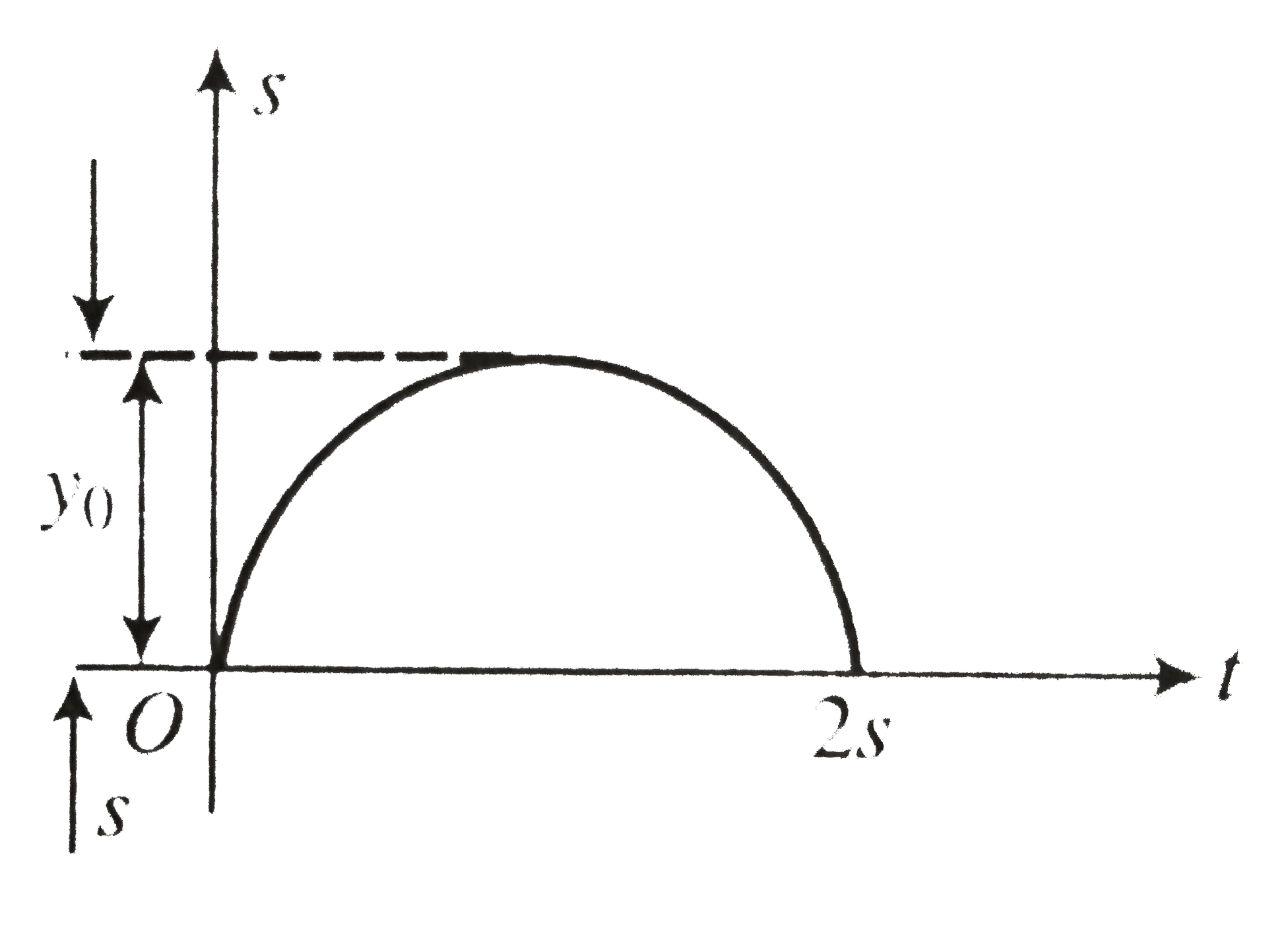 01:25View Solution
01:25View SolutionA ball is projected from the origin. The x- and y-coordinates of its displacement are given by `x = 3t and y = 4t - 5t^2`. Find the velocity of projection `("in" ms^(-1))`.
01:47View SolutionA grasshopper can jump a maximum distance `1.6 m`. It spends negligible time on the ground. How far can it go in `10 s` ?
02:21View SolutionA particle is moving in a circular path of radius 1 m. under the action of a centripetal force, the speed `sqrt2pi ms^(-1)` of the particle is constant. Find the average of the velocity `("in" ms^(-1))` between A and B.
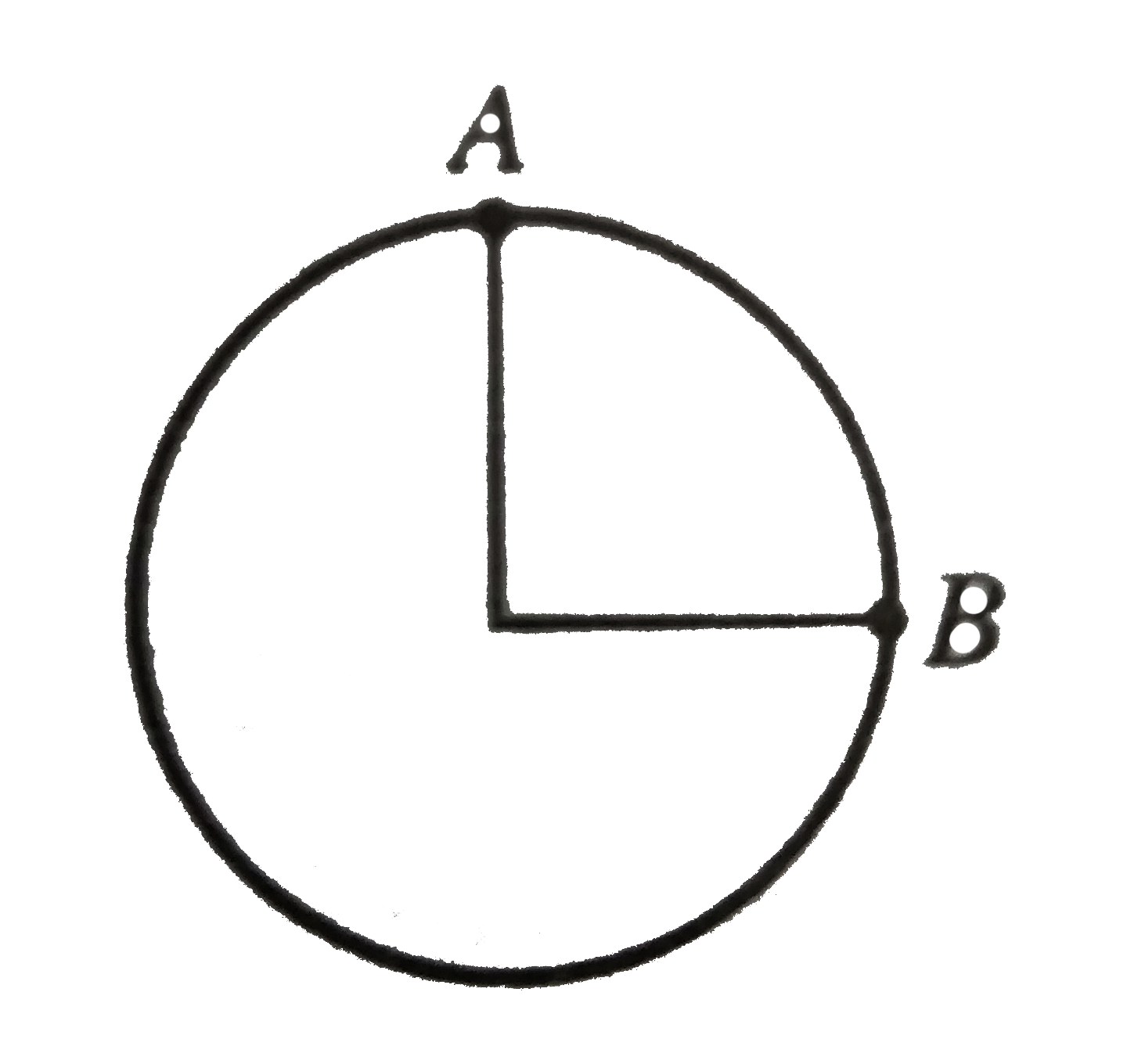 02:26View Solution
02:26View SolutionIn figure, find the horizontal velocity `u (in ms^(-1))` of a projectile so that it hits the inclined plane perpendicularly. Given `H = 6.25m`.
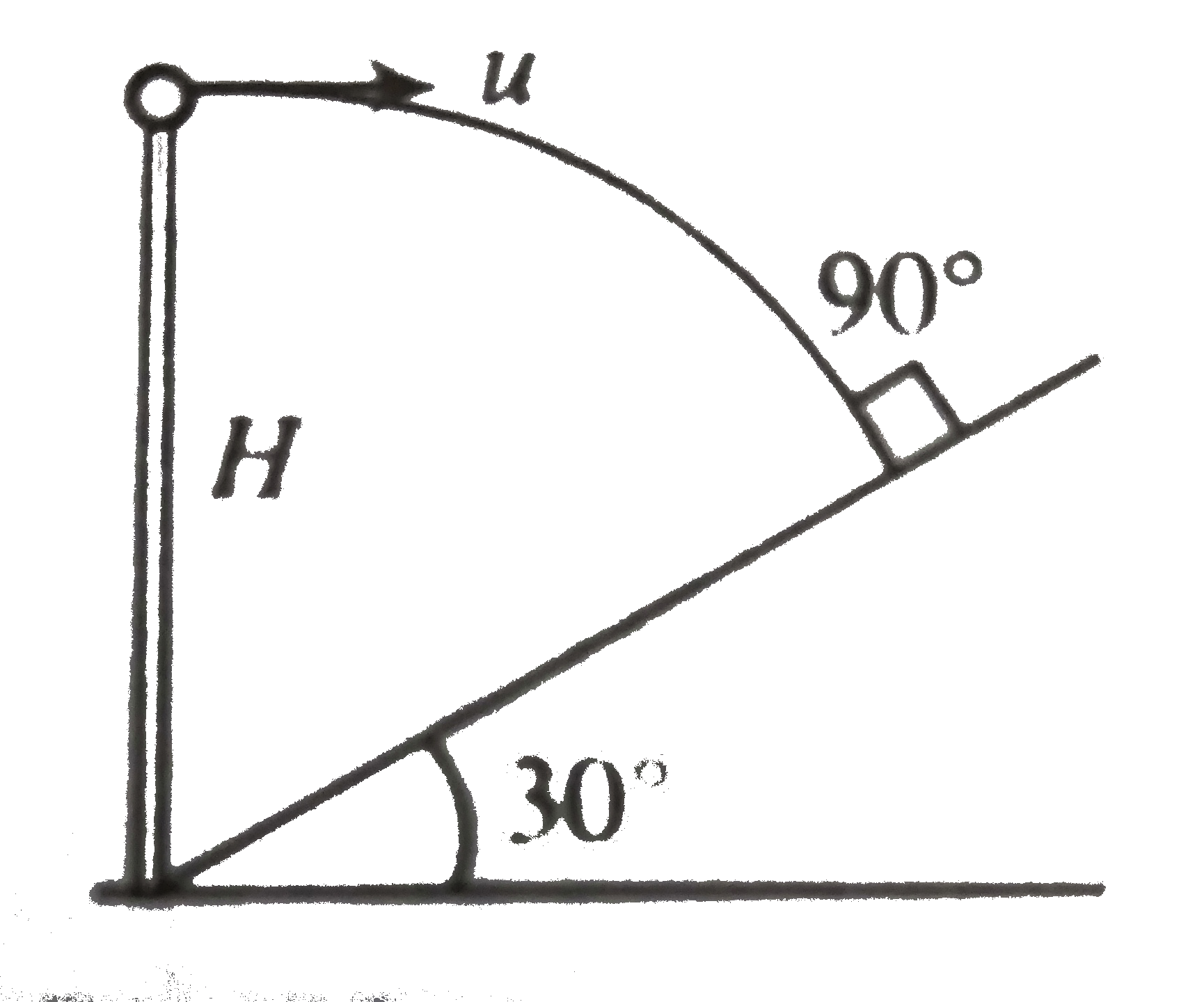 05:19View Solution
05:19View SolutionA particle is projected from a stationary trolley. After projection, the trolley moves with a velocity `2(sqrt15) m//s`. For an observer on the trolley, the direction of the particle is as shown in the figure while for the observer on the ground, the ball rises vertically. The maximum height reached by the ball from the trolley is h meter. The value of h will be .......... .
 02:56View Solution
02:56View SolutionA projectile is launched at time t = 0 from point A which is at height 1 m above the floor with speed `v ms^(-1)` and at and angle `theta = 45^@` with the floor. It passes through a hoop at B which is 1 m above A and B is the highest point of the trajectory. The horizontal distance between A and B is d meters. The projectile then falls into a basket, hitting the floor at C a horizontal distance 3 d meters from A. Find l (in m).
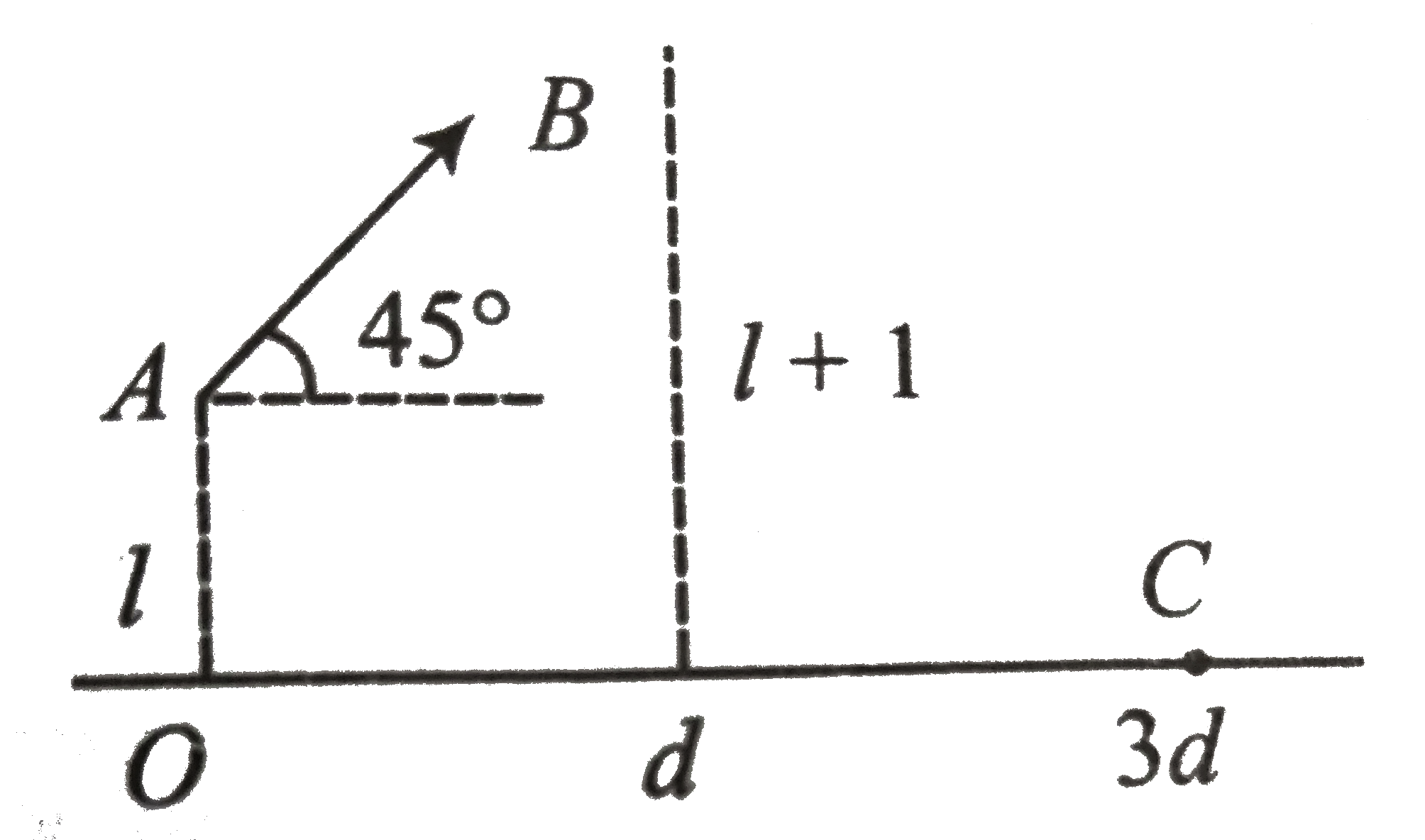 06:20View Solution
06:20View SolutionA particle moves in a straight line. Its position ( in m) as function of time is given by
`x = (at^2 + b)`
What is the average velocity in time interval ` t = 3s to t = 5s in ms^(-1)`. (where a and b are constants and a `= 1ms^(-2), b = 1m`).01:33View SolutionA particle can move only along x-axis. Three pairs of initial and final positions of particle at two successive times are given
Pair Initial positions Final position
1 (-3)m (+5)m
2 (-3) m (-7)m
3 (+7) (-3),
Find the sum of magnitudes of displacement in the pairs
which give negative displacement in m.02:12View SolutionFigure shows a method for measuring the acceleration due to gravity. The ball is projected upward by a gun. The ball pases the electronic gates `1` and `2` as it rises and again as it falls. Each gate is connected to a separate timer. The first passage of the ball through each gate stars the corresponding timer, and the second passage through the same gate stops the timer. the time intervals `/_\t_(1)` and `/_\t_(2)` are thus measured. The vertical distance between the two gates is `d`. If `d=5m, /_\t_(1)=3s, /_\t_(2)=2s`, then find the measured value of acceleration due to gravity (in `m//s^(2)`)
 03:31View Solution
03:31View SolutionAcceleration of particle moving rectilinearly is `a = 4-2x`(where x is position in metre and a in ms^(-2))`. It si at instantaneous rest at x = 0. At what position `x 9`in meter) will the particle again come to instantaneous rest?
01:49View SolutionA big Diwali rocket is projected vertically upward so as to attain a maximum height of 160 m. The rocket explodes just as it reaches the top of its trajectory sending out luminous particles in all possible directions all with same speed v. The display, consisting of the luminous particles, spreads out as an expanding, brilliant sphere. The bottom of this sphere just touches the ground when its radius is 80 m. With what speed `("in" m//s)`are the luminous particles ejected by the explosion?
View SolutionA student throws soft balls out of the window at different angles to the horizontal. All soft balls have the same initial speed `v = 10sqrt3 ms^(-1)`. It turns out that all soft balls landing velocities make angles `30^@` or greater with the horizontal. Find the height h (in m) of the window above the ground.
01:49View SolutionA rope is strecthed between two boats at rest. A sailor in the first boat pulls the rope with a constant force of 100N. First boat with the sailor has mass of 250kg. Whereas the mass of second boat is double of this mass. If the initial distance between the boats was 100m. The time taken for two boats to meet each other is (neglect water resistance between boats and water)
 04:54View Solution
04:54View Solution
Integer Type questions and complete solutions for chapter MISCELLANEOUS KINEMATICS of CENGAGE PHYSICS (HINGLISH) of Class 11
