Complete Solutions to Check point 12.3 of chapter ELASTICITY of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | ELASTICITY |
| DC PANDEY NEET | Check point 12.3 |
| Number Of Questions | 15 |
| Academic Year | 2023-24 |
If the volume of a wire remains constant when subjected to tensile stress, the value of Poisson's ratio of the material of the wire is
View SolutionWhich statement is true for a metal
View SolutionFor a given material, the Young's modulus is 2.4 times that of rigidity modulus . Its poission's ratio is
View SolutionStress-strain curve for four metals are shown in figure. The maximum Young's modulus of elasticity is for metal
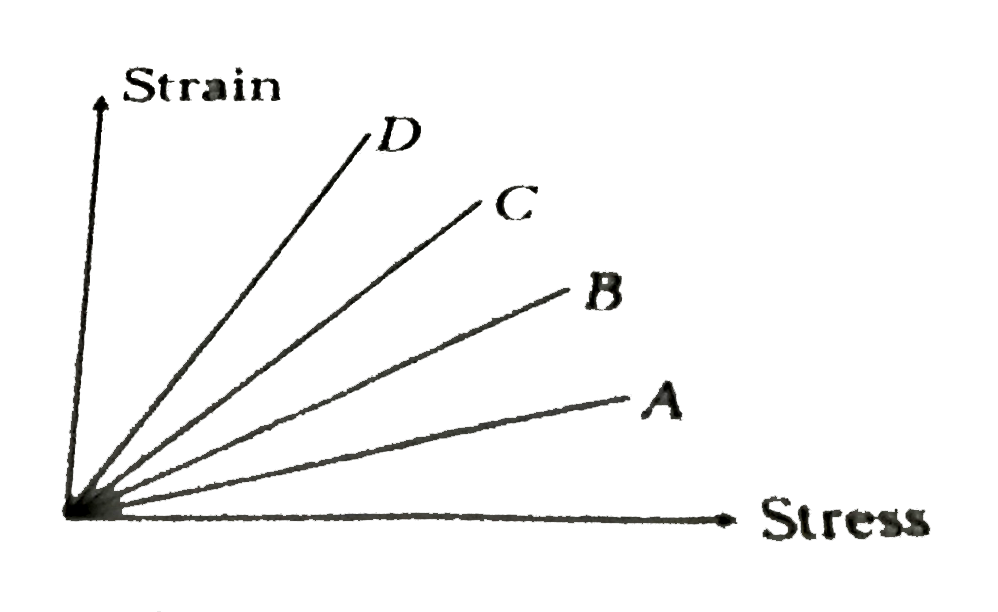
View SolutionSubstances which can be elastically stretched to large value of strain are called
View SolutionA stretched rubber has
View SolutionA metallic rod of length l and cross-sectional area A is made of a material of Young's modulus Y. If the rod is elongated by an amount y,then the work done is proportional to
View SolutionThe work per unit volume to stretch the length by `1%` of a wire with cross sectional area of `1mm^2` will be. `[Y=9xx10^(11)(N)/(m^2)`]
View SolutionWhen a load of 10 kg is hung from the wire, then extension of 2m is produced. Then work done by restoring force is
View SolutionA wire suspended vertically from one of itsends is strached by attached a weight of `200 N` to the lower end . The weight streches the wire by `1 mm` . Then the elastic energy stored in the wire is
View SolutionIf a spring extends by `x` on loading, then the energy stored by the spring is (if T is tension in the spring and k is spring constant)
View SolutionTwo wires A and B are made of same material. The wire A has a length l and diameter r while the wire B has a length 2l and diameter r/2. If the two wires are stretched by the same force the elongation in A divided by the elongation in B is
View SolutionA wire of length `50 cm` and cross sectional area of 1 sq. mm is extended by `1mm`. The required work will be `(Y=2xx10^(10) Nm^(-2))`
View SolutionWhen a force is applied on a wire of uniform cross-sectional area `3xx10^-6m^2` and length 4 m, the increase in length is 1mm. Energy stored in it will be `(Y=2xx10^(11)(N)/(m^2)`)
View SolutionWhen a 4 kg mass is hung vertically on a light string that obeys Hooke's law, the spring stretches by 2 cm. The work required to be done by an external agent in stretching this spring by 5 cm will be (`g=9.8(metrs)/(sec^2)`)
View Solution
Check point 12.3 questions and complete solutions for chapter ELASTICITY of DC PANDEY ENGLISH of Class 11
All Exercises from DC PANDEY NEET Class 11 PHYSICS ELASTICITY
- Assertion And Reason
- Chapter Exercise
- Check point 12.1
- Check point 12.2
- Check point 12.3
- Example
- Exercise 15.1
- Exercise 15.2
- Level 1 Single Correct
- Level 1 Subjective
- Level 2 Comprehension Based
- Level 2 More Than One Correct
- Level 2 Single Correct
- Level 2 Subjective
- Match the columns
- Medical entrances s gallery
- MiscellaneousExamples
- Solved Examples