Complete Solutions to SOLVED EXAMPLES of chapter DYNAMICS of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | DYNAMICS |
| S CHAND | SOLVED EXAMPLES |
| Number Of Questions | 99 |
| Academic Year | 2023-24 |
A particle is moving along, the x axis. At time `t_(1)= 1s` the particle is located at `x_(1)= 5m " and at "t_(2)= 11s` the particle is at `x_(2)= 20m`. Calculate its displacement, average velocity and average speed during this time interval.
View SolutionA particle is moving along the x-axis. Its position at any instant is given by the equation `x= bt^(2)` where b is a constant and `b=1`. What is the instantaneous velocity at `t= 4s`? Find the average velocity from `4s" to "4.01s`.
View SolutionThe variation of the position coordinate of a particle with time, moving along the x-axis is given by, `x=8-7t+10t^(2)`. Calculate the initial velocity and acceleration of the particle.
View SolutionA car starts from rest travelling along a straight line with uniform acceleration, attains a velocity of `40.0 m"/"s " in "8.0s`. What is its acceleration ?
View SolutionBy seeing a child on the railway track the driver applies brake. The speed of the train is reduced from 90 kmph to 36 kmph in 5s. What is the distance travelled by the train during this time interval?
View SolutionIn the problem 4.5, how much time would elapse in bringing the train to rest from 90 kmph, assuming its deceleration to be uniform?
View SolutionIn the problem 4.6, what distance would be covered by the train when it is brought to rest from 90 kmph in `25"/"s` ?
View SolutionThe speed of a car is reduced from 60 kmph to 30 kmph over a displacement of 20 m. What is the acceleration of the car assuming to be constant?
View SolutionA particle starts from rest and travels with a uniform acceleration of `2 m"/"s^(2)`. What is the distance travelled by the particle in the 10th second of its motion?
View SolutionA car passes three points A, B and C at `5 m"/"s, 10 m"/"s " and "15 m"/"s` respectively, with uniform acceleration. If the distance AB= 200m, calculate the distance BC?
View SolutionThe two ends of a train moving with uniform acceleration passes a certain point with velocities 36 kmph and 72 kmph. What is the velocity with which the middle point of the train passes the same point?
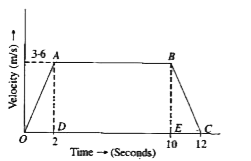
View SolutionThe variation of the velocity of a particle moving along a straight line is illustrated in Fig. 4.1.11. What is the distance traversed by the particle in 4s?
View SolutionA lift is going up. The variation in the speed of the lift is given in the graph (see Fig. 4.1.12). What is the height to which the lift takes the passengers?
View SolutionA stone is thrown vertically downwards with a velocity of `2 m"/"s` from the top of a tower of the height of 40m. Find
The velocity of the stone after 1s.

View SolutionA stone is thrown vertically downwards with a velocity of `2 m"/"s` from the top of a tower of the height of 40m. Find
How high above the ground will the stone be after 2s?

View SolutionA stone is thrown vertically downwards with a velocity of `2 m"/"s` from the top of a tower of the height of 40m. Find
With what velocity will the stone hit the ground?
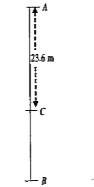
View SolutionA stone is thrown vertically downward with a velocity u from the top of a tower of height h and another stone is thrown vertically upward with the same velocity u. What is the ratio of the speeds of the two stones when they hit the ground near the base of the tower?
View SolutionFrom the top of a tower one stone is dropped and another stone is thrown vertically downwards with velocity of `3 m"/"s`. After how much time will their separation be 10m?
View SolutionA stone is thrown vertically upwards such that when it reaches half to its maximum height it has a velocity of `6.9 m"/"s`. What is the maximum height attained by the stone?
View SolutionA ball falls freely on a perfectly elastic plate from a height of `3.0m`. At the instant `t= 0`, the velocity of the ball is `v= 0`. Draw the velocity time graph for the motion of the ball.
View SolutionA bus and a car start from rest and run along a straight road with uniform acceleration of `2 m"/"s^(2)" and "3 m"/"s^(2)` respectively, with the car behind the truck at a distance of 100m. How long will it take before both the bus and car travel side by side?
View SolutionTwo trains A and B, 125m and 100m long are moving in opposite directions on parallel tracks. The velocity of the train B is three times that of the train A. The trains take 4sf to pass each other, calculate the velocity of each train?
View SolutionTrain A is moving with a speed of `30 m"/"s`. At time `t=0`, the driver of this train sees another train on the same track at a certain distance ahead of him moving at a speed of `15 m"/"s`, along the same direction. The driver of the train A immediately applies brake and the train moves with a constant retardation of `4 m"/"s^(2)`. What is the distance between the trains so that they do not collide?
View SolutionA boy runs towards a stationary bus with a speed of `5 m"/"s`. When he is 5m behind the bus, the bus suddenly starts moving with a constant acceleration of `1 m"/"s^(2)`. How lond does the boy take to get in the bus?
View SolutionA ball is suspended by a string of length `0.75m` and is made to oscillate in a vetical circle. The ball has a speed of `1 m"/"s` when `theta= 10^(@)`. What is the magnitude of the radical component of the acceleration at that instant? What is magnitude of the tangential acceleration? Find the magnitude and direction of the total acceleration when `theta= 10^(@)`.
View SolutionA shell is fired horizontally with a velocity of `150 m"/"s` from the top of a tower. It hits a target on the ground after 3s.
What is the height of the tower?
View SolutionA shell is fired horizontally with a velocity of `150 m"/"s` from the top of a tower. It hits a target on the ground after 3s.
Where is the target situated?
View SolutionA shell is fired horizontally with a velocity of `150 m"/"s` from the top of a tower. It hits a target on the ground after 3s.
With what vertical velocity does the shell strike the ground?
View SolutionWith what velocity must a stone by thrown horizontally from a window `172.5m` above the ground in a building A so that it enters a window 50m above the ground in another building B? The two buildings separated by a horizontally distance of 50m.
View SolutionA ball is projected horizontally from the top of a tower 25m high. After how long does it strike the ground. Calculate the initial velocity of the ball if the line joining the point of projection to the point where it hits the ground makes an angle of `45^(@)` with the horizontal?
View SolutionStarting from rest a ball falls freely through a height h and is then made to strike an inclined plane of angle `theta` as shown in Fig. 4.2.11. The ball rebounds elastically off the plane and strikes the plane at another point below the first impact point. What is the distance between the two impact points ?
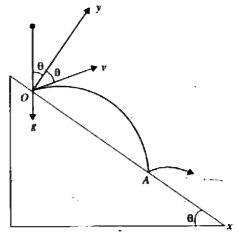
View SolutionA body falling freely from a given height H hits an inclined plane in its path at a height h. As a result of this impact the direction of the velocity of the body becomes horizontal. For what value of (h/H), the body will take the maximum time to reach the ground.
View SolutionTwo stones are projected horizontally from the top of a tower with velocities v and 2v respectively. Calculate the ratioof the times taken by them to reach the ground. Also find the ratio of the horizontal distances travelled by them?
View SolutionA projectile is fired with a muzzle velocity of `300 m"/"s` at an angle of elevation `30^(@)` above the horizontal, Calculate the height at which it will strike a vertical cliff at a horizontal distance of 4500 m from the point of projection. What will be its velocity when it strikes the cliff ?
View SolutionWhat is the angle of projection for which the maximum height is equal to half of the horizontal range ?
View SolutionWhat should be the speeds of two persons such that when they move uniformaly towards each other, they get `3.0 m` closer each second and when they move uniformly in the same direction with the original speeds, they get `3.0 m` closer each 10s ?
View SolutionA person can row a boat `4 m"/"s` in still water.
Suppose he is crossing a lake where the current is `2 m"/"s`, in what direction will his boat be headed if he wants to reach a point directly opposite to his starting point ?
View SolutionA person can row a boat `4 m"/"s` in still water.
If the lake is 200 m wide how long will it take him to cross the river ?
View SolutionIn problem 4.2.11, how long will the person take to row 100 m down the lake and then back to his starting point ?
View SolutionA constant force `vecF` acting on a body of mass `m_(1)` produces an acceleration of `4.00 m"/"s^(2)`. The same force applied to another body of mass produces an acceleration of `2.00 m "/"s^(2)`.
What is the value of the ratio `(m_1)/(m_2)`?
View SolutionA constant force `vecF` acting on a body of mass `m_(1)` produces an acceleration of `4.00 m"/"s^(2)`. The same force applied to another body of mass produces an acceleration of `2.00m "/"s^(2)`.
If `m_(1)` and `m_(2)` are combined what is the common acceleration under the action of the same force ?
View SolutionA particle of mass 10.0 kg starts from rest and moves through a dostance of 5 m in 2s under the action of a costant force. What is the magnitude of the force ?
View SolutionA particle of mass 4.0 kg moves with a constant acceleration of `(3.0 hati+ 5.0 hatj) m"/"s^(2)`.
Calculate the resultant force `vecF` and its magnitude.
View SolutionA body fo mass 20 kg is moving with a velocity `50 ms^(-1)`. Find the force required to stop it in
5 s .
View SolutionA body fo mass 20 kg is moving with a velocity `50 ms^(-1)`. Find the force required to stop it in
In a distance of 250 m ?
View SolutionA block of mass m is placed on a horizontal frictionless table. Under the action of a force of constant magnitude `F = (mg)/(4)` the block starts moving. During the translatory motion of the block the angle `theta` between the direction of the force varies as `theta=bs` where b is a constant, and s is the distance travelled by the block from its initial position. Derive an expression for the velocity of the block in terms of the angel `theta`.
View SolutionOn a planet A a body weighs 25 N. On another planet B, The magnitude of acceleration due to free fall is `1.5 g (g = 9.8 m "/" s)` and the body weighs 50 N. What is the mass of the body and its free-fall acceleration in planet A ?
View SolutionA ball of mass `0.50 kg` is thrown with a speed of `10 m"/" s`. A stationary receiver catches the vall and brings it to rest in `0.02s`.
What is the impulse dellvered to the ball ?
View SolutionA ball of mass `0.50 kg` is thrown with a speed of `10 m"/" s`. A stationary receiver catches the vall and brings it to rest in `0.02 s`.
What is the average force exerted on the receiver ?
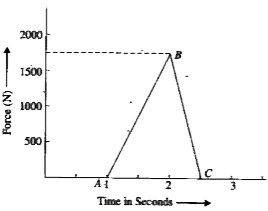
View SolutionThe force- time graph for a ball struck with a bat is as shown in Fig. 4.3.5. From the graph calculate.
The impulse delivered to the ball.
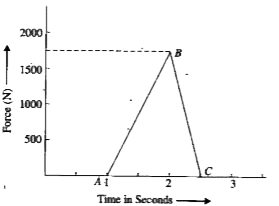
View SolutionThe force- time graph for a ball struck with a bat is as shown in Fig. 4.3.5. From the graph calculate.
The average force exerted on the ball.
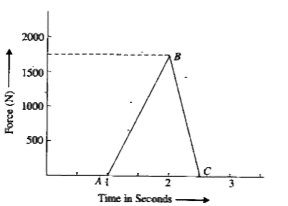
View SolutionThe force- time graph for a ball struck with a bat is as shown in Fig. 4.3.5. From the graph calculate.
The peak force exerted on the ball.
View SolutionTwo masses 340 gm and 300 gm are connected at the two ends of a light inextensible string that passes over a frictionless pulley. What is the distance travelled by the masses in 6 seconds, starting from rest ?
View SolutionTwo masses are connected at the two ends of a light inextensible string that passes over a frictionless pulley. The difference in the masses is `0.5 kg`. The system moves through 10 m in 2s, starting from rest. What are the masses ?
View SolutionA trolley of mass `1.5 kg` is pulled along a table by string attached to a mass of `0.02 kg` hanging down over a pulley. (See Fig. 4.3.13). If the trolley starts from rest calculate the distance travelled by the body in 5s.
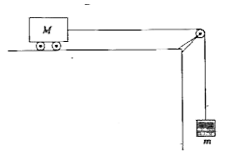
View SolutionThe total mass of an elevator and its content is 500 kg. The elevator is moving downwards with a velocity of `4.9 m "/"s`. The elevator is brought to rest in a distance fo 30 m. Assuming the acceleration of the elevator to be uniform, calculate the tension T is the cable supporting the elevator.
View SolutionTwo equal masses 300 gm each are connected at the two ends of light inextensible staring that passes over a frictionless pulley. AS small mass (rider) is kept on one of them for 3 s and the nremoved. If the velocity of the system is `9 m "/" s`. What is the mass of the rider ?
View SolutionA gun weighing 10kg fires a bullet of 50g with a velocity `300 m"/"s`. With what velocity does the gun recoil? Calculate the resultant momentum of the gun and the bullet before firing and after firing.
View SolutionA body of mass 10 kg travelling with a velocity of `2 m "/"s` collides with a body of mass 20 kg at rest. If the velocity of the first body is `1 m "/"s` after collision in the same direction, calculate the velocity of the second body.
View SolutionA shell moving with a velocity of `1000 m "/"s`, suddenly bursts into two fragments which continue to move in the same direction with velocities `1200 m"/"s" and "400 m "/"s`. What is the ratio of the masses of the two fragments ?
View SolutionA 3000 kg meteorite has a speed of `300 m"/"s` just before colliding head on earth. What is the recoil speed of the earth ? Mass of the earth `= 6 xx 10^(24) kg`.
View SolutionA man of mass `49.5 kg` stands on a frozen pond near to a wall. He throws a ball of mass `0.50 kg` with a speed of `10 m"/"s` relative to the ground, at the wall and then catches the ball after it rebounds from the wall.
How fast is the moving after he catches, the ball ?
View SolutionA man of mass `49.5 kg` stands on a frozen pond near to a wall. He throws a ball of mass `0.50 kg` with a speed of `10 m"/"s` relative to the ground, at the wall and then catches the ball after it rebounds from the wall.
How many times does the man have to go through this process before his speed reaches `1.0 m"/"s` relative to the ground ?
View SolutionA rocket, set for vertical firing weighs 50kg and contains 450kg of fuel. It can have a maximum exhaust velocity of `2 km"/"s`
What should be its minimum rate of fuel consumption.
To just lift off the launching pad.
View SolutionA rocket, set for vertical firing weighs 50 kg and contains 450 kg of fuel. It can have a maximum exhaust velocity of `2 km"/"s`
What should be its minimum rate of fuel consumption
To give it an acceleration of `20 m"/"s^(2)`.
View SolutionA rocket, set for vertical firing weighs 50 kg and contains 450 kg of fuel. It can have a maximum exhaust velocity of `2 km"/"s`
What will be the speed of rocket when the rate of consumption of fuel is `10 kg"/"s`.
View SolutionA block of mass 2kg is pushed 2m along a frictionless horizontal table by a constant force of 15N. What is the work done by
The applied force.
View SolutionA block of mass 2kg is pushed 2m along a frictionless horizontal table by a constant force of 15N. What is the work done by
The normal force exerted by the table.
View SolutionA block of mass 2kg is pushed 2m along a frictionless horizontal table by a constant force of 15N. What is the work done by
The force of gravity.
View SolutionA lady pulls a vaccum cleaner with a force of magnitude 75N. The force makes an angle of `30^(@)` with the horzontal. If the vaccum cleaner is displaced `5.0m` in the direction of the force calculate the work done.
View SolutionA constant force `vecF= (6.0hatj+4hatj)N` acts on a particle and it undergoes a displacement `S= 3.0hati+4hatj`. Calculate the work done by the force.
View SolutionThe relationship between force and position of a body moving along x-axis, is as shown in Fig. What is the work done by the force in displacing the body from x= 1 m to x= 5m?
View SolutionThe force acting on a particle moving parallel to the x-axis is `F= 2x+3x^(2)`. Calculate the work done in displacing the particle from x= 1 to x= 2.
View SolutionA body moves along the circumference of a circle of radius 2m. What is the work done by a force of magnitude 15 newton acting on the body inclined at an angle `60^(@)` to the tangent at any point on the circle?
View SolutionA car of mass 2000kg is pushed from rest to a speed v by doing a work of 4000J. The car moves through a distance of `20.0m`. Neglecting friction between the car and the road
What is the final speed v of the car?
View SolutionA car of mass 2000kg is pushed from rest to a speed v by doing a work of 4000J. The car moves through a distance of `20.0m`. Neglecting friction between the car and the road
What is the horizontal force exerted on the car?
View SolutionA `50.0g` bullet is accelerated to a rifle barrel `72.0cm` long to a speed of `720 m"/"s`. Calculate the average force exerted on the bullet while it is accelerated, using work-energy theorem.
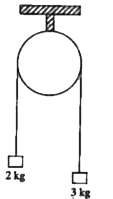
View SolutionTwo masses 2kg and 3kg are connected at the two ends of a light inextensible string that goes over a frictionless pulley. The masses are held at rest beside each other and then released. Find the speed of each mass, at the instant they have both moved `0.05m`?
View SolutionThe variation of a conservative force acting on a particle is `F= -Ax+Bx^(2)N` where A and B are constants and x is in m. Find the potential energy associated with the force?
View SolutionIf `U_(x, y)= (1)/(2)k (x^(2)+y^(2))` find the x and y component of the force and the total force on the particle.
View SolutionThe potential energy function for the between two atoms in a diatomic molecule can be approximately be expressed as,
`U_(x)= (a)/(x^(12))-(b)/(x^6)` where 'a' and 'b'
are positive constants and x is the distance between the atoms. Find the force between the two atoms?
View SolutionA block of mass m is placed on the top of a vertical spring of force constant k and pushed downward, compressing the spring a distance x. After the block is released it travels upward and then leaves the spring. Calculate the maximum height h to which it rises above the point of release?
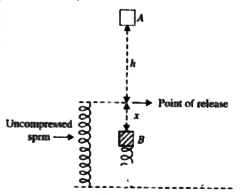
View SolutionA ball rolls down from the top of a curved slide of height 10m. The ball starts from rest at the top. Assuming that there is no friction, what is the speed of the ball at the bottom?
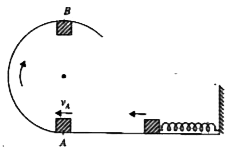
View SolutionIn Fig. a block of mass `m= 1.0kg` is pushed against a horizontal massless spring, compressing the spring by an amount x. When the mass is released, the block travels along a frictionless, horizontal surface to the point A. The point A is at the bottom of a vertical circular track of radius `1.5m`. The block continues to move up the track. The block experiences an average frictional force of `5.0 N`
The speed of the block at the bottom of the track is `v_(A)= 11 m"/"s`. Calculate the value of x, if the spring constant of the spring is `500 N"/"m`.
View SolutionIn Fig. a block of mass `m= 1.0kg` is pushed against a horizontal massless spring, compressing the spring by an amount x. When the mass is released, the block travels along a frictionless, horizontal surface to the point A. The point A is at the bottom of a vertical circular track of radius `1.5m`. The block continues to move up the track. The block experiences an average frictional force of `5.0 N`
What is the speed of the block at the top of the track, `(v_B)`?
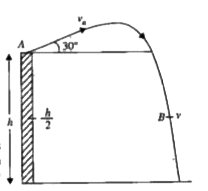
View SolutionA shot is fired from the top of a tower at an angle `30^(@)` to the horizontal with a velocity `v_0`. If the height of the tower is h, calculate the speed of the shot at an altitude `h"/"2`, by energy methods.
View SolutionA solid of density half that of water falls freely under gravity from a height of `19.6m` and then enters water. Upto what depth will the ball go? How much time will it take to come again to the water surface?
View SolutionThe total mass of a lift is 1500kg. The lift ascends through a vertical height of 50m in 2 minutes. If 25% of the power developed is wasted, calculate the power of the engine operating the lift?
View SolutionA constant force of 100N acts for 5s on a body of mass 10kg, initially at rest. Find
The work done by the force.
View SolutionA constant force of 100N acts for 5s on a body of mass 10kg, initially at rest. Find
The final K.E.
View SolutionA constant force of 100N acts for 5s on a body of mass 10kg, initially at rest. Find
The average power of the force?
View SolutionAn engine of mass 7000kg is going up an incline of 1 in 10 at the rate of 36 kmph. What is the power of the engine if the coefficient of friction is `0.05`?
View SolutionA sand bag of mass 10kg is suspended with a 3m long weightless string. A bullet of mass 200gm is fired with a speed of `20 m"/"s` into the bag and stays in the bag. Calculate
The speed acquired by the bag.
View SolutionA sand bag of mass 10kg is suspended with a 3m long weightless string. A bullet of mass 200gm is fired with a speed of `20 m"/"s` into the bag and stays in the bag. Calculate
The energy converted to heat in the collision.
View SolutionA bullet of mass `0.01kg` is fired horizontally into a 4kg wooden block at rest on a horizontal surface. The coefficient of kinetic friction between the block and the surface is `0.25`. The bullet remains embedded in the block and the combination moves 20 m before coming to rest. With what speed did the bullet strike the block?
View SolutionFig. shows two blocks of masses `m_(1)= 3kg" and "m_(2)= 5kg` both moving towards right on a frictionless surface with speed `10 m"/"s" and "4 m"/"s` respectively. To the back compression of the spring when the blocks collide?
View SolutionA bullet of mass m moving with horizontal velocity v strikes a stationary block of mass M suspended by a string of length L. Then bullet gets embedded in the block. What is the maximum angle made by the string after impact?
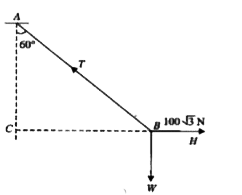
View SolutionA load suspended by a string is pushed to one side by a horizontal force of `100sqrt(3)N` and the string makes an angle of `60^(@)` with the vertical. Using the triangle law of forces calculate
The load and the tension in the string.
View SolutionAt the extremities of a uniform road 1 m long, two parallel forces are exerted. When the forces act in the same direction their resultant is 8 N and when the forces act in the opposite direction their resultant is 2 N. Find the forces and the line of action of their resultant.
View Solution
SOLVED EXAMPLES questions and complete solutions for chapter DYNAMICS of S CHAND PHYSICS (ENGLISH) of Class 11